Suppose a satellite is orbiting the Earth. The gravitational and centripetal force supposedly point towards the Earth. Therefore, the net force is towards the Earth. Since the satellite doesn't fall immediately towards the earth, what's the third force holding the satellite in orbit? First impression tells me its the elusive "centrifugal force", but I was told to use this with caution as it's often a misnomer. What's the correct line of reasoning behind this?
$\begingroup$
$\endgroup$
5
-
1$\begingroup$ net forces can end up being centripetal. Centripetal force isn't a <b>type</b> of force the way gravity or the electric forces are. $\endgroup$– Zo the RelativistCommented Apr 10, 2012 at 21:29
-
$\begingroup$ gravity and centripetal forces are one and the same thing in this case... in a manner of speaking, ` gravity provides the necessary centripetal force required to make the earth move around sun`.... $\endgroup$– Vineet MenonCommented Apr 18, 2012 at 7:08
-
1$\begingroup$ There is no force. The sattelite has a constant velocity tangential to the earth and thats enough. This velocity is the square root of the gravitational potential. $\endgroup$– Abhimanyu Pallavi SudhirCommented Jun 6, 2013 at 13:08
-
5$\begingroup$ essentially a duplicate of physics.stackexchange.com/q/9049/50583 $\endgroup$– ACuriousMind ♦Commented Jan 24, 2016 at 18:28
-
$\begingroup$ Of course not @ACuriousMind ! The question you are comparing with is enough different and somehow related to this one. You may read my answer to understand why this is different. $\endgroup$– user104909Commented Jan 24, 2016 at 18:50
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
2
In this case, the gravitational force is the centripetal force, i.e. the force which keeps the satellite moving in orbit. As you have correctly surmised, the net force is towards the Earth, and the satellite will accelerate in that direction.
What makes you think there is another force "holding the satellite in orbit"?
-
$\begingroup$ True, it's actually the gravitational force that keeps the satellite in orbit. Were it not for it, the satellite would fly away. $\endgroup$ Commented Apr 10, 2012 at 22:42
-
$\begingroup$ What makes the satellite move though? I'm currently on this topic and I really don't get how an acceleration will produce a velocity vector that is perpendicular to it? $\endgroup$ Commented Dec 29, 2014 at 1:01
$\begingroup$
$\endgroup$
2
Elaborating on tmarthal and tmac's answers:
Centripetal force
The force which acts on a body undergoing circular motion, always pointing towards the centre of the circle. This force ensures that the body remains at a fixed distance from the center of the circle. It can be gravitational, electrostatic, magnetic etc. Edit: It can also be a combination of two or more different kinds of forces.
Think of it this way:
The green thingy is a ball whose velocity is initially along the black horizontal line. Now I apply a force along the red line, which is perpendicular to the black one.
This causes the ball to turn ever so slightly downwards, so that it has a new velocity (v').
At the same time, I adjust the direction of my force so that I have a new force (F') which is perpendicular to v'.
So the velocity keeps on changing direction, and I keep on adjusting the direction of my force so that the ball moves in a circle. At any point, the force is perpendicular to the velocity.
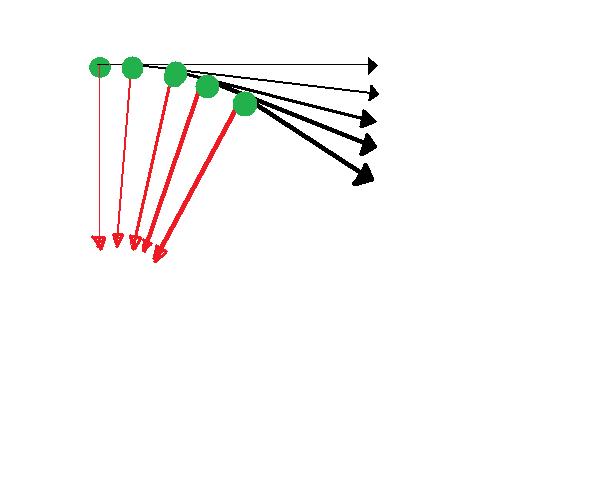
Notice that had there been no force, the ball would simply have moved in a horizontal straight line. The force 'forces' the ball to move in a circle. ;-) No force is required to 'balance' the centripetal force.
Also, in this example I would have to continuously change the direction in which I apply a force on the ball, so as to keep it moving in a circle. In your example, the gravitational force on the satellite is the centripetal force which keeps the satellite moving in a circle. It is always acting towards the center of the earth, so the 'adjusting' happens automatically as the satellite moves around the earth.
Centrifugal force
This one is a little more complicated. It's best explained through an example.
Imagine that you're sitting on a merry-go-round which is spinning in a circle. You are moving in a circle on the merry-go-round. Hence, there must be some force which is acting on you, pulling you towards the center of the merry-go-round. In the absence of such a force, you would fly off the merry-go-round. It's easy to guess that here, the centripetal force acting on you is composed of (1) the friction between you and the floor of the merry-go-round and (2) the force applied by the handlebars and other parts of the thing on you, as a result of you pushing against them.
Now this is the important part - you, sitting on the merry-go-round, believe that you are not accelerating. (A person standing on the ground knows that you are accelerating, as your velocity is continuously changing its direction thanks to the centripetal force acting on you). Since you believe that you are not accelerating, you believe that the net force acting on you is zero. Thus, you feel an outward force acting on you, which 'balances' the centripetal force provided by the handlebars and friction. This is the so-called centrifugal force.
This is true for all accelerating frames - anyone inside the frame thinks that they are not accelerating, and hence feels the effect of a "pseudo" force which acts opposite to whatever real force is acting on them and making them accelerate.
To summarize, centripetal force is a real force which must act on a body for the body to travel in a circle. It acts towards the center of the circle. Centrifugal force is a force felt by a body if it is sitting in a frame which is travelling in a circle. It acts away from the center of the circle.
-
$\begingroup$ Where does the velocity come if without any force being exerted on the object in its direction, $\endgroup$ Commented Dec 29, 2014 at 1:40
-
$\begingroup$ Also that outward force. So basically the reason the horse in the circus running in a circle not does not get pulled into the center of circle is that the horse exert a opposite force onto the ground?? $\endgroup$ Commented Dec 29, 2014 at 1:43
$\begingroup$
$\endgroup$
You're actually thinking that the satellite's momentum is keeping it in orbit. A huge man-made force (a rocket) put the satellite in orbit, this orbit is actually the horizontal velocity (relative to the earth's surface) that the satellite is travelling in.
Remember: If there was a rock at the same height as the satellite with no horizontal velocity, it would fall directly back to earth.
Satellites usually travel at very high absolute speeds, around 10 km s$^{-1}$. Depending on the orbit type, the direction of the velocity is not quite perpendicular to the radius. Note that the earth's radius is 6,371 km. So you have a satellte going 10 km s$^{-1}$ around an object about 600 times its length. Remember that gravity is an acceleration downward of 9.8 m s$^{-2}$, or about 0.01 km s$^{-2}$. So after 100 seconds of gravity working on the satellite it has travelled about the same order of magnitude as the body that it is orbiting. If the satellite travels further than the radius of the body (in this case, Earth( in the time gravity pulls down on it then it remains in orbit.
So there is a certain speed called the "escape velocity" that the satellite needs to be moving faster than to remain in orbit, otherwise it slowly spirals down to earth.
$\begingroup$
$\endgroup$
Looks like you are thinking that motion should be along the line on which the Net Force lies as well as in the direction of Net Force.
This is not necessary. Force is only supposed to change the velocity of the body. The resulting velocity is not necessarily/always along the line of acceleration i.e, change of velocity. The Centripetal force create change in the instantaneous velocity and the Final velocity is again along the tangential direction of instantaneous location of the body along circular path.
If you want to fall the body towards the center of the circular path while it is performing circular motion, you shall have to apply a force which gives you the final velocity directed towards the center of the circular path. In that case your force can not be called "Centripetal Force" because of its definition.