When a rod inclined to a wall slips, rate of change of which angle does the angular velocity represent? Is it the rate of change of angle with which the rod is inclined to the horizontal ? I'm not able to visualize it. In pure rotation it is quite simple to see which angle is changing and hence the angular velocity. Also, is centre of mass of the rod the axis of rotation? If so how would the motion of rod look when observing from the axis. It doesn't appear seem to be like a circle, though I'm only saying it through my intuition. A diagram perhaps would help in my visualisation.(Assume no friction anywhere)
$\begingroup$
$\endgroup$
1
-
$\begingroup$ The instantaneous centre of rotation is not the centre of mass of the rod. It is the point at the intersection of the vertical line through B and the horizontal line through A. This is because the ends or the rod are moving tangentially along the floor/wall, so the instantaneous centre of rotation is perpendicular to both at the points of contact. $\endgroup$– sammy gerbilCommented Jan 14, 2017 at 3:47
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
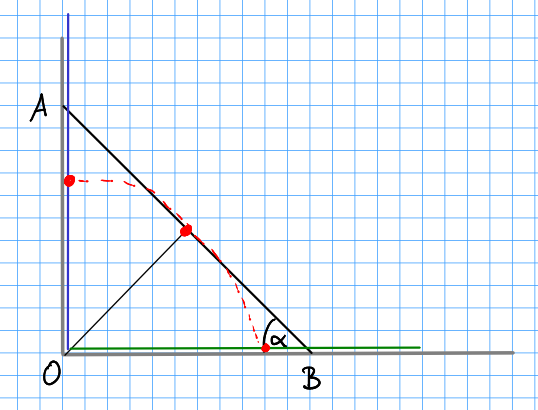
Let $\alpha$ be the angle between the rod and the floor at point $B$ (see my drawing). Then we can write the points $A$ and $B$ with the length $L$ of the rod as $$ \vec A = L \sin(\alpha) \begin{pmatrix} 0 \\ 1 \end{pmatrix} \,,\qquad \vec B = L \cos(\alpha) \begin{pmatrix} 1 \\ 0 \end{pmatrix} \,. $$
The center of gravity $M$ of the rod is just the average of $A$ and $B$. It is $$ \vec M = \frac{\vec A + \vec B}2 = \frac L2 \begin{pmatrix} \cos(\alpha) \\ \sin(\alpha) \end{pmatrix} \,. $$
From this you can see that this midpoint of the rod will describe a circle when the rod slides down. My drawing is not very good, but the rod starting vertically (blue) slides down (black) and finally lies on the floor (green). The midpoint (red) describes a circle.
The angular velocity should just be $$ \omega = \frac{\mathrm d}{\mathrm dt} \alpha \, $$ nothing more. The motion of the rod is translation as well as rotation. The rotation around any point can always be separated into a center-of-mass motion and a center-of-mass rotation.
answered Feb 1, 2016 at 16:28
-
$\begingroup$ Maybe you should add that at some point, if there is no friction, point A loses contact with the wall and the movement of the center of mass is no longer a circle. $\endgroup$– DimitriCommented Feb 1, 2016 at 16:38
-
$\begingroup$ I want to know how the motion of rod would look when viewing from the frame of center of mass of the rod and not the motion of the center of mass from ground. $\endgroup$ Commented Feb 1, 2016 at 16:58
-
$\begingroup$ In the center of mass frame you would only see the rotation. This frame is attached to the red dot. You would just see the rod being rotated by $\alpha$. Or are you interested in the exact time evolution of this motion? $\endgroup$ Commented Feb 2, 2016 at 17:52