Why there is a factor of 4 in van der Waal's Volume Correction?
-
$\begingroup$ In book it has written that molecules have sphere of influence $\endgroup$– SireesCommented Dec 8, 2015 at 8:25
-
$\begingroup$ Same thing I am not understanding $\endgroup$– SireesCommented Dec 8, 2015 at 8:30
-
$\begingroup$ Please be more specific. Which correction are you talking about (including the equation into the post would already help a lot), what do you not understand about it? $\endgroup$– ACuriousMind ♦Commented Dec 8, 2015 at 13:40
-
$\begingroup$ There's also some explanations of this here: physics.stackexchange.com/questions/119568/… $\endgroup$– AldenCommented Jan 27, 2017 at 13:23
1 Answer
Consider one mole of gas composed of non-interacting point particles that satisfy the ideal gas law:
$$p=\frac{RT}{V_{m}}=\frac{RT}{v} $$
Next assume that all particles are hard spheres of the same finite radius r (the van der Waals radius). The effect of the finite volume of the particles is to decrease the available void space in which the particles are free to move. We must replace $V$ by $V − b$, where $b$ is called the excluded volume or "co-volume". The corrected equation becomes:
$$p=\frac{RT}{V_{m}-b} $$
The excluded volume $b$ is not just equal to the volume occupied by the solid, finite-sized particles, but actually four times that volume. To see this, we must realize that a particle is surrounded by a sphere of radius $2r$ (two times the original radius) that is forbidden for the centers of the other particles. If the distance between two particle centers were to be smaller than $2r$, it would mean that the two particles penetrate each other, which, by definition, hard spheres are unable to do.
The excluded volume for the two particles (of average diameter $d$ or radius $r$) is:
$$b'_{2}=4\pi\frac{d^{3}}{3}=8\cdot(4\pi r^{3}/3) $$
which divided by two (the number of colliding particles) gives the excluded volume per particle:
$$b'=b'_{2}/2 \rightarrow b'=4\cdot (4\pi r^{3}/3) $$
So $b′$ is four times the proper volume of the particle. It was a point of concern to van der Waals that the factor four yields an upper bound; empirical values for $b′$ are usually lower. Of course, molecules are not infinitely hard, as $\textit{van der Waals}$ thought, and are often fairly soft.
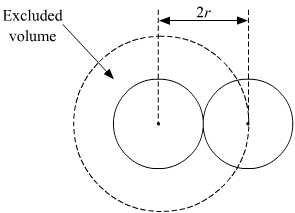
Source: Wikipedia
-
-
-
$\begingroup$ As we considered this for one mole but one mole consist 6.023*10^23 molecules $\endgroup$– SireesCommented Dec 8, 2015 at 9:38
-
5$\begingroup$ What is the logic behind the reasoning that we must divide the obtained value by 2 since two particles are colliding? And really, why do we consider the whole sphere, when clearly, other molecules can still occupy some portion of this sphere and is not completely "excluded"? $\endgroup$– user106570Commented Aug 24, 2016 at 9:01
-
$\begingroup$ See my answer here to get some idea of why the value must be divided by 2: physics.stackexchange.com/a/307924/143458 $\endgroup$– AldenCommented Jan 27, 2017 at 13:26
