Van der waals equation why should we divide excluded volume by 2?

Question

So been looking at the correction term $b$ for the Van Der Waals equation. I understand that we look for the excluded volume. I see it as the volume where no (center of a) particle can enter.

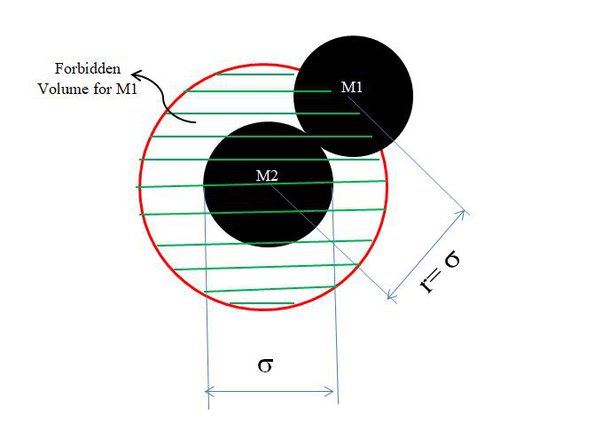
We start by looking at 2 molecules/atoms with radius $r$, as on the picture below.

I understand that M1 cannot enter the sphere with radius $2r$ centered around the center of M2. Apparently this is the excluded volume for the 2 molecules, so now we have to divide this volume by 2 to get the excluded volume per molecule. That's the problem: I don't understand why this sphere is the excluded volume for 2 molecules.

The way I see it, the sphere with radius 2r around the center of M2 is the excluded region for the center of M1. The center of M1 can not enter this volume.

In my eyes M2 is excluded as well, from a sphere with radius 2r centered at the center of M1. Then we would get the union of these 2 spheres: volume of them both - the overlap between them. This would then be the excluded volume for the two particles. Then to get the excluded volume per particle you would divide this by 2.

I am missing something and would like some help with wrapping my head around this.

Answer 1

To understand what is equal to $b$, you should consider the system from the prospective of one molecule. Then you will be able to use that for all other molecules as well. Let's find the volume which a molecule cannot occupy. As you said previously, that would be the volume of a sphere of radius $2r$ (or just $r$ if we look at the picture) multiplied by the number of molecules, meaning "the volume of a sphere of radius $2r$ per molecule". Look that you don't need to subtract the volume of one molecule from it as, from the prospective of the chosen molecule, it cannot occupy this volume either. And as I understand, by that logic you don't need to divide the volume by two.