1
$\begingroup$

I am not being able to answer, where is actually the force of gravity directed towards ?

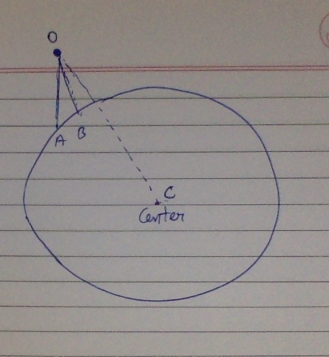
Look at this picture.

enter image description here

This is a planet with the centre ‘C’. And O is an object. Every point of the planet is attracting the object,The points A and B are also pulling it towards themselves, then why do we say, the force is directed towards the centre?

Also, If i drop the object, will it move towards A , B or towards the centre ?

Improve this question
$\endgroup$
1
  • $\begingroup$ And once you have your head around this, here's another one: If O is at some radius r inside the planet, it works out that all the effects of the points of the planet further out than r cancel out and the total gravity experienced is the same as if the planet were only of radius r. $\endgroup$
    – Keith
    Commented Nov 18, 2015 at 0:55

3 Answers 3

Reset to default
9
$\begingroup$

You have to think of the symmetry of the situation. For each point A and B, there is a point A' and B' opposite the center line that has an attractive force pulling in such a way that the components perpendicular to the center line are canceled. Therefore, the net force is along the center line.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Can you please elaborate ? I cannot understand you. $\endgroup$
    – Aaryan Dewan
    Commented Nov 17, 2015 at 17:24
  • 2
    $\begingroup$ @AaryanDewan Think about it this way, draw a point A' the same distance away from the center line but on the opposite side of A. There is nothing special about the point A that should draw the object to that point. A' is attracting it in the same fashion. If you were to flip the situation about the center line, A' would become A, so you shouldn't expect it to be attracted to one or the other. They both pull the object down toward the center line in the same way however. I can explain more if necessary. $\endgroup$
    – tmwilson26
    Commented Nov 17, 2015 at 17:29
  • $\begingroup$ Please explain your first line. Where do i have to locate the point A’ ? What is the centre line? The line joining O and the centre? $\endgroup$
    – Aaryan Dewan
    Commented Nov 17, 2015 at 17:35
  • $\begingroup$ @AaryanDewan Yes, the center line is the line you've drawn connecting $C$ to $O$. The point $A'$ would be the same distance from $O$ as $A$ and at the same angle from the centerline, but on the opposite side. They both pull the object toward them with equal magnitude of force. So, given their symmetry, they can't pull the object away from the center line. This is true for any point that you choose off of the centerline. $\endgroup$
    – tmwilson26
    Commented Nov 17, 2015 at 17:41
  • 3
    $\begingroup$ @AaryanDewan Well, in that case, you need to sum the contributions from every point inside the planet, depending on what effects you want to take into account. Then the orientation of the planet will be important as well. If you want to consider these things and perhaps others (air resistance, rotating earth, etc.), you should clarify that in your question. When we say that an object's gravity will act as a point mass with all of the mass at its center, the assumption is that of a spherical object. $\endgroup$
    – tmwilson26
    Commented Nov 17, 2015 at 18:01
2
$\begingroup$

As @tmwilson26, said the net force is directed along the center, here's the mathematics of it

if there is a small differential mass at A, there also exists another point A' laying just opposite to the center line(making a same angle $\theta$ with the center line)

The force $F$ experienced at point O has 2 components $F_x$ and $F_y$ where

where $F_y$ is along the radial direction and $F_x$ perpendicular to it

due to symmetry the $x$ components cancel out since they have equal and opposite directions, which leaves behind the $y$-component which is the radial component

Improve this answer
$\endgroup$
1
$\begingroup$

If the planet is spherically symmetric, the gravitational field is the same as if all the mass were concentrated at the center, so the attraction will be along the line toward the center. If it is not, it is not really fair to talk of the center. Imagine if there were a huge mountain at point A. This could change the gravitational field (a small bit) to pull the object more toward A. The equatorial bulge of the earth tends to pull satellites into the equatorial plane. You get a more complicated gravity model, which matters to some things and not to others.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.