1
$\begingroup$

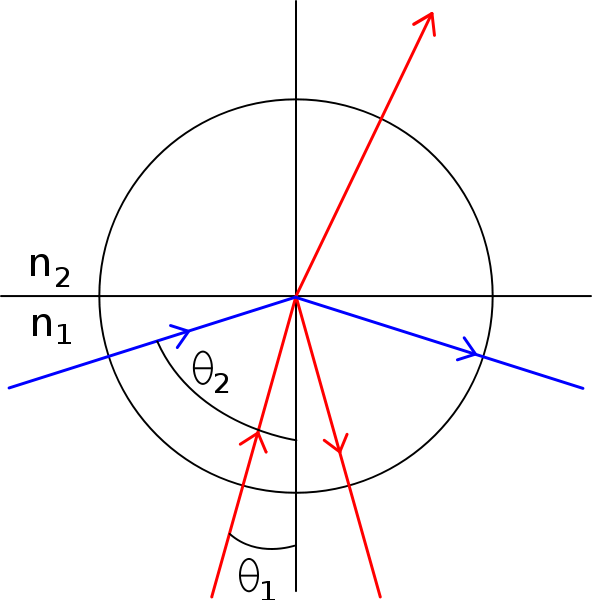
I have difficulties visualizing following task

A diver is $d = 10 m$ below the water surface ($n_{water} = 1.33$). What is the diameter of the circle through which the diver can see the sky?

That's all the information there is. What is the approach here? I find it strange that the range of vision is not considered.

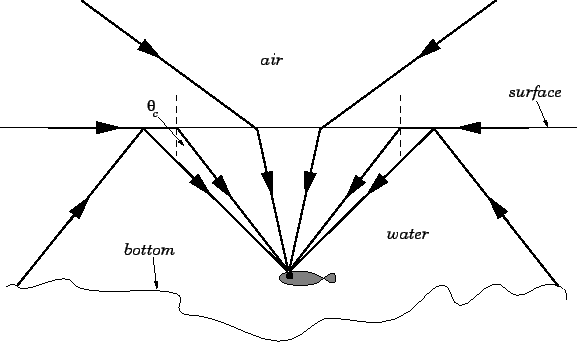
Inspired by the comments I've added a sketch from wikipedia

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Would "angle of total internal reflection" ring a bell ? ;-) $\endgroup$
    – Fabrice NEYRET
    Commented Oct 25, 2015 at 15:40
  • 1
    $\begingroup$ The question is about calculating the total internal reflection at the water surface and what that means for the ability of the diver to see what's happening above. You are required to assume a perfectly flat water surface, of course, otherwise ripples will allow the diver to see more than just this circle (even though his vision will be greatly distorted). $\endgroup$
    – CuriousOne
    Commented Oct 25, 2015 at 15:40

1 Answer 1

Reset to default
2
$\begingroup$

Hint: This is the sketch that you need to solve the problem- enter image description here

The angle of vision of the diver causes him to view the external world through a circular patch. This is the circle whose radius you want.
Now you just need to apply Snell's Law for the critical angle condition and solve it.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.