HOW is electric field inside a conductor placed in an external field zero?

Question

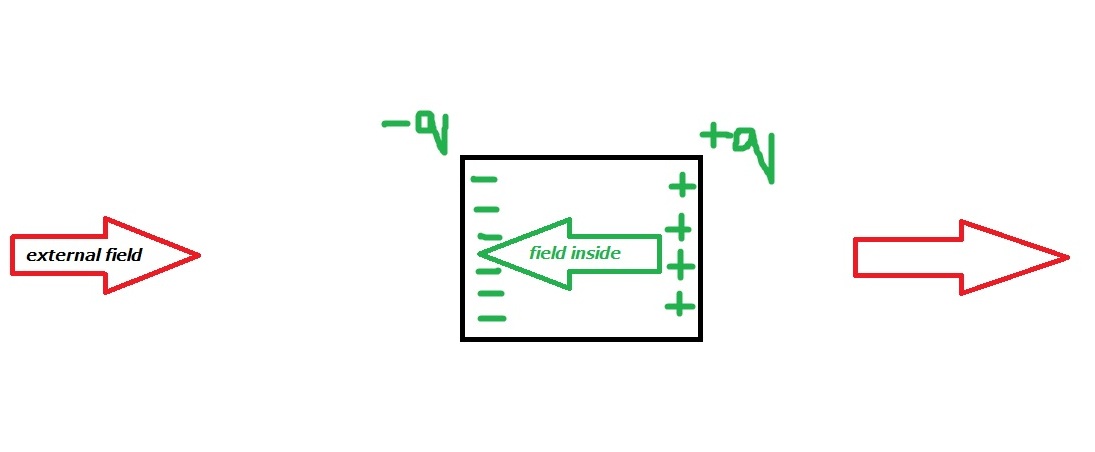
Lets assume that there is an external field +E acting on a conducting plate from left to right. Due to this, some electrons will drift from right to left to cancel out this electric field inside the conductor. But since some electrons have moved to the left surface of the sheet, there should(?) be an accumulation of positive charge on the right side of the sheet which should also have an electric field of its own (will this accumulation of positive charge on the right side of the sheet be equal in magnitude to the charge due to the accumulation of electrons on the left side ?). So how is the net electric field ever getting zero because there will always be some positive charge in the conductor which will have a field of its own. If maybe this field attracts some electrons to neutralize it, there will always be some positive charge due to this attraction of electrons to have a field of its own. Please tell me what exactly happens inside the conductor since my above idea is probably wrong.

I know that "its electrostatics so no field can move and thus field should be zero" but I want to know HOW this happens and no other question addresses this "HOW" or my concern about the positive charge build up (if there is any kind of buildup)

Can a conductor run out of electrons to cancel external electric fields? ^This answer talks about the electrons neutralizing a positive field by drifting to one side but then it loses context to what I want to know by talking about the impact of the external field strength (I want to know the impact of the field created by the positive charge inside the conductor, if there is any)

Why the electric field inside a conductor is zero? ^The answer in this thread talks about the negative charge moving (as i have already mentioned above) but doesn't tell whether there is any kind of positive charge build up on the opposite side and the field due to it (because the electrons have moved to the other side)

Answer 1

Take a metal block and place it in a uniform electric field which points from left to right, since the metal block has free electrons they will move opposite to the field, that is, to the left side of the conductor.Since the block is electrically neutral if a charge of -q appears on left side an equal positive charge +q must appear on right side. This will create another field inside the block from right to left.The movement of electrons will continue till the electric field inside the conductor is equal and opposite to the electric field outside the conductor after that the movement will cease as the field inside the conductor will become zero. The field produced inside is the net field of negative and positive charges induced in the conductor(here metal block).

Answer 2

But since some electrons have moved to the left surface of the sheet, there should(?) be an accumulation of positive charge on the right side of the sheet which should also have an electric field of its own (will this accumulation of positive charge on the right side of the sheet be equal in magnitude to the charge due to the accumulation of electrons on the left side ?). So how is the net electric field ever getting zero because there will always be some positive charge in the conductor which will have a field of its own.

An infinitely thin surface charge of density $\sigma$ in the $yz$-plane induces an electric field which in SI units is $$\vec E_\sigma = \frac{\sigma}{2\epsilon_0} \hat x \operatorname{sgn}(x).$$ Here $\operatorname{sgn}(x) = x/|x|$ is $+1$ if $x$ is positive, $-1$ if $x$ is negative, or $0$ if $x$ is zero. The key point is that it does not diminish with distance.

As a result, if a block of conductor has a surface charge $+\sigma$ on one side and a surface charge $-\sigma$ on the other side, there is a uniform field $\vec E_c = \pm (\sigma/\epsilon_0) \hat x$ between them (constructive superposition), but zero field outside (destructive superposition).

Now suppose we fill all space with some field $\vec E_0.$ The conductor forms the two surface charges so that $\vec E_c + \vec E_0 = \vec 0.$ By superposition, there is now no net field in the conductor. As a bonus result, the field outside of the conductor is doubled due to the effect of the conductor.

Answer 3

Let’s begin by underlining the condition in which the electrostatic field inside a conductor is zero and the condition is “static” or “steady state”. Consider that we are not the yet there and that we still have an electric field different than zero inside the conductor, in which case, any positive charge carrier will be forced to move in the direction that the field vector points to or, if negative, contrary to that direction, in both cases, the new positions of these charges tend to compensate the very same field that just moved them. The charged carriers in a conductor will keep moving until the electric field is zero and only then is that this electric field qualifies as electrostatic.