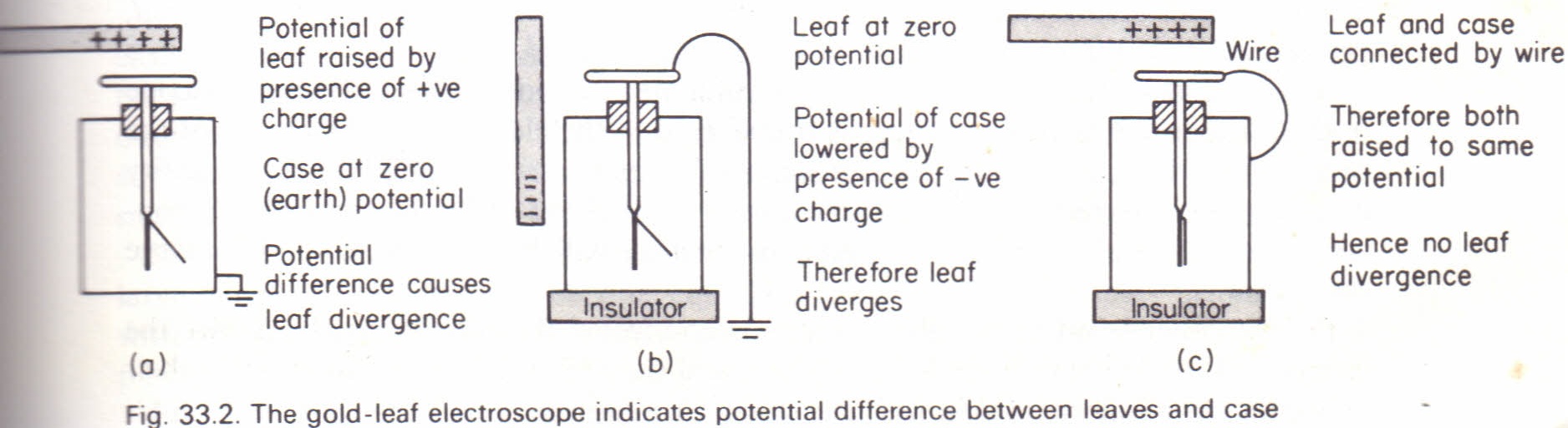
An Electroscope consists of a lightweight, conducting needle hinged to a conducting pole. If the pole is charged, the needle will deflect from its zero-point because it is charged the same way as the pole.
I now read - and also verfied myself - that an electroscope measures not only charge but also VOLTAGE. This was a real surprise, and I still don't understand why. Here's the experimental setup:
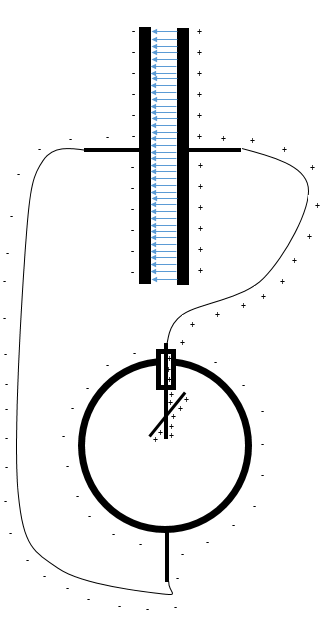
A plate capacitor is charged electrostatically and connected to an electroscope:
As expected, the electrostatic charge on the positive plate will deflect the electroscope needle.
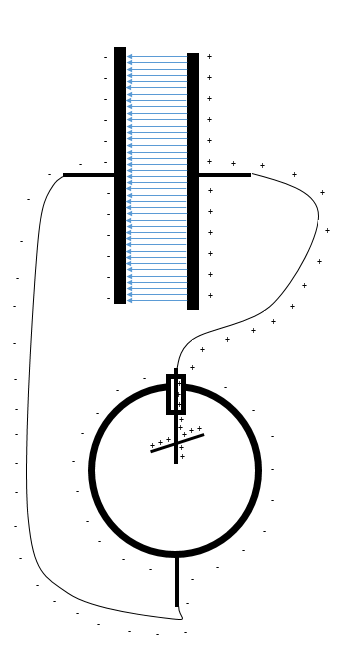
I now proceed to move apart the two plates of the capacitor. This will not change the charge on the plates nor will it affect the field between the plates, but it will change the Voltage across the plates: The Capacity of the Capacitor changes, the charge stays the same, so the Voltage will change. Or, stated differently, the charges on the plates are separated even further by pulling the plates apart and the resulting work increases the voltage. Here's the math:
$$C=\frac{Q}{U}\Rightarrow U=\frac{Q}{C}$$
where:
C: Capacitance
Q: Charge
U: Voltage
The Capacitance of a plate Capacitor with Surface Area A and plate Distance D is given by:
$$C=\frac{\epsilon_{0}A}{D}$$
$\epsilon_{0}$ is the vacuum electric permeability.
Inserting this into the above equation, the Voltage is then given by:
$$U=Q\frac{D}{\epsilon_0A}$$
If D increases, U will increase. But Q will remain the same, and also the Electrical Field between the Capacitor Plates:
$$E=\frac{U}{D}=\frac{Q}{\epsilon_0A}$$
However, the Electroscope needle moves!
How is this possible? The charge on the plates remains the same, and so does the force field - the charges should not shift around in the capacitor or the connected electroscope. But the electroscope needle moves, indicating that the capacitor now has a higher voltage. But how would it know that?