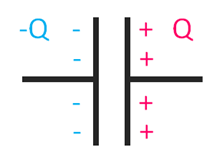I'm not sure I got Faraday's capacitor and electroscope experiment

Question

I was reading Feynman's lecture 10 on the Lessons on Physics vol. 2 book, in which he talks about dielectrics.

https://www.feynmanlectures.caltech.edu/II_10.html

(...) However, using a simple electroscope and a parallel-plate capacitor, Faraday discovered that this was not so. His experiments showed that the capacitance of such a capacitor is increased when an insulator is put between the plates. (...)

I'm trying to understand Faraday's experiment with a capacitor and an electroscope.

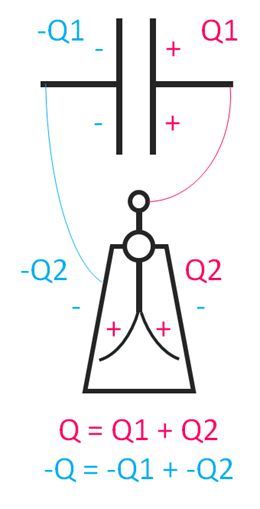
Let's say I have a capacitor charged with charge Q.

And then I connect an electroscope in parallel with the capacitor.

Since both are conductors and connected to each other, the charges will redistribute among them until both reach the same electric potential.

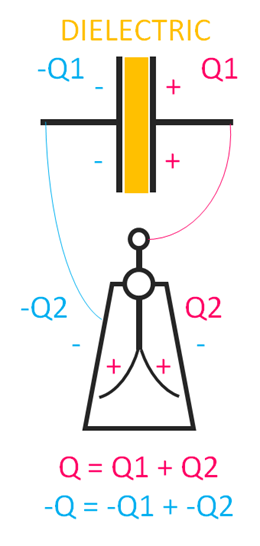
Now comes the part that gets me confused, when we insert a dielectric between the capacitor's plates.

To my understanding, $C1$, which is the capacitor's capacity, is given by

$$ C1 = \frac{Q1}{V} $$

and, since we know experimentally that $C1$ increases, then we can deduce that either $Q1$ increases or $V$ goes down.

We know for sure that $V$ goes down. And, since both the capacitor and the electroscope are at the same electric potential, then $V'$ (smaller $V$) is the same for both.

But Feynman says that Faraday found experimentally that the electroscope's reading goes down! Therefore, charge $Q2$ has to decrease (because it's the charge on the electroscope) and $Q1$ has to increase to such a ratio that

$$ V' = \frac{Q1'}{C1'} = \frac{Q2'}{C2'} $$

still holds true.

Therefore, $Q1$ absolutelly is going to change, as will $Q2$. It doesn't stay constant, even though $V$ has changed.

Is my reasoning correct? Because at the lecture Feynman says that $Q$ stays constant, but I guess in his hypotetical example there's no electroscope attached to the capacitor.

Answer 1

Here is the complete part of the exert from Feynman.

One might at first believe that there should be no effect whatsoever. However, using a simple electroscope and a parallel-plate capacitor, Faraday discovered that this was not so. His experiments showed that the capacitance of such a capacitor is increased when an insulator is put between the plates.

Because at the lecture Feynman says that Q stays constant - I do not see that in his text.

You are quite with your analysis in that the charge stored in the capacitor is shared with the electroscope because that too has a capacitance.

$Q_{\rm capacitor,initial} = Q_{\rm capacitor,final} + Q_{\rm capacitor,final}$.

Thus connecting an electroscope to the capacitor does decrease the voltage across the capacitor plates from $\frac{Q_{\rm capacitor,final}}{C_{\rm capacitor}}$ to $\frac{Q_{\rm capacitor,initial}}{C_{\rm capacitor}}$.
That did not stop Faraday showing that adding a dielectric did have an effect as mentioned by Feynman.

How much the electroscope changed the charge on the capacitor depends on the relative sizes of the capacitance of the capacitor and the electroscope.

You might find it interesting to know what Faraday actually did and not take the statement, using a simple electrometer and a parallel-plate capacitor too literally.

For a start Faraday used a torsional (balance) electrometer which was a modified version of the apparatus which Coulomb used when investigating the inverse square law for electrostatics. Faraday writes The measuring instrument I have employed has been the torsion balance electrometer of,. Coulomb, constructed, generally, according to his directions . . .

He used a concentric sphere capacitor whose capacitance with air in it was about $9\,\rm pF$ although of course he did not know the value! Here is his diagram of it with my annotations to make the labelling clearer.

Two concentric spheres a and h, and insulated handle l with a conducting rod i passing through the middle to a sphere B at the top to enable the capacitor to be charged.
The seal between the top part (see left diagram) and the bottom was good enough so that Faraday could investigate the dielectric properties of gases and liquids as well as solids.

Using his apparatus Faraday measured the specific inductive capacity, the ratio between the capacities of two condensers of the same size and shape, one of them filled with the specified dielectric, and the other an air condenser.

Another interesting things is he added a constant amount of charge using a Leyden phial (jar) charged to the same voltage which was checked by using a spark gap voltmeter, A Leyden phial is to be charged to such a degree that it would give a spark of about one-sixteenth or one-twentieth of an inch in length between two balls of half an inch diameter.

You can download a compilation of Faraday's papers from the Archive.org website with the relevant page 360 of the text, pdf page 373, starting at section 1161 [360,373,1161].
The electrometer [368,381,1180], capacitor [371,384,1188], numerous precautions [375,388,1197], procedure [378,391,1208] and finally the results [380,393,1215] are all described in amazing detail when you consider the experiments were done around 1837.