In the Faraday pilot-wave fluid droplet dynamics, the fluid wave is meant as an analogy for the wavefunction. More specifically, the experiments are constructed as physical implementations analogous to the de Broglie-Bohm theory, where a particle with discrete coordinates is 'guided' by a pilot wave which follows the Schrödinger equation.
To be a bit more explicit, the de Broglie-Bohm theory works with a standard wavefunction $\psi(\mathbf q,t)$ on a single- or multi-particle configuration space $\{\mathbf q\}$, which obeys the Schrödinger equation
$$
i\hbar\frac{\partial}{\partial t}\psi(\mathbf q,t)=-\frac{\hbar^2}{2m}\sum_i\nabla_i^2\psi(\mathbf q,t)+V(\mathbf q)\psi(\mathbf q,t).
$$
This wavefunction then guides an actual 'particle' with coordinates $\mathbf q(t)$ on the configuration space (so it may represent the coordinates of multiple particles) by matching its momentum to the local momentum of the wavefunction, understood as
$$
m_k\frac{\mathrm d\mathbf q_k}{\mathrm dt}=\hbar \operatorname{Im}\left(\frac{\nabla_k\psi(\mathbf q,t)}{\psi(\mathbf q,t)}\right)
$$
for the $k$th particle. The particle is then distributed on the configuration space according to $|\psi(\mathbf q,t_0)|^2$ at an initial time $t_0$, and it retains that distribution; upon measurement it is the configuration-space particle that gets detected.
The water droplets behave similarly but not exactly in this way. The fluid surface acts in a wave-like way, and it influences the particle-like droplet, exchanging energy and momentum with it. The analogy is, however, nowhere near exact, and it is described in considerable (but still readable) detail in
Faraday pilot-wave dynamics: modelling and computation. P.A. Milewski et al. J. Fluid Mech. 778 361 (2015).
In short, the droplet moves balistically between bounces, and during the bounces its horizontal component $\mathbf X(t)$ obeys the Newton equation
$$
m\frac{\mathrm d^2\mathbf X}{\mathrm dt^2}+\operatorname{drag}(t)\frac{\mathrm d\mathbf X}{\mathrm dt}=-F(t) \nabla \overline\eta
$$
where $\overline\eta=\overline\eta(x,y)$ is the fluid surface height you'd have if the droplet wasn't bouncing.
This means, to begin with, that there's two big differences between the Faraday-wave droplets and the de Broglie-Bohm theory. For one, the wave's influence on the mechanical system is of a rather different character. To tack on to this, the droplet can influence the Faraday wave, which is unthinkable in Bohmian mechanics.
So, to summarize: the liquid wave is an (imperfect) physical analogue for the Bohmian wavefunction. What is the fluid surface itself an analogue for? Nothing. That's an over-reading of the analogy. It is just an analogy, and not all elements of the analogy need to mean something on the other side. The status of the analogy is well summarized by J.W.M. Bush in the introduction of
The new wave of pilot-wave theory. J.W.M. Bush. Physics Today 68 no. 8, 47 (2015). U. Arizona eprint.
The bouncing-droplet experiments are indeed important. They show that particle-like system can indeed display wave-like behaviour, like two-slit interference or mode quantization, from more fundamental interactions which we might have missed at first. In this sense, they are an encouragement to keep looking for a Bohmian-like explanation for quantum mechanics.
On the other hand, bouncing-droplet experiments are fundamentally limited. They have a hard time simulating the three-dimensional motion of a single-particle, and they mostly cannot simulate the quantum mechanics of multiple particles (even two particles on one dimension) since the wavefunction is a wave on the (#particles)$\times$(#dimensions)-dimensional configuration space. In other cases, such as Mandel dips, the quantum wave interference occurs over even more abstract spaces.
The bouncing-droplet experiments, like other quantum analogues such as elastic rods, are therefore not proof of anything, and they have no new implications on the foundations of quantum theory. They are an interesting testing ground, and it would be good to see them take on interesting foundations issues, like Bell inequalities and contextuality, where they could produce interesting new questions and takes which could then be mirrored on the quantum side. As they are now, though, they're simply an interesting curiosity, I'm afraid.
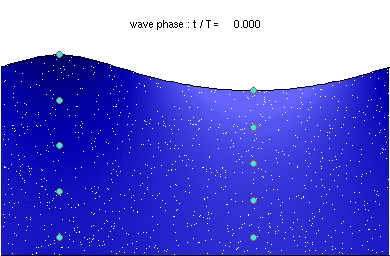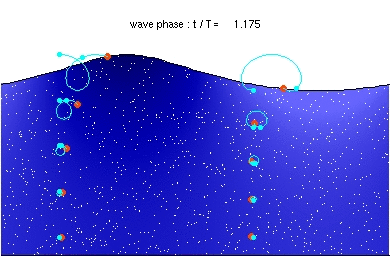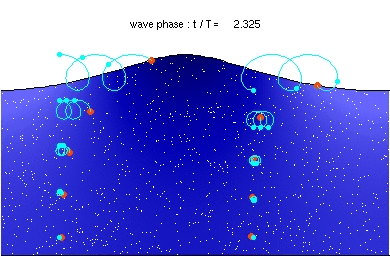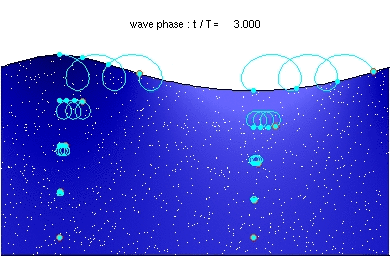GNUFDL image by Kraaiennest, see 
