Taking selfies while falling, would you be able to notice a horizon before hitting a singularity?

Question

I am generally interested in the role of "pings"^(0a) between participants (a.k.a. "signal roundtrips"^(0b), as familiar for instance from Synge's "five point curvature detector") in the determination of geometric relations;
and I am frequently missing their explicit consideration (e.g. from answers to questions such as this: "Would you notice if you fell into a black hole?" (PSE/q/187917) ). Therefore I'd like to ask a related question in which pings are plainly the main point of the setup description:

Consider, as a thought-experiment, a person who is falling^(1a), while taking a sequence of selfies, operating a convenient device^(0c) with a "front camera" and a "display" (or even several such devices, all separated from the face of the person under consideration). While taking these selfies the person under consideration is also directly reviewing^(0d) the resulting photiographs. Can this person notice anything "peculiar, associated with a horizon^(1b)" before hitting a singularity^(1c)?

^{(0abcd: Note that there is no explicit mentioning at all of signal "pings" or "roundtrips" in the question "Would you notice if you fell into a black hole?" (PSE/q/187917) by user3137702, nor in any of the answers submitted to that question.)}.

^{(1abc: Applicable (geometric) notions such as "to fall", "horizon" and "to hit a singularity" shall be presumed as used in this answer.)}.

---

EDIT

To whom it may concern:

Recently there has been some HTML code inserted at the top of my question; anonmously, without any apparent entry in the version history of my question, and without any notification given to my "inbox" ...

For the benefit of the anonymous editor who possibly failed to appreciate as a sufficient distinction that

• the question "How can anything ever fall into a black hole as seen from an outside observer?" (PSE/q/21319) is concerned with

  "an outside far-away observer [who] see[s] objects slowly fade and slowly disappear as they approach the event horizon"

• while my question is ostensibly concerned with

  "a person taking and reviewing a sequence of selfies"

... let me add the tag equivalence-principle to my question, which IMHO may be considered to have some sort of relevance to my question (while it is evidently presently absent from question PSE/q/21319).

Answer 1

The answer must be closely related to my answer in Thought experiment - would you notice if you fell into a black hole? You can certainly use a similar Eddington-Finkelstein coordinate diagram to consider it (the E-F coordinates transform away the coordinate singularity at the event horizon). NB: This considers only GR and a non-rotating black hole (and assumes that the black hole is not accreting so you don't fry). Note also that this is quite different to the case of a stationary observer outside the black hole; here the observer freefalls along with the event they observe.

I think it does depend on the radial distance between your face and the camera and the size of the blackhole. Looking at this diagram for a Schwarzschild black hole (in Eddington Finkelstein coordinates) we could construct pings made out of light travelling along the inward light cone boundary (where the null geodesic is always at 45 degrees) representing light travelling inwards from face to camera, immediately followed by light travelling back from the camera to the face, which would be represented by light travelling along the outward radial null geodesic defining the upper-right side of the light cone. [I am assuming here that the camera is radially further in than the face].

The worldlines of camera and face en-route towards the singularity in Eddington Finkelstein coordinates. Light cones are shown at two positions. The first where light is emitted radially inwards from face towards camera, the second radially outwards from camera to face.

In the example in the drawing, the separation between your face (head) and camera (feet) is small enough, that light emitted towards the camera from the face at the event horizon reaches it well before the camera reaches the singularity. This allows time for the return signal to reach the face. This would be a realistic proposition for a supermassive blackhole where you might have tens of seconds (of proper time) before reaching the singularity. [A stellar-sized black hole would rip your camera apart before you got near the event horizon.]

However, there will come a point, closer to the singularity, where the face and camera worldlines curve over to be almost parallel with the null geodesics of inward (and outward) light, so that light signals cannot make the round trip before your face hits the singularity. Someone smarter than me could do the maths to see algebraically where that it is for a free-falling observer and a given radial separation between face and camera.

There will be no discontinuity in behaviour at the event horizon.

A similar situation pertains if you dive in head-first. This second diagram shows the null geodesics from face to camera and then from camera to face for that case. Again, nothing changes abruptly at the event horizon, you can still take and see selfies as you pass through the event horizon and until some (proper) time as your face reaches the singularity. Thus in both cases, you can see the camera right up to the point of annihilation.

This shows the situation where you take the picture as your face crosses the event horizon with the camera outside the event horizon. The outward signal from your face travels vertically in Eddington-Finkelstein coordinates. Then the inward signal from the camera travels at 45 degrees and intercepts the worldline of your face before it reaches the singularity.

Answer 2

This is largely the same answer as Rob's, though rather than use Eddington-Finkelstein coordinates I'm going to use Kruskal-Szekeres coordinates because I think this makes the argument easier to understand. This is what the situation looks like in Kruskal-Szekeres coordinates:

For the non-nerd the Kruskal-Szekeres coordinates seem formidably complicated, but you don't have to fully understand them to appreciate what is going on. The green line shows your trajectory and the blue line shows the trajectory of the camera you are holding in front of you. The red curve is the world line of the singularity, so you hit the singularity when your (green) world line intersects the red one and the camera hits the singularity where the blue and red line meet.

The key thing that makes the SK coordinates so useful is that on this diagram outgoing light rays travel on straight lines at 45º from lower left to upper right. So the two pink lines I've drawn show two outgoing light rays. Now let's zoom in so we can see exactly what happens as you fall through the event horizon:

Point (a) is outside the horizon. So at point (a) you take a photograph and the light from the flash reaches you just as you'd expect. So far so good.

Point (b) is inside the horizon. However even inside the horizon you can see immediately from the diagram that the light from the flash at point (b) can still reach you. Inside the horizon the light from the flash cannot move outwards and is doomed to hit the singularity. However you (the green line) are falling inwards faster than the light is, so you and the light from the flash can still meet.

However at point (c) the light from the flash cannot reach you because it hits the singularity first. Therefore at point (c) the light from the flash can't reach you and there is an (apparent) horizon in between you and the camera.

So this answers your question. As you first fall through the horizon you wouldn't notice anything special. You'd still be able to take your selfies. However at some point an apparent horizon would form between you and the camera and you would notice this. The solution would be to bring the camera nearer to you so the flash light could still reach you. However as you approach the singularity you'd need to bring the camera nearer and nearer to keep taking pictures. At th singularity itself the spacing between you and the camera would need to be zero.

Answer 3

If the singularity is spacelike (as in a Schwarzschild black hole) then the answer is no, simply because the singularity is in the future. Information about the singularity would only be available in the future light cone of the singularity, and there is none because the singularity is the end of time. You won't see the destruction of the camera because it is spacelike separated from the destruction of you.

For a question like this, where only causality matters and not tidal forces, you don't need to worry about curved spacetime or general relativity at all: there are special-relativistic analogues of the situation that preserve all of the important features. My answer to that other question covers this with a setup involving synchronized time bombs.

If the singularity is timelike, as in the charged and/or rotating vacuum solutions, then it can be detected and studied in the same way as any ordinary object. (But not by taking selfies.) It can also be avoided like any ordinary object. These solutions probably aren't realistic, though, since they are also (if you avoid the singularity) traversable wormholes.

Answer 4

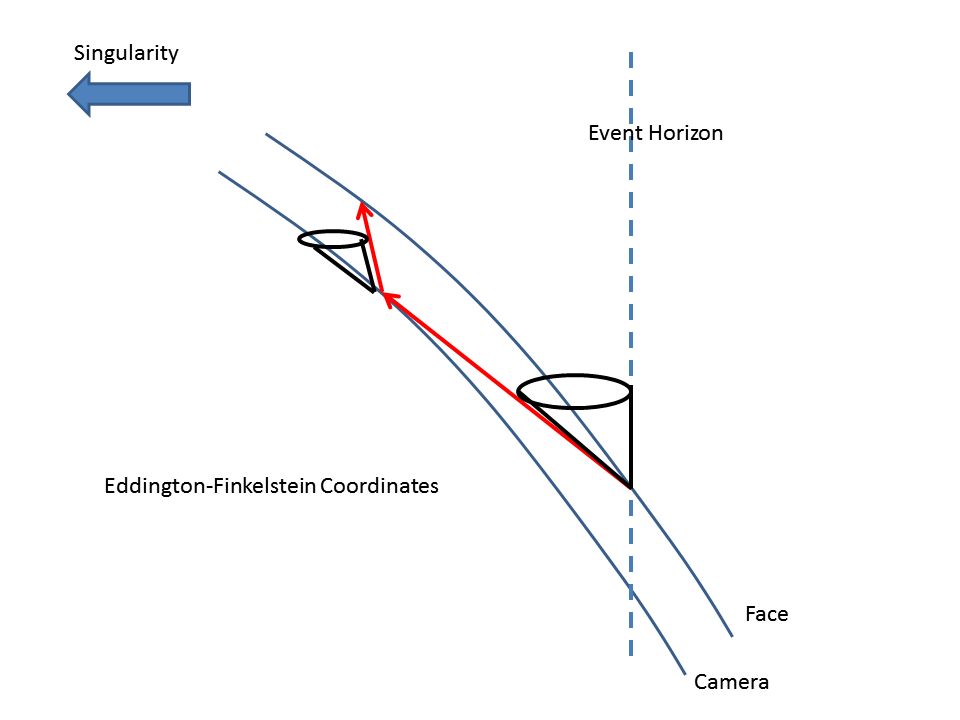
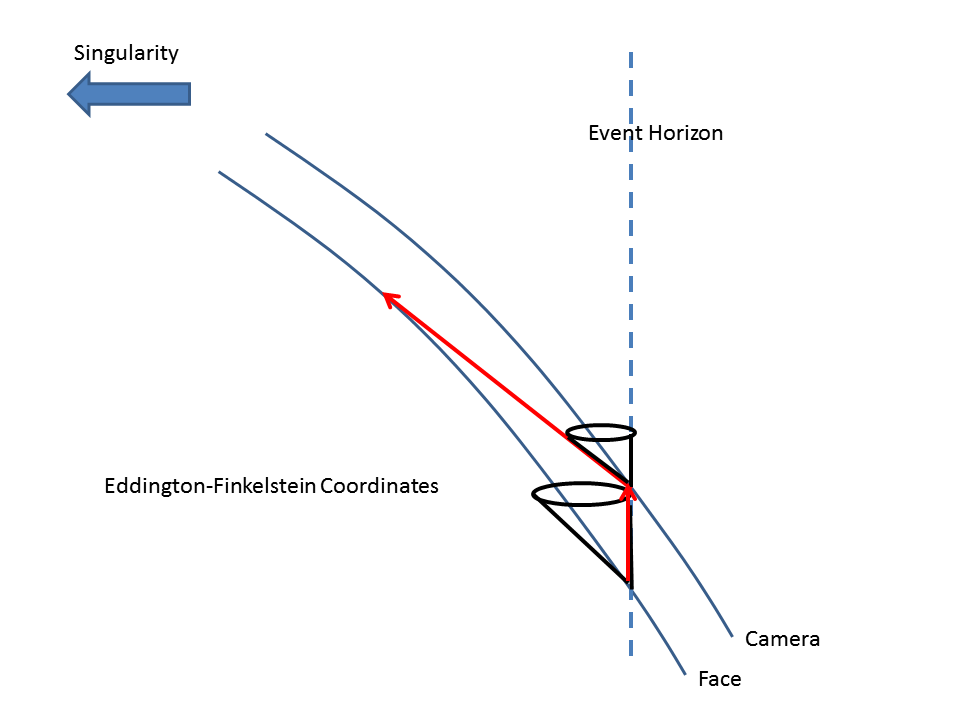
For trying to give a conclusive answer it seems necessary to rigorously characterize the geometry (the "conicidence structure", incl. the "light-cone structure") of the region under consideration (arguably with exception of "the singularity itself"). Unfortunately, this seems complicated (as may be gathered from efforts to tackle related problems at least approximately). Therefore the following only gives the outlines of an argument for a special case.

Let's consider one smartphone ($\mathsf A$) "falling freely" and "radially (towards the singularity)", and another smartphone ($\mathsf B$; "in the other hand", separated from $\mathsf A$) "moving radially" as well, and such that $\mathsf A$ and $\mathsf B$ remain "parallel (in the sense of Marzke-Wheeler)" throughout. (This condition can presumably be satisfied based on the otherwise undefined notions "radial" and "free fall", where the latter appears explicitly in the M-W definition of "parallelism", too.)

Further, Person ($\mathsf P$) shall move along such that throughout

• $\mathsf P$ finds coincident pings wrt. $\mathsf A$ and $\mathsf B$;
  in other words: for each of $\mathsf P$'s indications (such as any particular "facial expession" of $\mathsf P$) $\mathsf P$ observed/reviewed that smartphone $\mathsf A$ had observed and in turn displayed/reflected this indication of $\mathsf P$ and in coincidence $\mathsf P$ observed/reviewed that smartphone $\mathsf A$ had observed and in turn displayed/reflected this same indication of $\mathsf P$,

• $\mathsf A$ finds coincident pings wrt. $\mathsf P$ and $\mathsf B$;
  in other words: for each of $\mathsf A$'s indications (such as any particular "flash signal" of $\mathsf A$) $\mathsf A$ observed/took the picture (in coincidence) of both $\mathsf P$ and $\mathsf B$ reflecting this indication of $\mathsf A$, and likewise

• $\mathsf B$ finds coincident pings wrt. $\mathsf P$ and $\mathsf A$.

Moreover, let's require throughout (provided it can be satisfied at all) that $\mathsf P$ and $\mathsf A$ are M-W-parallel to each other, and that $\mathsf P$ and $\mathsf B$ are M-W-parallel to each other, too.

The Marzke-Wheeler construction of (the definition how to measure) "parallelism" of a pair of suitable participants involves reference to a certain set of events, such as the "reflection event off particle (II)" and the "reflection event off particle (III)" in this sketch of (the definition how to measure). If three participánts are pairwise M-W-parallel wrt. the same set of (at least several) events then let's call them "aligned to each other".

The point is: participants $\mathsf A$, $\mathsf B$ and $\mathsf P$, as described above (finding mutually coincident pings, and being pairwise M-W-parallel to each other) are not "aligned to each other". In other words, the configuration specified so far has $\mathsf A$ and $\mathsf B$ "falling one behind the other on the same radial track", while $\mathsf P$ is "moving along on the side", and possibly "rotating around $\mathsf A$'s and $\mathsf B$'s track".

Now, there may be certain additional participants identified in reference to $\mathsf A$, $\mathsf B$ and $\mathsf P$; namely:

• participant $\mathsf N$ such that any one among $\mathsf A$, $\mathsf B$, $\mathsf P$ and $\mathsf N$ finds coincident pings with respect to the three others; and likewise

• participant $\mathsf Q$, distinct and separate from $\mathsf N$, such that any one among $\mathsf A$, $\mathsf B$, $\mathsf P$ and $\mathsf Q$ finds coincident pings with respect to the three others.

Toegether, the specified configuration of the five participants $\mathsf A$, $\mathsf B$, $\mathsf N$, $\mathsf P$ and $\mathsf Q$ resembles that of five vertices of a (regular) triangular bi-pyramid (a.k.a. "(regular) triangular di-pyramid"), with $\mathsf N$ and $\mathsf Q$ corresponding to the two opposite "pyramid tips", and $\mathsf A$, $\mathsf B$ and $\mathsf P$ "at the waist".

In an actual regular triangular bi-pyramid (flat, non-rotating, in a flat region) the distance between its two opposite "pyramid tips" is of course equal to the $\sqrt{6}$-fold of the distance between any other pair of vertices.
Correspondingly it may be checked, for instance, whether

(1) $\mathsf N$ observed the completion of 2 consecutive "signal roundtrips" to and from $\mathsf Q$ before the completion of the corresponding 5 consecutive "signal roundtrips" to and from $\mathsf P$ (since $2~\sqrt{6} \lt 5$),

(2) $\mathsf N$ observed the completion of 20 consecutive "signal roundtrips" to and from $\mathsf Q$ before the completion of the corresponding 49 consecutive "signal roundtrips" to and from $\mathsf P$ (since $20~\sqrt{6} \lt 49$),

(3) $\mathsf N$ observed the completion of 9 consecutive "signal roundtrips" to and from $\mathsf Q$ after the completion of the corresponding 22 consecutive "signal roundtrips" to and from $\mathsf P$ (since $9~\sqrt{6} \gt 22$), etc.

Further, for each pair of the participants $\mathsf A$, $\mathsf B$, $\mathsf N$, $\mathsf P$ and $\mathsf Q$ it may be required (or at least be checked) whether an additional participant can be identified as "middle between" the pair under consideration; i.e. by finding coincident pings and by "alignment" as described above. For instance, participant "$\mathsf M[~\mathsf A, \mathsf B~]$" would be identified as the (unique) "middle between" $\mathsf A$ and $\mathsf B$ (throughout the entire trial) by

• $\mathsf M[~\mathsf A, \mathsf B~]$ finding for each indication coincident pings with respect to $\mathsf A$ and $\mathsf B$, and

• $\mathsf M[~\mathsf A, \mathsf B~]$, $\mathsf A$ and $\mathsf B$ being throughout aligned with respect to each other.

Comparing again to geometric relations in actual regular triangular bi-pyramid (flat, non-rotating, in a flat region) it may be checked moreover whether

(4) $\mathsf M[~\mathsf A, \mathsf P~]$ found coincident pings necessarily with respect to $\mathsf A$, $\mathsf P$,
but also with respect to $\mathsf M[~\mathsf A, \mathsf B~]$, $\mathsf M[~\mathsf B, \mathsf P~]$, $\mathsf M[~\mathsf N, \mathsf P~]$, $\mathsf M[~\mathsf P, \mathsf Q~]$, $\mathsf M[~\mathsf A, \mathsf N~]$, and $\mathsf M[~\mathsf A, \mathsf Q~]$,

(5) $\mathsf M[~\mathsf N, \mathsf Q~]$ found coincident pings with respect to $\mathsf A$, $\mathsf B$, and $\mathsf P$,

(6) $\mathsf M[~\mathsf N, \mathsf Q~]$ found coincident pings with respect to $\mathsf M[~\mathsf A, \mathsf B~]$, $\mathsf M[~\mathsf A, \mathsf P~]$, and $\mathsf M[~\mathsf B, \mathsf P~]$, and

(7) $\mathsf M[~\mathsf N, \mathsf Q~]$ found the completion of any 1 "signal roundtrip" to and from $\mathsf P$ coincident to the completion of the corresponding 2 "signal roundtrips" to and from $\mathsf M[~\mathsf A, \mathsf B~]$.

Recalling that the light-cone structure in the region under consideration is complicated, it may be argued that

• the criteria (4 ... 7) may not (all) be found satisfied exactly; and satisfied at least approximately only in the limit as $\mathsf A$, $\mathsf B$ and $\mathsf P$ are not separated from each other, and

• by quantifying the possible deviations from the criteria (1 ... 7) being satisfied, or by similar/related measurements, the region containing $\mathsf A$, $\mathsf B$ and $\mathsf P$ may be characterized, as they are "falling". An applicable quantity of particular interest for this purpose is apparently (the sign of) "Karlhede's invariant", cmp. http://arxiv.org/abs/1404.1845 .

Answer 5

Therefore I'd like to ask a related question in which pings are plainly the main point...

OK. I would refer you to Einstein talking about the speed of light varying with gravitational potential. And to Irwin Shapiro, who was involved in pinging radar signals to Venus and back, saying "the speed of a light wave depends on the strength of the gravitational potential along its path". And to the "coordinate" speed of light wherein "at the event horizon of a black hole the coordinate speed of light is zero".

Consider, as a thought-experiment, a person who is falling(1a), while taking a sequence of selfies, operating a convenient device with a "front camera" and a "display"

No problem. Let's say they're taking a selfie right now, just as they're at the event horizon. Only the coordinate speed of light is zero. So has the light moved from their face to their camera yet? No, not yet.

While taking these selfies the person under consideration is also directly reviewing the resulting photographs. Can this person notice anything "peculiar, associated with a horizon(1b)"

No, because the light hasn't got to their camera yet, and electronic signals in the camera haven't worked their way through to the screen yet, and the light from the screen hasn't got to their eye yet, because the coordinate speed of light is zero. And of course the electrochemical signals haven't moved from their eye to their brain yet. Houston, we have a problem.

before hitting a singularity(1c)?

How's that going to happen? At the event horizon the coordinate speed of light is zero, and nothing can go faster than the speed of light. Even falling observers. Yes, people talk about finite proper time, but have a read of Kevin Brown's Formation and Growth of Black Holes and note this:

"This leads us to think that, rather than slowing down as it approaches the event horizon, the clock is following a shorter and shorter path to the future time coordinates. In fact, the path gets shorter at such a rate that it actually reaches the future infinity of Schwarzschild coordinate time in finite proper time."

The infalling observer crosses the event horizon at a time that we would say is future infinity. That's the end of time. So he hasn't got there yet, and he never ever will. Nor has he noticed passing the event horizon, and nor does he notice that he doesn't notice anything any more. Just as you don't notice when you fall asleep. As for what you're usually told in popscience books like Black holes and Time Warps, well, I would urge you to take things like Interstellar and time travel with a pinch of salt. I would also urge you to read up on Oppenheimer's frozen star. And note this: in GR we say that all coordinate systems are equally valid, but when light doesn't move there is no way to measure distance and time, so there is no coordinate system to be equally valid. As for Eddington-Finkelstein coordinates, note this from Wikipedia:

"They are named for Arthur Stanley Eddington and David Finkelstein, even though neither ever wrote down these coordinates or the metric in these coordinates. Roger Penrose seems to have been the first to write down the null form but credits it (wrongly) to the above paper by Finkelstein, and, in his Adams Prize essay later that year, to Eddington and Finkelstein. Most influentially, Misner, Thorne and Wheeler, in their book Gravitation, refer to the null coordinates by that name".

These coordinates effectively sit a stopped observer in front of a stopped clock and claim that he sees the clock ticking normally "in his frame". He doesn't. He doesn't see anything. Because the coordinate speed of light is zero.