Do interaction-free measurements require a physical collapse or splitting in order to be truly interaction free?

Question

Interaction-free quantum experiments like Renninger's experiment or the Elitzur-Vaidman bomb tester are often taken to be examples of interaction-free measurements of a system. Unfortunately, such assumptions presuppose the ability to post-select in the future just to make sense. Interpretationally speaking, it is hard to see how post-selection can possibly be made without some form of physical collapse of the wave function or a preferred physical splitting of the wave function into branches. Without either a physical collapse or splitting, is it possible to gain information about a system of which we are totally ignorant about the preparation of its properties we are interested in without an actual interaction with it? Basically, does the idea of interaction-free measurements only make sense within some interpretations of quantum mechanics and not others? Is there a philosophical reading of the two-state formalism which does not presuppose a collapse or a splitting? In the two-state formalism, does the necessity of normalizing the overall probability factor to 1 entail the ontological reality of the other outcomes because we have to sum up over their probabilities to get the rescaling factor? The other outcomes where the bomb did in fact go off?

Answer 1

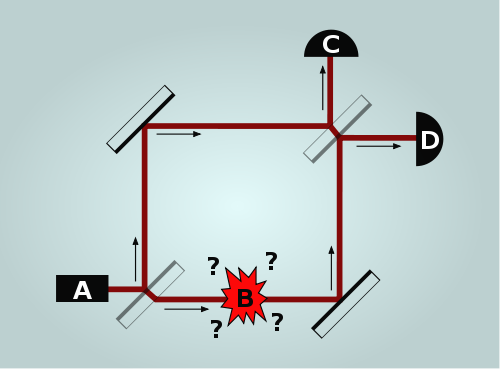
The Elitzur-Vaidman bomb tester isn't really an interaction free measurement. Analyze it using consistent histories. Suppose initially, for the three possible bomb states, we start off with the mixture diag(p, 1-p, 0) for dud, workable but unexploded, and exploded respectively. Let $P_c$ correspond to the projector of a photon detected at C. Let $P_l$ correspond to the projector the photon traveled along the lower path, and $1−P_l$ it travelled along the upper path. Consider the chain operators $C_1≡P_c P_l$ and $C_2≡P_c (1−P_l)$.

Note that $Tr[C_1 ρ C_2^\dagger]=p/4≠0$. The consistency conditions are not satisfied. In a realm where we know the photon was detected at C, we can't say whether or not it took the lower path. It's not really interaction free after all. That presupposes the photon didn't take the lower path.