7
$\begingroup$

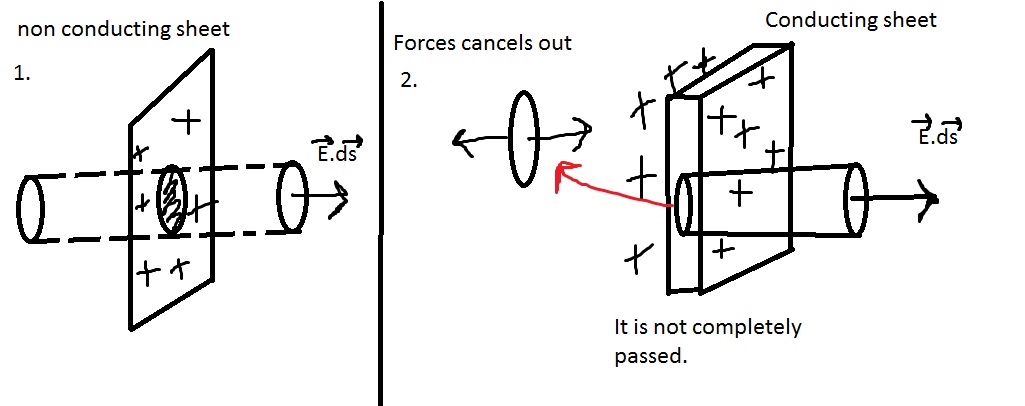
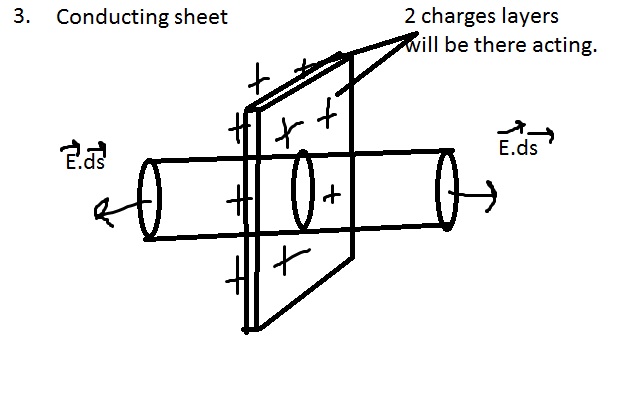
I have this grave confusion that I have been having since a while. When we calculate the electric field due to an infinite plane sheet of charge then the answer comes out to be $σ/2ε$. In this case we take a cylindrical Gaussian surface which extends to both the sides and that is why we have halved the electric field to get the answer. Now when I try to get the same for a charged conducting surface then why does the value double itself? The diagram in my book shows a rectangular slab kind of a conductor in which one flat surface of the cylinder (the Gaussian surface) is embedded inside the conductor and the other is outside. According to the diagram,then , there should be a back side of the conductor. So if I construct a Gaussian surface such that it extends out from both the sides ,then I should get the same answer as that for a charged sheet! Why do we not do this in this case?

All I know is that for a charged conductor the field inside is 0 and the charge resides on the surface.

Also considering any one surface of the conductor ,the field lines move outward. Do the field lines move inside the conductor due to the charges present on the surface?That is -if I have a slab like conductor with a point S inside it, then is there any field due to the surface charge at that point.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ Wouldn't the charge enclosed also double though? $\endgroup$
    – mr blick
    Commented May 12, 2015 at 18:48
  • $\begingroup$ +1 thanks a lot!I had failed to notice this before !:( could you also answer my last question ? $\endgroup$
    – Karan Singh
    Commented May 12, 2015 at 18:52
  • $\begingroup$ Np. Also, no there is no field. This is just a property of conductors. In the case of a slab, the charge on the opposite surface creates an electric field that exactly cancels the electric field produced by the other side $\endgroup$
    – mr blick
    Commented May 12, 2015 at 18:56
  • $\begingroup$ Does it mean that they will create a field at that point but due to the opposite nature they add up to 0? $\endgroup$
    – Karan Singh
    Commented May 12, 2015 at 18:58
  • $\begingroup$ More on capacitors and factors of 2: physics.stackexchange.com/q/110480/2451 $\endgroup$
    – Qmechanic ♦
    Commented May 12, 2015 at 19:03

2 Answers 2

Reset to default
7
$\begingroup$

The basic concept is to see the charge distribution among them. From these example I hope your doubt will be quite clear

First let us take one non-conducting sheet then,

$$E\cdot \mathrm ds + E\cdot \mathrm ds + 0 = \frac{1}{\varepsilon_0}(\sigma\cdot \mathrm ds) $$

For curved surface it will be zero and let us take sigma distribution on sheet. See Figure 1.

enter image description here

Now for conducting sheet (Case 1)

Here Charges will distribute automatically. So now Let us take one gaussian cylinder passing through that sheet but not completely like till half of it sheet as shown in diagram. NOTE: It is not completely passed from the other end.

$$E\cdot \mathrm ds = \frac{1}{\varepsilon_0}(\sigma\cdot \mathrm ds)$$

Reason : Because at the middle of the sheet there is flat surface of our gaussian cylinder and at that surface there will be 2 forces acting on it. First one acting left hand side and other on right hand side due to charge distribution at both of the surfaces equally. See figure 2.

Again for conducting sheet (Case 2)

Now again let us take gaussian cylinder and this time it will pass from other end completely. Now just before going to question let us take one example. Imagine that if we put one cylinder inside sheet then we will see that 2 layers sheet will come out. First from front of the sheet and another from the back side. Why I said this because since charge is equally distributed on that conducting sheet so charges will be in front and back side so that's why if we take one gaussian cylinder then 2 charge layer will act at that time means there will be change in the above equation which we are writing.

enter image description here

So the equation would be like

$$2E\cdot \mathrm ds = \frac{1}{\varepsilon_0} (2\sigma \cdot \mathrm ds)$$

Which will make our equation

$$E=\frac{\sigma}{\varepsilon_0}$$

Do the field lines move inside the conductor due to the charges present on the surface

No, electric field lines never move inside the conductor. That's why electric field inside the conductor is zero as conductors are equipotential.

Improve this answer
$\endgroup$
0
$\begingroup$

"So if I construct a gaussian surface such that it extends out from both the sides ,then I should get the same answer as that for a charged sheet! Why do we not do this in this case ?" If you do that you have to realize that the other side has the same amount of charge as the first, so the field is doubled.

When you consider a differential element of charge on the surface $\mathrm dq=\sigma\,\mathrm ds$ then, as you noted, this element alone also generates field lines going inside the conductor. However, we know that inside the conductor there are no lines (field=0). This is because the charge distributed over the remaining conductor's suface cancels the filed created by the charge in the surface element $ds$.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.