Why do the electric field cross the metal plate (with 2 surfaces)?

Question

First let me tell you what am I talking about, an infinite (just for questions) plane sheet or non-conducting sheet is that have only one surface and we say that the electric field due to it is $$\frac{\text{surface charge density}}{2\varepsilon}.$$ But a conducting plate has 2 similar surfaces and I say that we have a positive charge density equal to $\sigma$ on both surfaces so I have found in many books that they superpositioned the electric field due to both the sides of the metal plate, like:

but, the electric field due to one surface should not have an impact on the other side as there is metal inside it and electric field lines do not cross the conductor.

Answer 1

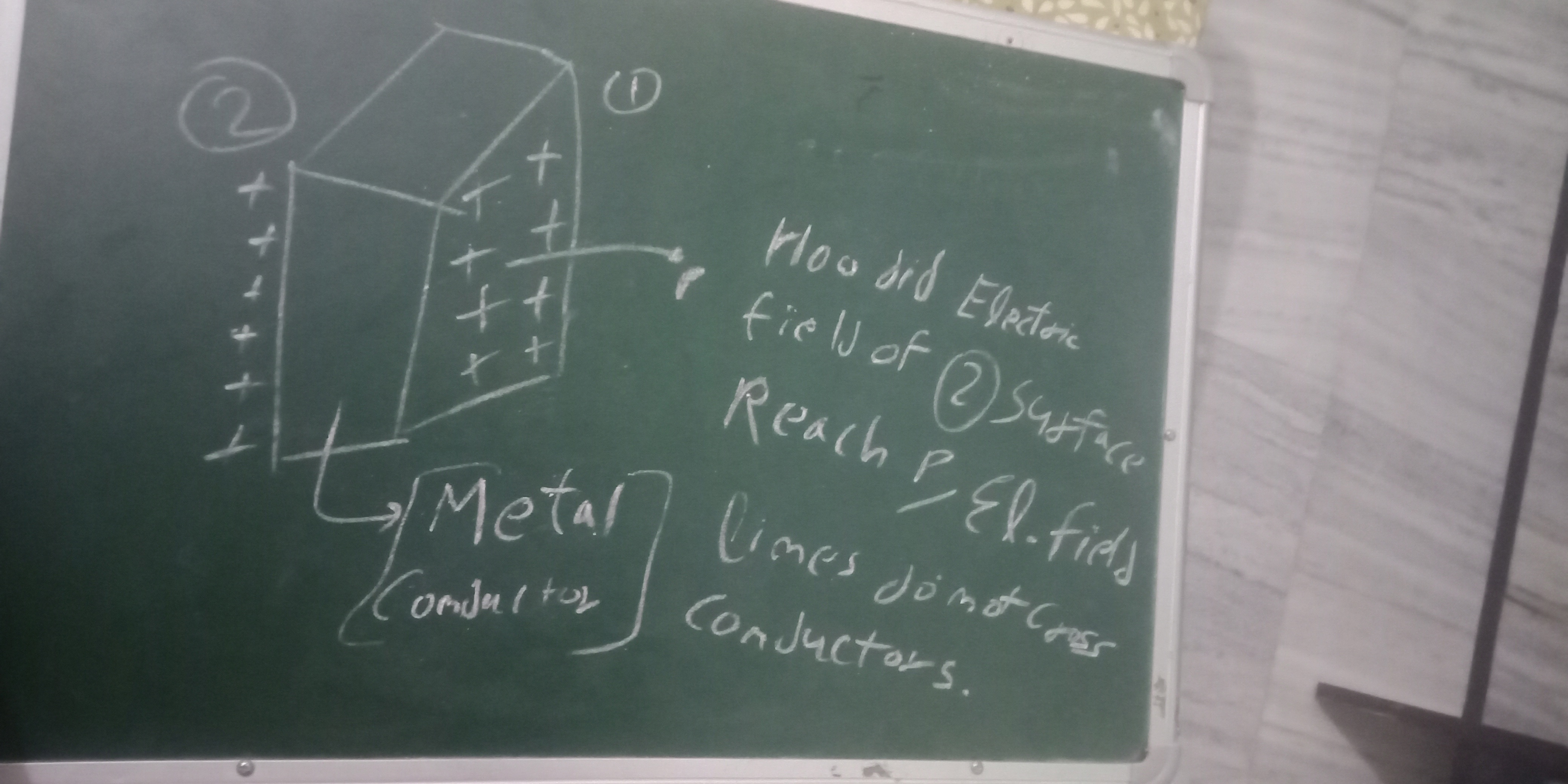
I believe you are correct in saying we are not able to superimpose $\vec E_1$ and $\vec E_2$. The expression $\frac{\sigma}{\epsilon_0}$ can be derived from Gauss' Law.

Gauss' Law

States that the electric flux $\Phi_E$ through any closed surface is:

$$\Phi_E = \frac{q}{\epsilon_0} ,$$

where $q$ is the algebraic sum of any enclosed charges. A closed surface can be thought of as any surface completely enclosing a volume, such as a sphere or prism.

Electric Flux

A quantity roughly analogous to the number of field lines passing through a surface. For a uniform electric field, the electric flux through a flat surface is given by the dot product between the electric field $\vec E$ and surface normal $\vec A$:

$$\Phi_E = \vec E\cdot \vec A ,$$

where $\vec A$ is a vector perpendicular to the surface with magnitude equal to the surface area $A$.

Derivation

Consider a closed cylindrical surface with one face at the prism centre and another outside of the prism.

Let face 1 be the circular face inside the prism, face 2 be the rounded face and face 3 be the circular face outside the prism. We now consider the electric flux through each of the 3 faces:

• In static situations, the electric field inside any conductor must be 0 or charges would still be moving. This implies the flux $\Phi_1$ through face 1 is 0.

Assuming the prism is sufficiently large, electric field lines may be assumed parallel and normal to the prism surface, meaning:

• Electric field lines are also parallel to face 2, making them perpendicular to the surface normal. The flux $\Phi_2$ through face 2 is thus also 0.

• Electric field lines are perpendicular to face 3, making them parallel to the surface normal. The flux $\Phi_3$ through face 3 is thus equal to $EA$, where $A$ is the circular face area.

Finally, in static situations, all excess charge on a conductor lies on the surface. Thus, the charge enclosed within our cylinder is just $\sigma A$. By Gauss' Law, we have:

$$\frac{\sigma A}{\epsilon_0} = EA ,$$

so dividing through by $A$ gives us:

$$E = \frac{\sigma}{\epsilon_0}$$

as required.

I hope this makes sense! I have tried to draw a diagram, but as this is my first time responding to a post, I haven't yet worked out how to do so. This post Electric field due to a charged conductor may help as well.