I did a bit of reading about this, and it turns out that conformal blocks are actually quite relevant to my research! So I figured it was worth the time to investigate in some more detail. I've never studied conformal field theory formally, but I hope I'm not writing anything outright wrong here. (I lost my first draft and had to reconstruct it, which is why it's taken so long)
In conformal field theory, it's common to represent coordinates on a two-dimensional space by using complex numbers, so $\vec{r} = (x,y)$ becomes $\rho = x + iy$. In this notation, the theory is invariant under the action of a Möbius transformation (a.k.a. conformal transformation),
$$\rho \to \frac{a\rho + b}{c\rho + d}$$
in which $a$, $b$, $c$, and $d$ are complex constants that satisfy $ad - bc \neq 0$. The transformation has three complex degrees of freedom - in other words, if you specify three initial points and three final points on the complex plane, there is a unique Möbius transformation that maps those three initial points to the three final points.
So any function of four coordinates on the plane, for example a four-point correlation function of quantum fields,
$$G_4 = \langle \phi_1(\rho_1,\rho_1^*) \phi_2(\rho_2,\rho_2^*) \phi_3(\rho_3,\rho_3^*) \phi_4(\rho_4,\rho_4^*) \rangle$$
has only one real degree of freedom, after you factor out the gauge freedoms corresponding to the Möbius transformation. In other words, you can map any three of those coordinates on to three fixed reference points (for example $0$, $1$, and $\infty$), and you're left with a function of only one variable, something like
$$x = \frac{(\rho_4 - \rho_2)(\rho_3 - \rho_1)}{(\rho_4 - \rho_1)(\rho_3 - \rho_2)}$$
This opens the door to write $G_4$ as a simple function of this one ratio (at least, simpler than a function of four independent coordinates).
The particular part of CFT in which conformal blocks are applied (as far as I can tell; I'm starting to get a little out of my depth here) has to do with Virasoro algebras. Specifically, the way the individual fields $\phi_i$ transform under a conformal transformation is described by the group defined by the Virasoro algebra. The four-point function $G_4$ can be written as a sum of contributions from different representations of the group,
$$G_4(\rho_1,\rho_2,\rho_3,\rho_4) = \sum_l G_l f(D_l, d_i, C, x) f(D_l, d_i, C, x^*)$$
Here $l$ indexes the different representations; $C$ is a constant (the "central charge" of the Virasoro algebra); and $d_i$ and $D_l$ are anomalous dimensions of the external fields and the internal field respectively. The function $f$ is called a conformal block.
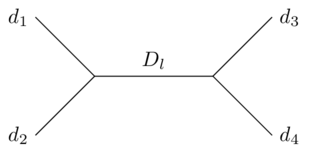
$f$ is useful because it can be calculated (in principle or in practice, I'm not sure which) using only information about a single representation of the Virasoro group. It can be expressed as a series in $x$ of a known form, the coefficients of which depend on the structure of the group.
Further Reading
- Belavin A. Infinite conformal symmetry in two-dimensional quantum field theory. Nuclear Physics B. 1984;241(2):333-380. Available at: https://doi.org/10.1016/0550-3213(84)90052-X.
- Zamolodchikov AB. Conformal symmetry in two dimensions: an explicit recurrence formula for the conformal partial wave amplitude. Communications in Mathematical Physics (1965-1997). 1984;96(3):419-422. Available at: https://doi.org/10.1007/BF01214585.
- Zamolodchikov AB. Conformal symmetry in two-dimensional space: Recursion representation of conformal block. Theoretical and Mathematical Physics. 1987;73(1):1088-1093. Available at: https://doi.org/10.1007/BF01022967.
and of course DiFrancesco et al's book.

