2
$\begingroup$

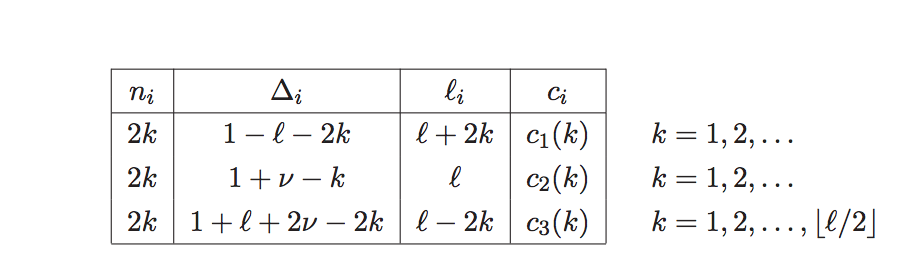
I'm trying to calculating the four point function for the 3D Ising model. To do so I need to calculate the 3D Conformal Blocks . I found a paper which has a recursive relation for calculating the Conformal Blocks: equation (3.10). The authors are Filip Kos, David Poland and David Simmons-Duffinb, and the title is Bootstrapping the O(N) vector models. $$h_{\Delta,\ell}(r,\eta)=h_\ell^{(\infty)}(r,\eta)+\sum_i\frac{c_i r^{n_i}}{\Delta-\Delta_i}h_{\Delta_i+n_i,\ell_i}(r,\eta)\tag{3.10}$$

However, I am unsure how to actually implement it. I want to compute (3.10) up to $r^{12}$. I imagine my expression is going to look like a sum of the $h^{(\infty)}$ times coefficients with powers of $r$. However I'm not so sure that is the correct interpretation of this recursion relation. If anyone can point me in the right direction that would be much appreciated.Also I do not know how many times I need to plug the right hand side into the left hand side. If I want to order 12 I imagine I do it 12 times.

enter image description here

As an example of what I am doing. I'll sum up to $k=1$ and pretend I'm looking for order up to $r^2$ only

$$h(V,L)=\sum _{k=1}^{\frac{L}{2}} \frac{c(k)_3 r^{2 k} h(L+2,L-2 k)}{2 k-L+V-2}+\frac{c(1)_1 r^2 h(1-L,L+2)}{L+V+1}+\frac{c(1)_2 r^2 h\left(\frac{5}{2},L\right)}{V-\frac{1}{2}}+H(\infty ,L)$$

after iterating this once so I only get $r^2$ terms I get

$h(1.4,0)=1.11111 c(1)_2 r^2 \left(\frac{2}{7} c(1)_1 r^2 h(1,2)+\frac{1}{2} c(1)_2 r^2 h\left(\frac{5}{2},0\right)+H(\infty ,0)\right)+0.416667 c(1)_1 r^2 \left(\frac{1}{4} c(1)_1 r^2 h(-1,4)+2 c(1)_2 r^2 h\left(\frac{5}{2},2\right)-c(1)_3 r^2 h(4,0)+H(\infty ,2)\right)+H(\infty ,0)$

and now expanding up to $r^2$ yields

$h(1.4,0)=r^2 \left(0.416667 c(1)_1 H(\infty ,2)+1.11111 c(1)_2 H(\infty ,0)\right)+H(\infty ,0)$

all of the $h$'s from the rhs are gone and I have something in terms of $H(∞,2)$ which I know how to evaluate. Is this the correct approach, I'm asking because even though I'm doing it this way I'm getting utter nonsense for my answer. To get the Conformal Block I then multiply this by $r^V$ which gives $.08$ instead of $.6707$ I should be getting.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ Just do it to the order $r^0$, then $r^1$, then $r^2$, etc, and at some point enlightement will come. $\endgroup$
    – Sylvain Ribault
    Commented Jul 7, 2018 at 14:14
  • $\begingroup$ I have been trying to do that. Am I right to assume there is a another sum that is being suppressed in this expression that is summing the k's from 1 to infinity, while the first sum that is present only goes up to 3, for the 3 n's in the table? $\endgroup$
    – Daniel Berkowitz
    Commented Jul 7, 2018 at 17:44
  • $\begingroup$ @DanielBerkowitz, $i=(k,t)$ where $t=1,2,3$ is the type of the pole. The point is that to any given order in $r$ you need to keep only finitely many terms in the sum, and $h$ which appear in these terms you need to know to a strictly lower order. Just track powers of $r$ carefully. $\endgroup$
    – Peter Kravchuk
    Commented Jul 8, 2018 at 2:49
  • $\begingroup$ @PeterKravchuk this is the only formula I have for h, are you saying I need to find some other formula for h so that when I iterate this expression I can plug in some known value of h? $\endgroup$
    – Daniel Berkowitz
    Commented Jul 8, 2018 at 18:15
  • $\begingroup$ @SylvainRibault so lets say for r^0 I have h^infinity, now if I were iterate this to r^2 I would (h^Infinity) + (constant)*(r^2)*(h^infinity) and the rest are higher order in r and can be dropped assuming I'm only interested in order r^2 right? $\endgroup$
    – Daniel Berkowitz
    Commented Jul 8, 2018 at 19:14

1 Answer 1

Reset to default
2
$\begingroup$

To the order $r^2$, the block is given by the contributions of three poles with $k=1$, $$ h_{\Delta,\ell} = h^{(\infty)}_\ell + \frac{c_1(1)}{\Delta+\ell+1} r^2 h^{(\infty)}_{\ell+2} +\frac{c_2(1)}{\Delta-\nu} r^2 h^{(\infty)}_{\ell} + \frac{c_3(1)}{\Delta-\ell-2\nu+1} r^2 h^{(\infty)}_{\ell-2} + O(r^4) $$ To the order $r^4$, let us assume for simplicity $c_2=c_3=0$, we find $$ h_{\Delta,\ell} = h^{(\infty)}_\ell + \frac{c_1(1,\ell)}{\Delta+\ell+1} r^2 h^{(\infty)}_{\ell+2} + \left(\frac{c_1(1,\ell)}{\Delta+\ell+1}\frac{c_1(1,\ell+2)}{\Delta+\ell+3} + \frac{c_1(2,\ell)}{\Delta+\ell+3}\right) r^4 h^{(\infty)}_{\ell+4} + O(r^6,c_2,c_3) $$ To do such calculations you need good and consistent notations. (The original paper does not help, for example $c_1(k)$ actually also depends on $\ell$, see eq. (3.13).)

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.