I am doing a video for 8th grade on the subject. I'm showing the experiment of swinging a bucket of water over your head. Is there a huge difference between centrifugal and centripetal forces? Would it be wrong to say the two forces are practically the same thing?
$\begingroup$
$\endgroup$
15
-
1$\begingroup$ Centrifugal force is observed by the water or bucket which is in a noninertial frame ,what we observe is centripetal force. $\endgroup$– PaulCommented Apr 30, 2015 at 1:43
-
1$\begingroup$ I read recently in a book that centrifugal force can be addressed as a consequence of Newton's third law, i.e: Centrifugal force can be thought as the equal and opposite reaction to Centripetal force. $\endgroup$– RogUECommented Apr 30, 2015 at 2:42
-
3$\begingroup$ It's the difference between swinging the bucket and being the water. $\endgroup$– user121330Commented May 19, 2015 at 15:44
-
1$\begingroup$ Though the project is undoubtedly already in the past, wouldn't it be helpful to note that (at least in a middle school context) the real centripetal force and the not real centrifugal "force" are not really the same thing for a good and I think more glaring reason: because they "oppose" each other??? - they "act" in different and opposite directions - they are not in almost any way the "same thing" ---- or am I missing something? $\endgroup$– Code JockeyCommented Jan 21, 2016 at 15:34
-
1$\begingroup$ @Asher -- Please fix Wikipedia, then - it's mean to allow those innocent souls who blindly take it for gospel to be misinformed. $\endgroup$– Code JockeyCommented Jan 21, 2016 at 22:06
|
Show 10 more comments
9 Answers
$\begingroup$
$\endgroup$
2
I'm not fond of calling centrifugal force an "imaginary" force. I prefer to explain it in terms of reference frames:
In an inertial reference frame, Netwon's laws are sufficient to model (non-relativistic) motion.
In a non-inertial reference frame, additional forces (like centrifugal, Coriolis, etc.) must be added to the model.
What makes centrifugal different than centripetal is that centripetal force is due to interaction with another object (gravity for orbiting objects, tension for spinning a ball on a string, friction for an object on a spinning merry-go-round, etc), while centrifugal force must be added to the model "out of nowhere", just because you are in a rotating reference frame.
To explain this to an 8th grade class, I would give an example of two physicists: 1 standing on the ground, one spinning on a merry-go-round. They both perform measurements to detect the forces acting on the spinning physicist. The physicist on the ground only detects centripetal force, and notes that this causes the other physicist to travel in a circle. The rotating physicist detects centripetal force and an equal but opposite centrifugal force, resulting in him remaining at rest in his frame.
-
16$\begingroup$ +1 for disparaging "imaginary". Telling a casual observer that something they can feel is "imaginary" or "fictional" is not likely to encourage them toward learning more about science. Telling 'em that the same situation can be analyzed from more than one point of view (i.e., "frame of reference"), and that some models yield deeper insights than others seems more likely to spark their interest. $\endgroup$ Commented Apr 30, 2015 at 16:57
-
$\begingroup$ I'd say this could be used for her project instead of the bucket of water... I like your take on it - seems like you're stating that though it's not "real" for everyone, it certainly feels real and can be almost thought of as real for others $\endgroup$ Commented Jan 21, 2016 at 15:42
$\begingroup$
$\endgroup$
3
There is definitely a huge difference. Centripetal force is a real force that causes objects to move in a circular path or curved path that points to the center of the circle or curvature respectively. Centrifugal force is not a real force. It is an inertial force established so that Newtonian laws are valid when observing motion in an accelerated frame of reference.
-
$\begingroup$ I only have a 3-5 minute video. For simplicity, I was wondering if I should just call them the same and mention briefly a difference. $\endgroup$– NikkieCommented Apr 30, 2015 at 1:50
-
5$\begingroup$ To be frank, I would not prefer calling them the same. Once again I would like to highlight that centripetal force is a real force- it's the force that causes curved motion. In contrast, centrifugal force is not real. It is, as mentioned by @José Jorge Medina Serna, an imaginary force. When observing the bucket in the rotating frame, the bucket appears to be in linear motion, with no alterations of its direction. Therefore, an imaginary force must be incorporated so that the net force on the direction perpendicular to the tangential direction is zero. $\endgroup$ Commented Apr 30, 2015 at 2:05
-
2$\begingroup$ @Nikkie Why come to this site looking for advice and then not take it. These forces, (or force plus "force") are not the same, and any implication that they are to students causes difficulties later. $\endgroup$– ProfRobCommented Apr 30, 2015 at 6:24
$\begingroup$
$\endgroup$
4
Centripetal force is the force that an object feels when is in a circular motion.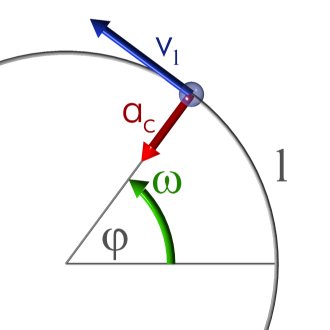 
In this picture, $a_c$ is the centripetal force, $v$ tangential velocity, and $\omega$ angular velocity.
Now, centrifugal force is the imaginary force that appears when the reference frame is in rotation.
answered Apr 30, 2015 at 1:43
-
1$\begingroup$ I would bet that in that image $a_c$ was meant to indicate the centripetal acceleration. The centripetal force is of course a positive multiple (by the mass) of the centripetal acceleration, so qualitatively the arrow could also indicate centripetal force. $\endgroup$ Commented Apr 30, 2015 at 6:39
-
$\begingroup$ When you say "imaginary force that appears" you mean that the force exists to justify the fact the that body isn't moving towards the center of the circle? $\endgroup$– Nadav S.Commented Apr 30, 2015 at 7:05
-
1$\begingroup$ @NadavS Not quite. It appears when your frame of reference is rotating. It would then be needed to explain the tendency of objects to move away from the center of the circle. $\endgroup$– TaemyrCommented Apr 30, 2015 at 7:32
-
2$\begingroup$ It's probably better to say centripetal force is the force that makes the object go in a circular motion. $\endgroup$ Commented Apr 30, 2015 at 8:20
$\begingroup$
$\endgroup$
1
For a 3-minute video I think I would avoid both concepts.
Centrifugal force can be a confusing concept for several reasons (not least of which that the mere mention of the term tends to attract pedantic know-it-alls who have decided it's their task in life to derail every discussion with loud patronizing explanations of how it doesn't exist at all). Since you don't have any particular reason to look at things from a rotating frame here -- it's not appreciably easier for this problem because in your rotating frame you have to take into account that the direction of gravity changes, and the bucket isn't even following a uniform circular motion; and there isn't any observer in the bucket whose experiences we need to explain -- there's no reason to step into the quagmire of centrifugal force.
Centripetal force would also seem to overcomplicate the situation here. Again, since the circular motion is not uniform (it's slower at the top due to gravity) you'd need to get into instantaneous angular velocities and other hairy stuff to justify its use properly. Unless you need to calculate the force with which the bucket pulls you upwards at the top of the swing, that's not really worth the trouble either.
That leaves the question of why the water doesn't fall out of the bucket, of course. Here's what I would say:
The water wants to fall down, but not at any cost. In particular it doesn't want to fall down faster than with an acceleration of 9.82 m/s². When you're pulling on the bucket in order to keep it going in a circle, you're forcing the water to move downwards faster than it would if it was falling by itself. It resists that motion (which you can feel in your arm), and doesn't move down any quicker than the bucket is forcing it to.
answered Apr 30, 2015 at 14:00
-
$\begingroup$ IMO @mbeckish's answer (carousel with two different reference frames) would probably be better suited for a 3-5 minute video, showing from each perspective for ~30 seconds, analysis from each perspective and overall each for about a minute, complete with after-effects arrows and traces drawn over the video. It would keep gravity changes negligible at least... if she wants the bucket of water, she could put that on a carousel... :-P $\endgroup$ Commented Jan 21, 2016 at 15:48
$\begingroup$
$\endgroup$
Centripetal and centrifugal forces are only equal for an object being held stably in a rotating system.
A good analogy is the difference between 1) the force of gravity and 2) the supporting force of the ground pushing up on you. Centrifugal forces and gravity aren't quite proper forces since they are really more like fixed accelerations; to represent them as a forces we need to multiply by the mass of the object.
The force of the ground pushing up on you, which counteracts gravity, is a very real force having to do with your feet's surface molecules straining against the ground's surface molecules. Likewise the centripetal forces for water in a spinning bucket, are real contact forces that support the water inside the bucket.
But for an object falling, there is no supporting force yet the gravitational force continues pulling downward, according to an observer standing on the ground. Likewise for a drop of water that falls out of the spinning bucket, there is a centrifugal force that pushes the drop away, according to an observer rotating with the bucket.
$\begingroup$
$\endgroup$
A totally fictitious example: Consider a distant object that is at rest in an inertial frame. In that frame you rotate at some uniform angular velocity. In your non-inertial rest frame, the object is rotating at the opposite angular velocity, it thus has a centripetal acceleration toward you. How then do we account for the fictitious centripetal force acting on the object? There is a centrifugal force due to using a non-inertial frame, but this force acts in the opposite direction, it is directed radially outward. However, there is another fictitious force acting on the object, the Coriolis force which has twice the magnitude of the centrifugal force and this is directed radially inwards (in this particular case). So, in this case, the fictitious centripetal force is the sum of the centrifugal and the Coriolis force.
answered May 27, 2015 at 20:13
$\begingroup$
$\endgroup$
It is wrong to say they are the same. I suspect for a short video that it would be better to choose one which is most relevant for your purpose and not mention the other.
Centripetal force is any force pulling inwards on an object to maintain its motion in a circle - in your case the force of your arm pulling on the bucket as it swings.
Centrifugal force has two possible meanings. It can be
- the Newton III counterpart to a centripetal force, or
- A fictional force that exits in a rotating reference frame, along with coriolis force.
In your case 1. is the force of the bucket pulling on your arm. I would only introduce 2. in a video if you film or animate a sequence with the camera rotating to match the movement of the bucket. In that sequence the bucket would be stationary and centrifugal force would pull it away from you.
$\begingroup$
$\endgroup$
Centrifugal and centripetal forces on a bucket, or weight, swinging in a horizontal circle are easier to explain than a bucket swinging in a vertical circle because gravity doesn’t complicate things in that case. According to Newton’s second law a centripetal force has to be exerted in order to keep the weight moving in a circle, i.e., accelerating toward the center of the circle. This is because without this force the weight would fly off in a straight line, according to Newton’s first law. According to Newton’s third law the weight exerts a force in reaction to the centripetal force. This is the centrifugal force.
$\begingroup$
$\endgroup$
2
Here's how you might explain the difference between the two forces to your class:
When you're whirling a bucket of water, centripetal force is carried by your arm, the rope, and the bucket. It points inward toward your shoulder, which is the center of the circle formed by the whirling bucket.
Centrifugal force is caused by the inertia of the water in the bucket. Inertia is the water's momentum to continue in a straight line at a right angle to your arm and the rope, tangential to the circle formed by the whirling bucket. Centrifugal force is not really a force - it's the apparent force that draws the water in the bucket away from your shoulder.
Wikipedia has a more detailed analysis of centrifugal force: http://en.wikipedia.org/wiki/Centrifugal_force
-
$\begingroup$ The tendency of water (or any object) to keep going in a straight line is simply inertia. $\endgroup$– bdslCommented Apr 30, 2015 at 11:59
-
$\begingroup$ bds!: Centrifugal force is an apparent force caused by the inertia of the water in the bucket. Thanks for pointing out my error. I've edited the answer. $\endgroup$– ErnieCommented Apr 30, 2015 at 12:51