There appears to be a lot of evidence that gas flows outward in Galaxies. I've been trying to parse through the available data and am unable to get a clear answer to this question: is there any evidence for an average flow of stellar material in our galaxy? That is, do we know if stars are falling in, falling out, in a perfectly stable orbit (or do we just not have enough data yet)?
-
$\begingroup$ @RobJeffries - If I'm parsing the articles correctly, there appears to be considerable evidence for 'bulk' motion of gas, meaning large populations of clouds appear to be moving radially outward from the center of the Milky Way. Since stars form from gasses, I would intuitively think that the stars would carry this momentum with them. I'm wondering if we've got any data, one way or another, on the average motion of stars in the disk: falling in, falling out, stable orbit or just don't know yet. $\endgroup$– user32023Commented Mar 4, 2015 at 13:24
2 Answers
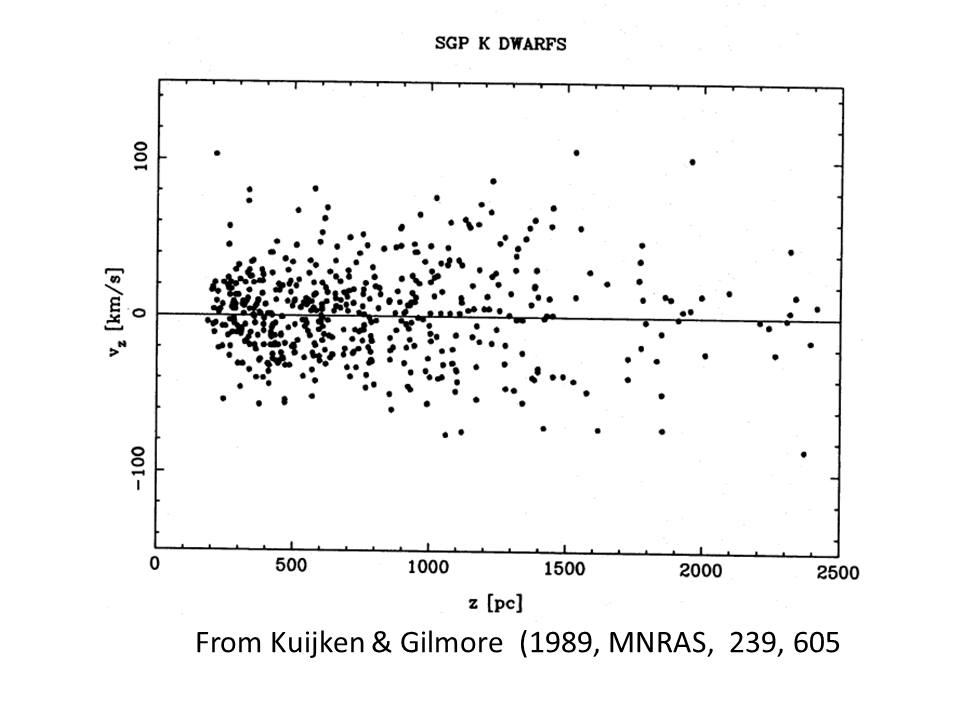
There has been lots of work done that studies the dynamics of stars with respect to Galactic disk and Galactic centre. Possibly the most celebrated is the work of Kuijken & Gilmore (1989) who studies large samples of K-stars towards the south Galactic pole. The aim of their experiment was to use the distribution of $z$ below the Galactic plane and $v(z)$, the velocity distribution, to constrain the mass contained in the Galactic disk. A side-benefit was of course that on the way they needed to look at the dependence of $v(z)$ on $z$, which I reproduce below.
This plot shows that there is no trend in the velocity towards or away from the plane with height. i.e. For this group of stars, and out to about 2000pc, there is no net expansion or contraction of the general stellar population with respect to the Galactic plane.
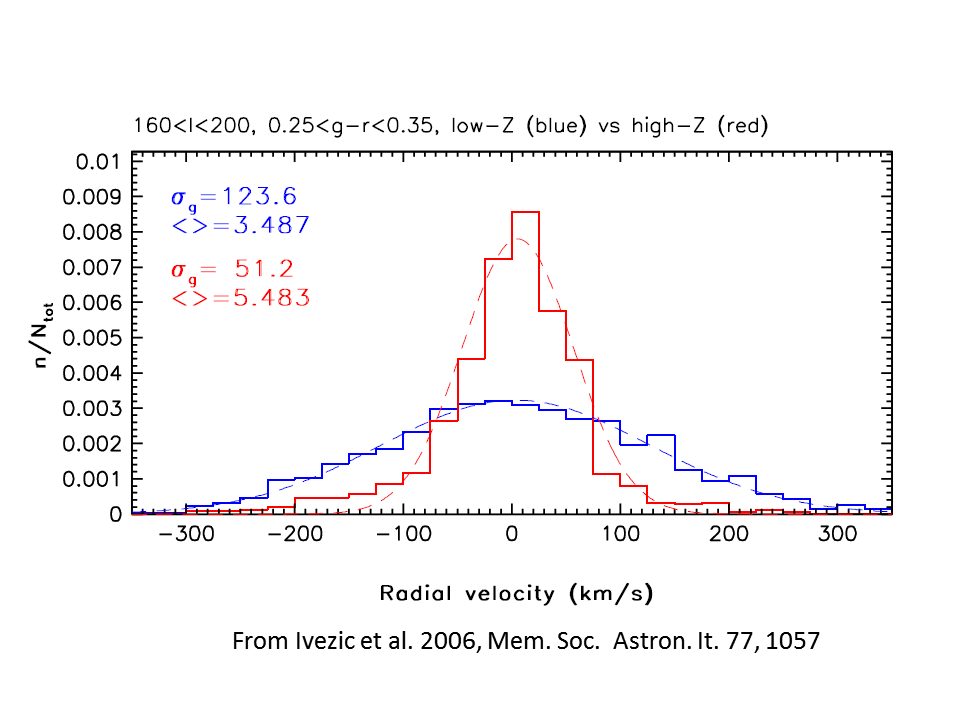
In the radial direction there are also extensive radial velocity surveys. For example the SDSS survey looked at stars all over Galactic space. I managed to find a paper that showed the radial velocity distribution towards the Galactic anti-center from Ivezic et a. (2006). These stars were observed out to distances of a few kpc from the Sun. The blue and red curves are for stars of low and high metallicity. The means of the distributions are close to zero - i.e. no net motion, but the low-metallicity (old) stars show a higher dispersion.
Most recently the LAMOST survey has been looking in the Galactic anti-center direction. The results can be found here. Between distances of 8 and 11.5 kpc from the Galactic center the mean velocities of A, F and G dwarfs show no systematic mean outflow with respect to the Sun, although they do show structure at the level of $\pm 30$ km/s associated with spiral arms.
Note that these velocities are heliocentric (i.e. with respect to the Sun). The Sun is known (see for example Schonrich et al. 2009) to move at approximately 10 km/s towards the Galactic centre compared with an average star in almost-circular motion at the same Galactocentric radius as the Sun (see here for some details and here for the solar motion with respect to the LSR; that the Sun is nearly radially at rest with respect to the Galactic centre is supported by the average radial velocities of maser sources [$-22 \pm 28$ km/s] orbiting the Galactic centre black hole - Reid et al. 2007 ). Therefore a heliocentric radial velocity shift of zero would indicate a small drift of perhaps $\sim 10$ km/s towards the Galactic centre (although note the velocity dispersions are broad and there must be at least a few km/s error).
Any notion that there is a net general drift inwards or outwards has more problems to solve than whether there is any kinematic evidence for it (there isn't, but only at the $\sim 10$ km/s level). A drift of only a few km/s (= a few kpc per billion years) outwards, which is in any case tiny compared to the orbital speed, could take a star from the bulge well out into the halo in a fraction of its, and the Galaxy's, lifetime. Thus, when we look radially outwards in the disk we should see predominantly old stars and any star interior to the solar orbit would have to be very young ($<2-3$ billion years old).
The only significant age trend is that the bulge stars are much older than an average disk star; there is little evidence for any trend in age within the disk itself. Star formation occurs at all radii in the disk. So one would have to further hypothesise that the drift began very recently. Why? What force could do that? Not the gas pressure (which is what drives any radial gas expansion): a typical ISM gas pressure is $10^{-13}$ Nm$^{-2}$ and exerts a force of only 1.5 kN on a entire main sequence star.
-
$\begingroup$ This is much closer to what I'm looking for, but, as you guessed, for the radial direction of the main disk. Here's the exact issue: our assumptions about Dark Matter are based on the observation of a rotational velocity and an assumption about a stable orbit (Kepler's laws only apply to stable orbits). So somewhere there must be a definitive study showing that there's no radial component (either inward or outward) in order to support the current calculations for the Dark Matter mass of our galaxy. I'm wondering where that data might be. $\endgroup$– user32023Commented Mar 4, 2015 at 21:52
-
$\begingroup$ "Radial direction of the disk"? Galactic outflows are perpendicular to the disk. I am no great expert, but I doubt that any serious work on dark matter relies on Kepler's laws at all, as it is not valid for a distribution of gravitating matter. What size of radial motions were you thinking of? We know indeed that the motion of gas and stars in the disk is mostly circular. $\endgroup$– ProfRobCommented Mar 4, 2015 at 22:08
-
$\begingroup$ Radial, meaning in the direction of the radius of the main disk. I'm not an expert either but I know that the general logic goes like this: the stars in the disk should obey Kepler's laws, however, they're traveling much faster than predictions based on the visible mass of the galaxy. If the stars are falling in or falling out, then we can't use the simple relation:$$ f(v) = \sqrt{GM/r^2}. $$when predicting the rotational velocity of a star at a given radius, r. Every description I've seen of the missing mass issue comes with a diagram of the Kepler prediction to highlight the missing mass. $\endgroup$– user32023Commented Mar 4, 2015 at 22:23
-
1$\begingroup$ @DonaldRoyAirey I have answered several similar queries in the last few weeks. Arguments like this are presented to simplify the discussion (usually for the layperson). A Keplerian orbit has all the mass concentrated at the centre so cannot be applied unless this is the case (or for a spherically symmetric mass distribution). It is approximately true for the case of the Galaxy if mass were to follow light. Real work uses real potentials and if not it should be criticised. [And it's $v \propto (M/r)^{1/2}$.] $\endgroup$– ProfRobCommented Mar 4, 2015 at 23:56
-
$\begingroup$ @DonaldRoyAirey I also disagree even that most explanations come with a diagram with the Kepler prediction superimposed. I just googled "rotation curve of Milky way" and followed the first image that came up on milkyway.cs.rpi.edu/milkyway/science.php Even this layperson's description correctly explains that we do not expect a strictly Keplerian curve. $\endgroup$– ProfRobCommented Mar 5, 2015 at 0:02
Due to their large inertial masses, the stars stay, but it seems there is actually evidence for gaseous outflows from the Milky Way.
See e.g. Carretti et al. (2013), and Fox et al. (2015).
Here and here are some popular versions of the latter.
At higher redshift, galactic outflows of gas are quite normal. But stars rarely leave their host galaxies except in cases where two galaxies collide, which can cause parts of the galaxies to be slung out. Usually, though, they will fall back onto the galaxies.
The gas being expelled can also fall back again, in which case we call it "galactic fountains". It may also leave the galaxy, enriching the intergalactic medium with metals.
-
$\begingroup$ I'm familiar with the gas billowing above and below the galactic plane. My question has more to do with the stars in the disk. Do we know whether these stars are falling in or out or in a stable orbit? $\endgroup$– user32023Commented Mar 4, 2015 at 13:28
-
$\begingroup$ In general, they don't. Outflows are caused by feedback processes from exploding supernovae heating up their surroundings, increasing its buoyancy, and from radiation pressure from hot (O and B) stars. The effect of this on such massive bodies as stars is miniscule. But in principle if 3+ stars come close enough, one may get slung out of the galaxy. This happens extremely rarely (or we would see many extragalactic stars), except in the case of galaxy mergers. If a star doesn't encounter bodies of similar masses, their orbits are stable. $\endgroup$– pelaCommented Mar 4, 2015 at 13:40
-
$\begingroup$ So, if a supernova balst wave passed through a molecular cloud containing stars, the gas and dust would get blown away, while the star would not move much. They would probably follow the gas a little due to its gravitational attraction, but since they are so massive and the gas velocities involved are so high, my gut feeling is they would soon "lose touch" with the gas and get decoupled. My gut feelings are backed up by observations and, for me in particular, by cosmological, hydrodynamical simulations. $\endgroup$– pelaCommented Mar 4, 2015 at 13:45
-
$\begingroup$ Thanks very much for the info, but I'm trying to separate speculation from actual data. Intuition tells us that the stars are under the influence of the inner mass and should either be falling in or in a stable orbit. But intuition tells us they should follow Kepler's law, so my question is really about kinematics: do we have any objective evidence, one way or another, showing the radial direction of the average star in the disk area of a spiral galaxy? $\endgroup$– user32023Commented Mar 4, 2015 at 13:53
-
$\begingroup$ Okay, I'm sorry, by "stellar material" you mean "stars". Yes, we have both observational evidence, theoretical models, and computer simulations showing that stars follow circular, not-very-elliptical paths, although they wiggle a bit up and down in the potential. Stellar feedback creates density waves in the plane of the disk which initiate new star formation (which we see as spiral arms), but only in rare, close encounters can individual paths be perturbed enough to send a star on some crazy path. I guess googling "stellar disk dynamics" could lead you to useful literature. $\endgroup$– pelaCommented Mar 4, 2015 at 19:38
