The term modulation as used here means amplitude modulation.
If you have some wave with a high frequency, call this $f(t)$, then you can multiply it by another signal with a much lower frequency, call this $g(t)$. The effect of $g$ is basically to modulate the intensity of $f$ up and down as a function of time.
In this case when you sum your two waves with frequencies $\omega_1$ and $\omega_2$ you get a wave $f(t)$ with a frequency $(\omega_1 + \omega_2)/2$, multiplied by a wave $g(t)$ with frequency $(\omega_1 - \omega_2)/2$. So the signal you observe is the product $g(t)f(t)$. If $\omega_1 \approx \omega_2$, which it is in this case, then $f$ is high frequency and $g$ is low frequency. So $g$ modulates $f$ exactly as in amplitude modulation.
It's the variation in the intensity of $f$ caused by $g$ that we hear as the beat.
Response to comment:
Have a look at this image from this article about amplitude modulation:
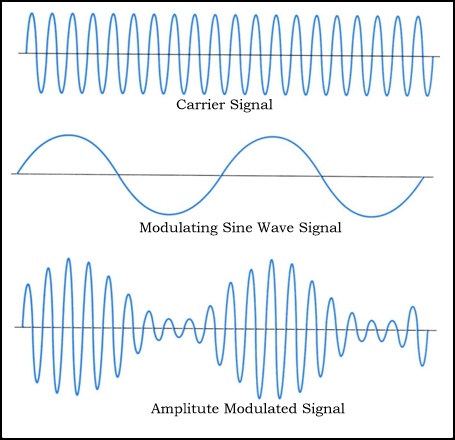
When the modulation is described as slow this means slow compared to the carrier frequency. That is, it means the frequency of the modulating wave $g$ must be a lot less than the frequency of the carrier wave $f$.
In your system the carrier signal is the function I've called $f(t)$ with a frequency $(\omega_1 + \omega_2)/2$. The modulating signal is the function I've called $g(t)$ with a frequency $(\omega_1 - \omega_2)/2$. Hopefully it's obvious that the frequency of $f$ has to be much higher than the frequency of $g$ because that gives a nice clear modulation. If the frequencies are too similar then when you multiply $f$ and $g$ you just get an unholy mess with no clear beat pattern.
If $\omega_1 \approx \omega_2$ the the average of the two frequencies is a high frequency and the difference is a small frequency.

