Muon decay why proper length in the earth frame?

Question

Consider the two diagrams below (ignore the velocities),

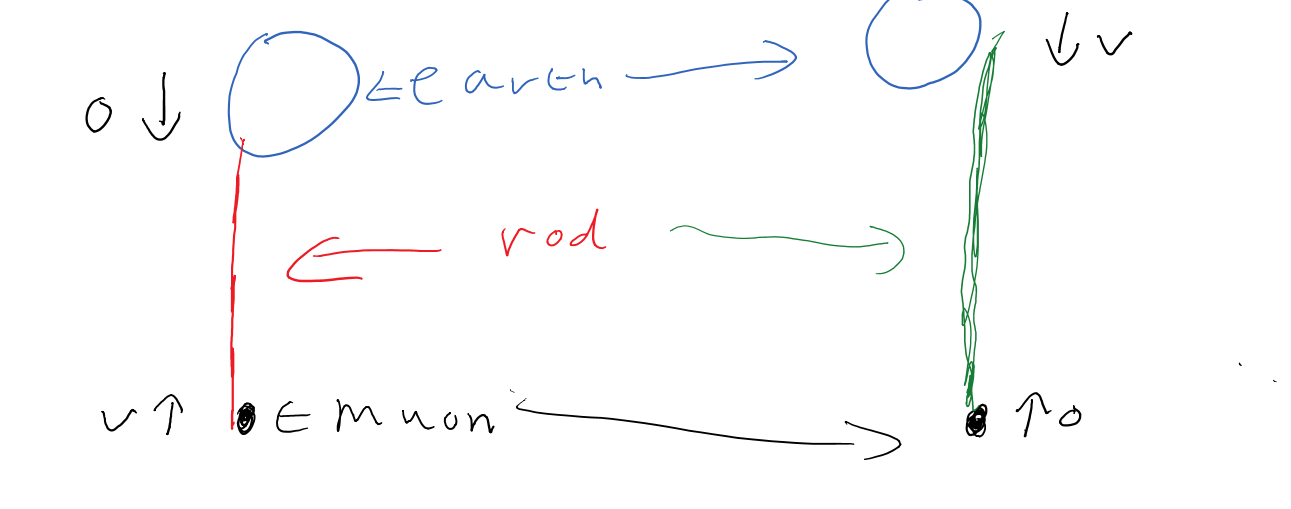
Consider the situation in the right hand picture where we have a rod connected to the earth. Let us now say that the muon is created at the end of the rod, both frames (the earth and muon frame) most agree on this fact, since the laws of physics are the same in both frames. Then it should be obvious that according to the muon the rod is length contracted and thus it has a shorter distance to travail then an observer in the earth frame would say the muon had to travail. Now consider the left hand diagram where the rod is instead connected to the muon (and therefore at rest with respect to it). In this frame the rod will therefore be contracted to someone at rest in the earth frame compared to the muon frame (and thefore the observer in the earth would say the muon has a shorter distance to travil then someone in the muon frame would say). It should be clear that that these two arguments contradict one another. So why is the first analysis, where the length is contracted in the muon frame correct whilst the second is wrong.

(note that the rods are included simply for illustration)

Answer 1

If the rod is connected to the muon, inertia suggests it has the same velocity as the muon and the rest frame of the rod is the same as the rest frame of the muon. So the muon-connected rod has its proper length in the muon's rest frame.

Likewise if the rod is connected to the earth, it has its proper length in the earth's rest frame.

The rod is always longest in the rod's rest frame, and has a contracted length in all other reference frames.

But what is the rod for? Suppose we want to use the rod to measure the muon's flight distance, so that the muon is born at one end of the rod and decays at the other. (For sake of argument we'll assume we can predict exactly when the muon will decay, rather than the half-life which is averaged over many different decays.) The rod in the earth's frame is pretty easy: the decay end is at our detector on the ground, and the birth end is somewhere in the upper atmosphere.

The rod attached to the muon is more subtle: the rod and the muon, in that reference frame, both have zero velocity, so the muon dies at the same end of the rod where it was born! In its rest frame the distance traveled by the muon is zero.

This is why we talk about length contraction in one case and time dilation in the other. The muon's rest frame is actually kind of boring: the muon sits alone, at rest, at the origin, and waits to die while the universe rushes around it. Keeping track of whether it's time to decay or not is essentially the only job that the muon has, which means that the decay always takes (on average) the "proper" time. Length contraction of the earth-atmosphere thickness is the only way to reconcile the muon's proper lifetime with the observational fact that we get lots of muons at sea level.