Muon decay in muons frame of reference, time muon expects us to record

Question

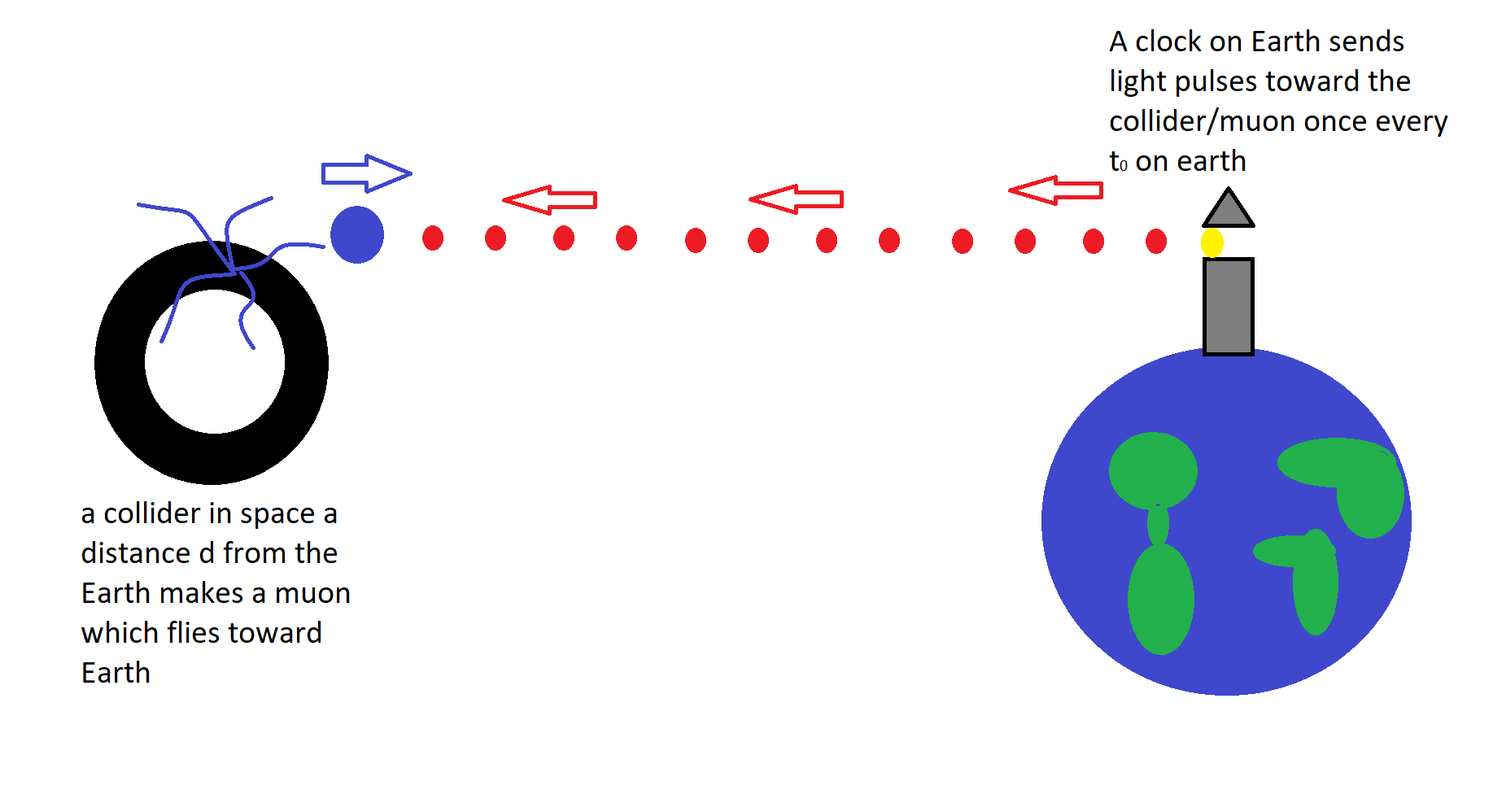
I have already asked a question regarding this concept but it was flagged as a duplicate. I know this misconception is very common for special relativity but I haven't found a question that talks about the misconception I'm having. Or atleast I haven't made the link between their question and mine. My question is why does it look like in this case the factor by which the clocks are slower is $γ^2$?

On the earth lets say a muon has to travel a distance $d$ at a speed $v$. We expect the time it takes for the muon to reach us as time $t$, where $t=d/v$, but factoring in time dilation of running clocks, the muon has only experienced time $t/γ$ in our frame of reference, hence we can observe it on Earth.

But in the muons frame of reference, the distance it has to travel is shorter by a factor of gamma, so the time it experiences to travel to earth will be $t_2$, where $t_2=v/(d/γ)$. This is the same time as we record in our frame of reference. But according to the muon, we too have our clocks running hence we should record in our labs, a dilated time, $t_2/γ$. This is our original expected time $t$, but reduced by a factor $γ^2$.

Why is this so? I am sure it has something to do with the simultaneity of events but I don't know where to start.

Answer 1

Just like with the twin paradox (and to some extent the train-tunnel paradox), your mistake is not taking into account the changing distance between the two objects, and how light signals sent from one object to the other would appear. In conclusion we will find that when a spaceship flies toward earth at relativistic speeds, rather than perpendicular to its displacement with earth, clocks on the Earth visually appears to be sped up, not slowed down.

Let's be more careful with the setup. A muon is created at a distance $d$ from the earth moving toward Earth with velocity $v$ straight toward the Earth. Let's say on Earth there's a clock that sends a light pulse to the muon once every $t_0=10^{-9}\,\text{s}$ (chosen so it gets many pulses before it decays) on Earth, and let's count how many pulses the muon receives as it traverses the distance $d$ (distance $d$ from the earth's perspective, and distance $d/\gamma$ from the muon's perspective). It'll be important that the clock was running and sending light pulses long before the muon was born. Because "when the muon was born" is not a well-defined moment on Earth, which is not the location the muon was born in. The number of light pulses that hit the muon between its creation and its arrival at Earth should be an agreed number in all reference frames. Note that muons are actually formed in collisions in the upper atmosphere between atmospheric atoms and high energy particles from space... but anyway please accept my example as a replacement of reality.

As you point out, the muon is aware of time dilation, and from its perspective time runs more slowly on Earth by a factor of $\gamma$. So it believes that light signals are being sent once every $t_0\gamma$. But the distance between the Earth and the muon at time $nt_0\gamma$ is $$D_n=d/\gamma-vnt_0\gamma$$ Of course light has the same speed in all reference frames. So this light pulse, sent at time $n t_0\gamma$ is received at time $$T_n= nt_0\gamma+d/\gamma c-vnt_0\gamma/c$$ So the time between the muon recieving light pulses is (note $\beta=v/c$): $$ T_{n+1}-T_n=t_0\gamma-\beta t_0\gamma=(1-\beta)\gamma t_0<t_0 $$ So yes, time visually appears to be sped up on earth when viewed by the muon. The total time the muon is moving toward the Earth is $d/(\gamma v)$, so the number of light pulses that are received is: $$ N=\frac{d/(\gamma v)}{(1-\beta)\gamma t_0}=\frac{d}{vt_0}\frac{1}{(1-\beta)\gamma^2}=\frac{d}{vt_0}(1+\beta) $$ Well shit... the naiive guess was that $d/vt_0$ signals should have been sent by earth between the creation of the muon and its arrival. But that's not what we got! So what went wrong? Well let's analyze this from the Earth's perspective, and let's not forget that many signals were still between the earth and the muon creation point before the muon was ever created.

The people on earth think that there are light pulses flying through space a distance $t_0c$ apart (and a speed $c$). And there's a muon flying toward earth, starting at a distance $d$ moving toward earth (and toward those light signals) at a speed $v=\beta c$. So the time between collisions between the muon and the light pulses is $t_0c/(c+\beta c)$. People on Earth believe it should take a time $d/v$ for the muon to arrive, so the total number of collisions is: $$ \frac{d/v}{t_0c/(c+\beta c)}=\frac{d}{vt_0}(1+\beta) $$ All good. Everyone agrees.

Answer 2

This is a classic paradox. Let's set $c = 1$ and start by working in the lab frame, where the muon is created at $x = 0$ and $t = 0$ and travels to the right with speed $v$. It decays at $x = d$ at the moment it crashes into a clock, which reads $t = t_{c,f}$. Then of course, we have $$d = v t_{c,f}, \qquad t_{c,f} = \gamma \tau$$ by the definition of speed and time dilation, where $\tau$ is the lifetime of the muon in its rest frame.

Now let's work in the muon's frame, described by primed coordinates. The muon is created at $x' = 0$ and $t' = 0$. At this moment in the muon's frame, $t' = 0$, the clock is at $x' = d/\gamma$ but its reading $t_{c,i}$ is not zero. Instead, performing a Lorentz boost back to the lab frame, $$t_{c,i} = t = \gamma (t' + v x') = \frac{\gamma v d}{\gamma} = v d.$$ Of course, the clock will crash into the muon at $t' = (d/\gamma) / v = \tau$, which is precisely when the muon decays. At this moment, the reading on the clock is $$t_{c,i} + \frac{\tau}{\gamma} = v d + \frac{d}{v \gamma^2} = \frac{d}{v} \left(v^2 + \frac{1}{\gamma^2} \right) = \frac{d}{v}$$ which is precisely the final reading $t_{c,f}$ we found in the lab frame. So the paradox is resolved by remembering that $t_{c,i}$ isn't zero, due to the relativistic loss of simultaneity effect.

Of course, this answer depends on the machinery of Lorentz transformations, reference frames, and synchronized clocks. AXensen's answer gives a different explanation directly in terms of what the muon and Earth observers "see".

Answer 3

It is to do with simultaneity. The time dilation equation only applies where you compare an interval, t',between two events that occur in the same place in one frame and the corresponding interval, t, between the same two events in another frame where they occur in two different places. The two events in the case of the muon are the start end end of its journey through the atmosphere, which occur in the same place in the muon's frame and in two different places in the Earth frame.

If you want to apply the equation from the perspective of a muon, you have to pick a pair of events that occur in the same place on Earth. Let's say the two events are two consecutive ticks on your watch. In the muon's frame, they will be about a minute apart (assuming the speed of the muon is such that the time dilation factor is around sixty).