I am learning about experiments on Quantum Optics and Quantum Tomography in order to understand how to measure two qubits with an arbitrary quantum state of their polarization degrees of freedom. Since I need to work with two-particle system, it is normally said that I have to work with a "black box" which provides me photon pairs. Something like this
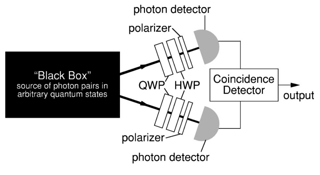
As it seems like a SPDC device, I suspect is a sort of entangled photons device (also because of the Coincidence Detector). According to what I understand by entangled photons, are states expressed like this
$$ \frac{1}{\sqrt 2}(|0\rangle_A \otimes |1\rangle_B + \mathrm{e}^{i\phi}|1\rangle_A \otimes |0\rangle_B) $$
But, as I precise to work with a tensor product state (two systems), I believe that I must use states like this
$$ a|0\rangle_A \otimes |0\rangle_B + b|0\rangle_A \otimes |1\rangle_B + c|1\rangle_A \otimes |0\rangle_B + d|1\rangle_A \otimes |1\rangle_B$$
Can that "black box" produce both types? if not, Can I use entangled photons as a two-particle system with an arbitrary quantum state?
