Elasticity of a body on which variable force is applied

Question

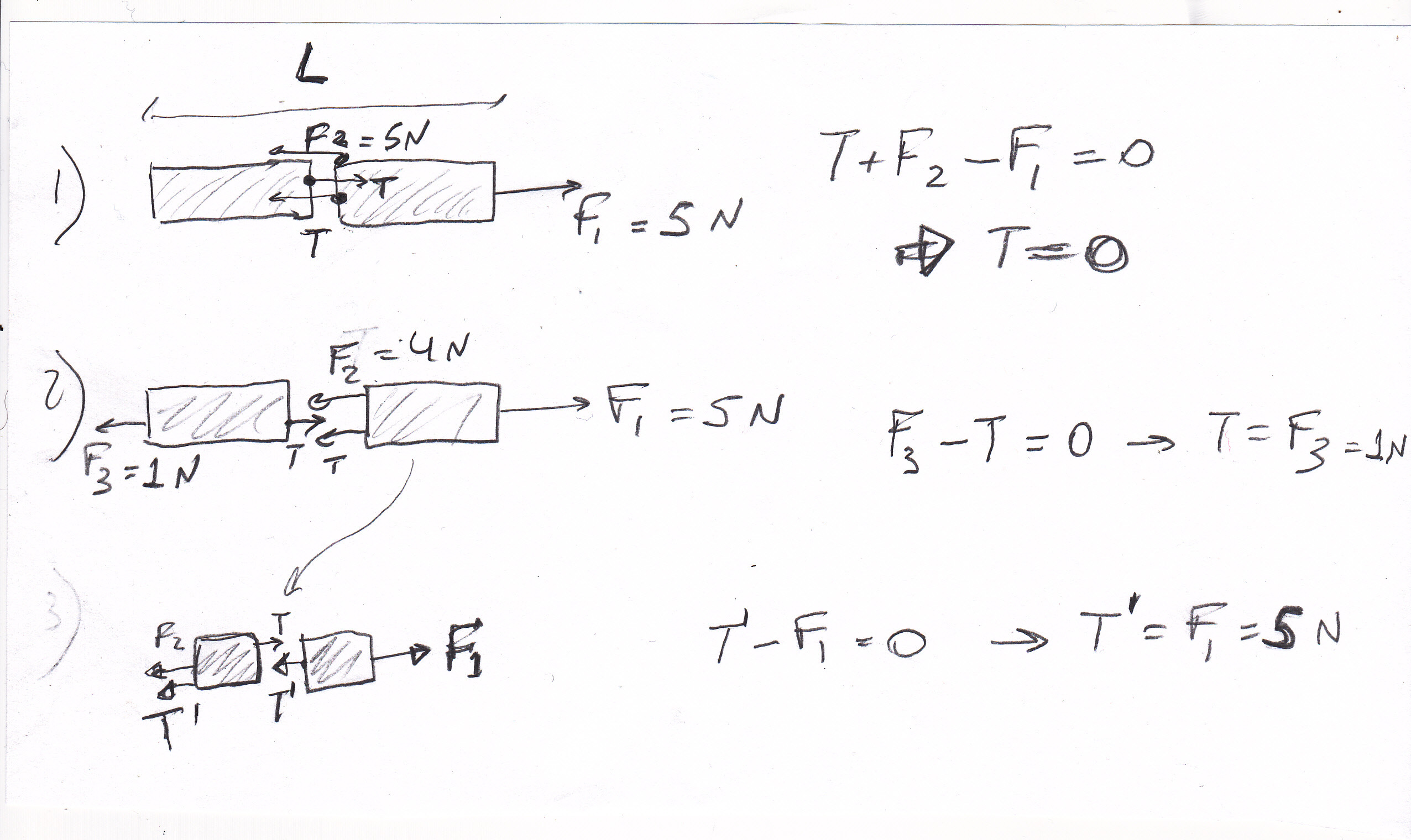
Let us consider a rod of some cross sectional area $A$ and length $L$. At one end a longitudinal foce of 5x acts on the rod. At a distance of $\frac{L}{2}$ from that point longitudinal force of 5x acts on the rod in the opposite direction. My doubts are:

Since the body is in equilibrium: the tension center of rod and the end where force is applied must be 5x? At the other end of the rod will any tension be present? (The end where no force is applied) does the entire rod elongate or only a part? If its a part, which part? Please provide some intuition.

Finally let us consider the same rod but this time- At one end 5x newtons act. At center of the rod 4x newtons act in the opposite direction. And at the other end x newtons act in the opposite direction. Thus the rod is in equilibrium:

Is the elongation in the rod uniform?

How is tension distributed along the rod? Will it exist such that every point on the rod is in equilibrium? Is it only the tension that will cause elongation? What will be or how should we find tension at a distance of $\frac{2L}{3}$ from the end where 5x newtons is applied?

Rod is of uniform density and Young's modulus $Y$ It follows hookes

Answer 1

You can analize the problem by imaginarily breaking the rod and calculating the internal tension, as in the pictures below. In the first case, you get that the half between the force at the center and the force-free end has no internal stress see(1), and thus it does not elongate. The other half however, has an internal tension T' (see (3) ) of 5N at any point inside, and thus elongates uniformily.

In the second example the first half has an internal tension of 1N ( see (2))and the second and internal one of 5N (see (3)). Each half elongates uniformily, but the elongation of each half is different. the tension at 2L/3 will be 1N.