2
$\begingroup$

enter image description here

My teacher gave this question as homework while we were learning Elasticity in class.

Question description:

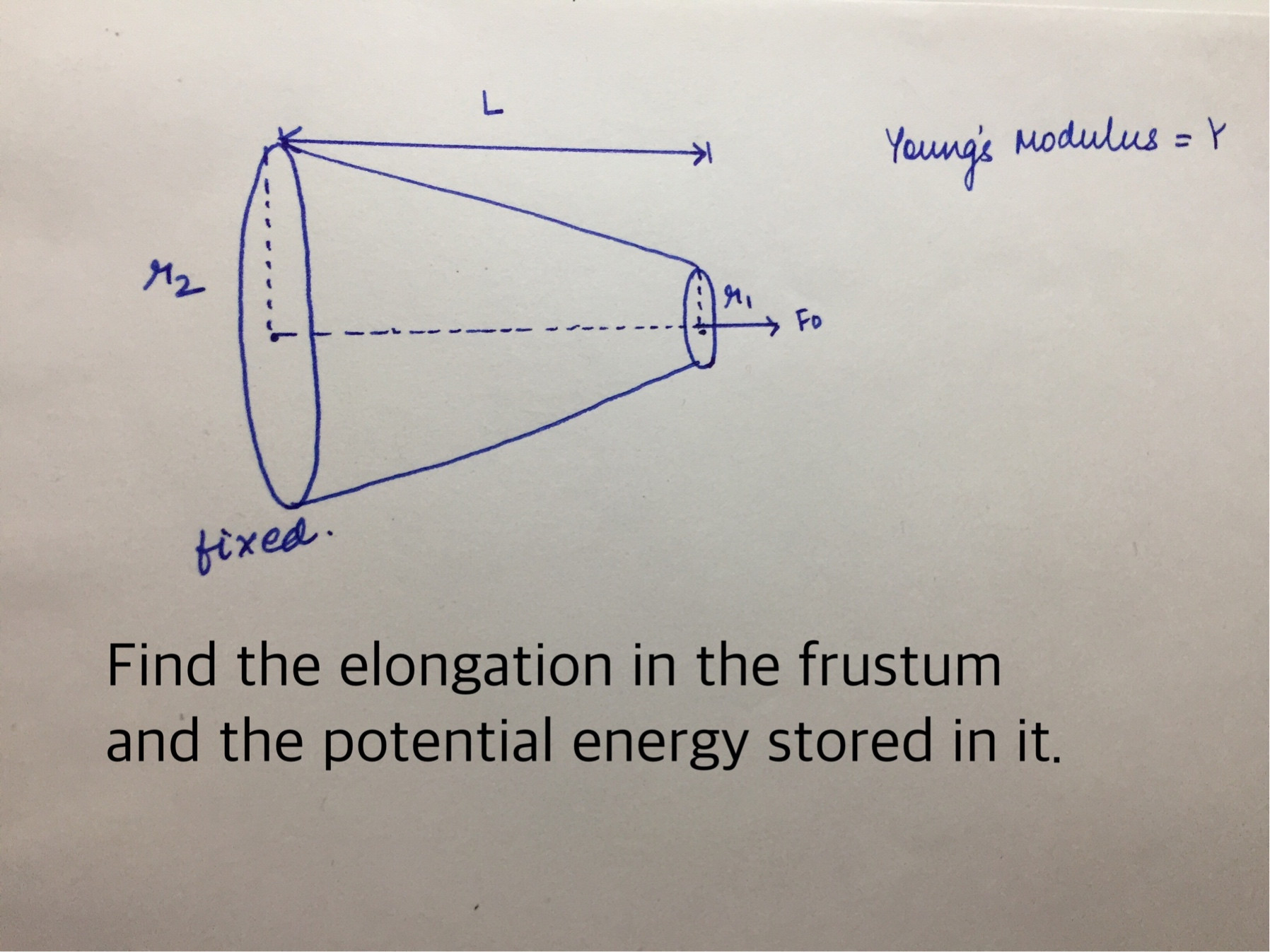
Objective: To find the total elongation in the frustum as well as the potential energy stored in it.

Given: Young’s Modulus of the frustum, $Y$

Height of the frustum, $L$

Smaller radius, $r₁$

Larger radius, $r₂$

Further details: The larger end of the frustum is fixed. A force F₀ is applied on the smaller end of the frustum.

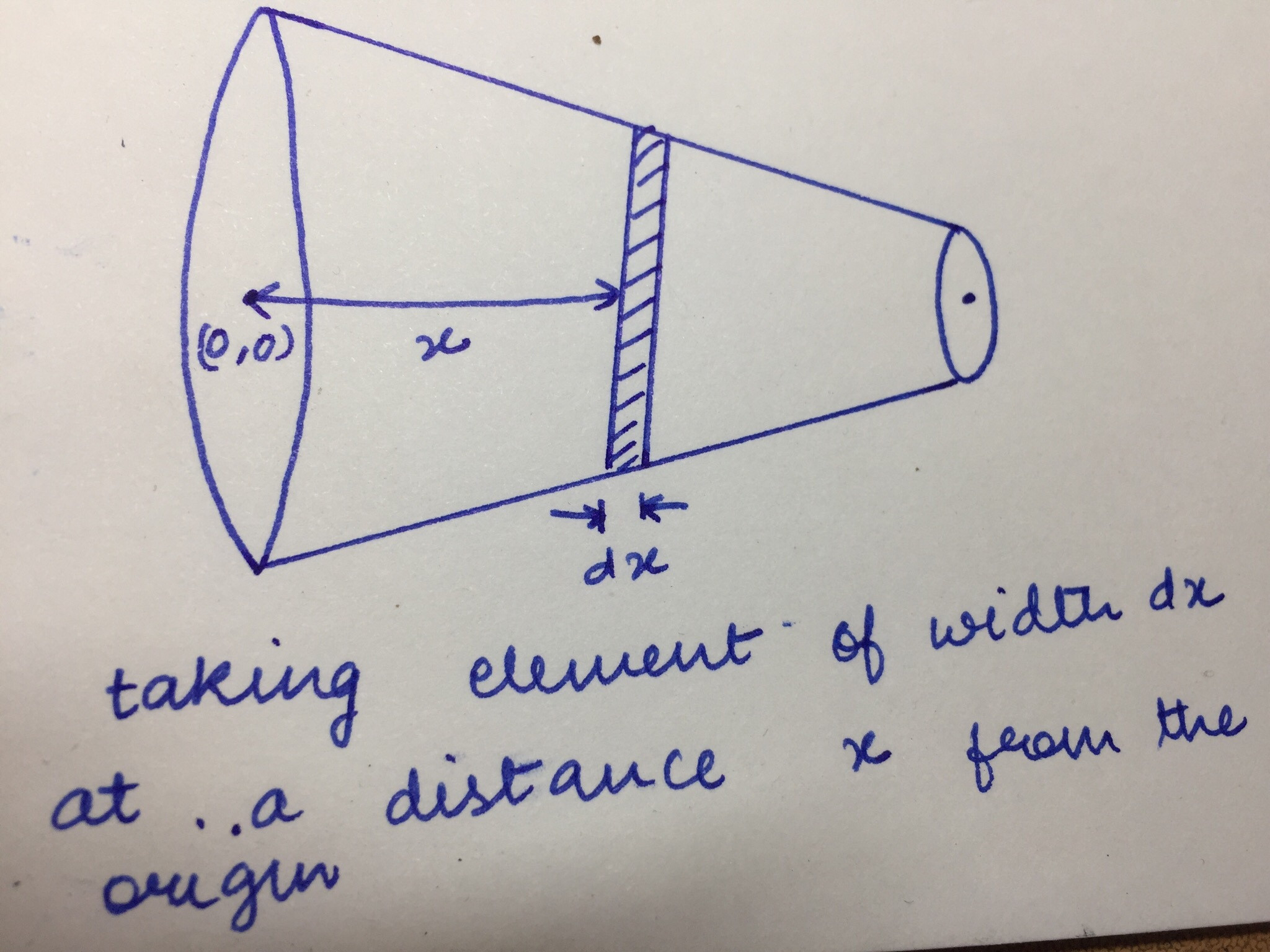
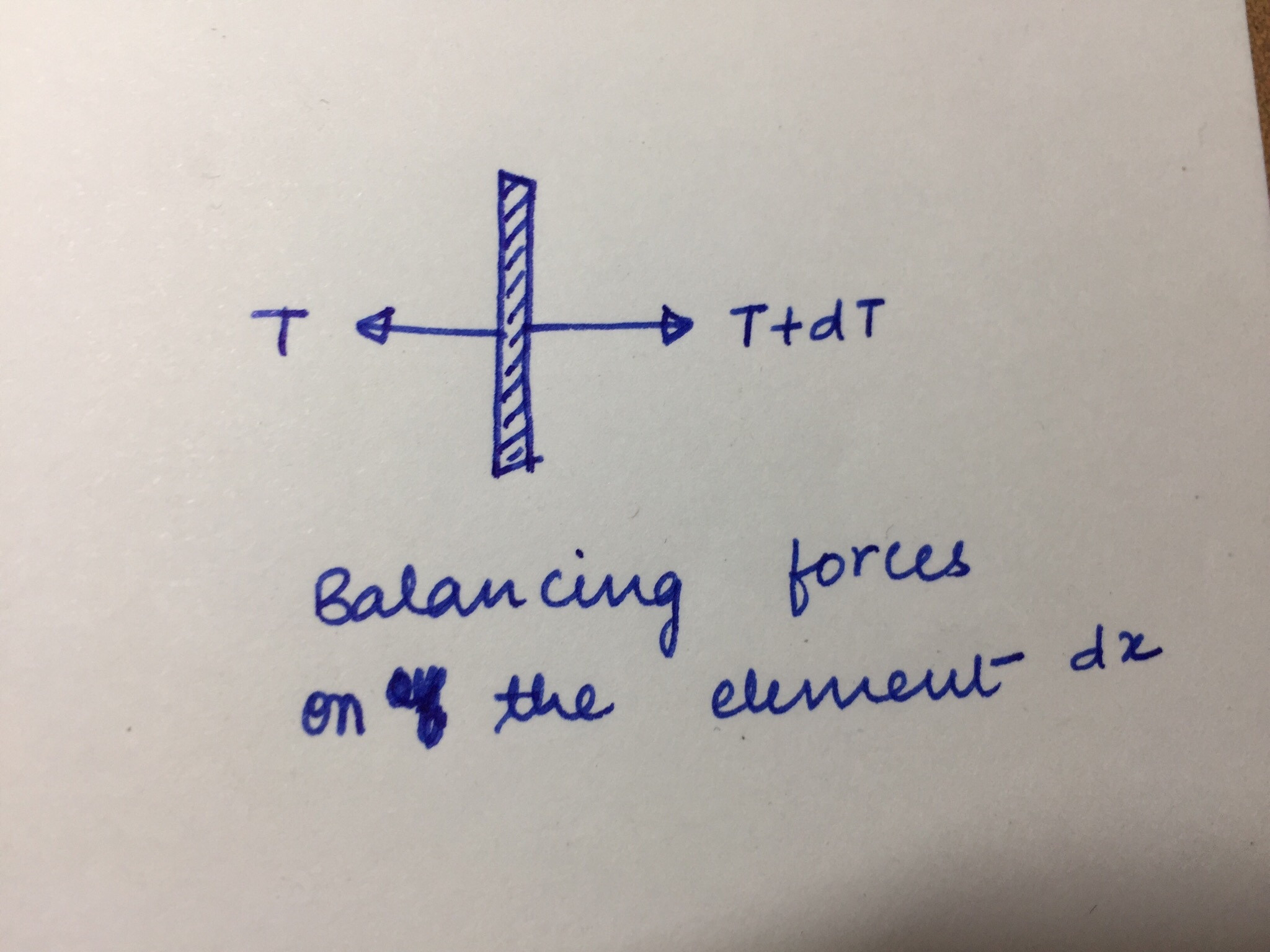
Attempt at the problem: I took an element dx at a distance x from the larger end and tried balancing forces on the element as follows:

enter image description here enter image description here

Where $T$ is the tension acting on the element due to it’s neighbouring elements. I am stuck here as I am not able to figure out what force equalises the extra dT tension on the element, since the net force on the element should be zero as there is no net acceleration of the frustum as a whole. Does this mean the element as an acceleration towards the right? If not, which force will equalise the extra tension on the element? Please help me out.

Improve this question
$\endgroup$
1
  • $\begingroup$ I think that you take this as a statics problem with each incremental disc suffering a different stress due to the change in area. At a given position $x$ you can find the radius of the incremental disc in terms of $r_1,\, r_2, \,L$ and $x$. This will give you the stress assuming a constant force $T$ is acting on each side of the incremental disc. Then use you definition of Young's modulus to find the the incremental extension and hence by integration find the total elongation. $\endgroup$
    – Farcher
    Commented Mar 18, 2018 at 10:52

3 Answers 3

Reset to default
2
$\begingroup$

The tension is constant, independent of x. It is the tensile stress that is changing. $$\sigma(x)=\frac{T}{A(x)}=E\frac{du}{dx}$$where u is the displacement and A(x) is the local cross sectional area. You just integrate this equation to get u(L).

Improve this answer
$\endgroup$
-1
$\begingroup$

The force dT that acts on the small element is the reason for the elongation for the frustrum. It results in an acceleration that is very very small. This acceleration is the reason for the elongation of the frustrum. The element dx moves a distance that is caused by the acceleration or the dT. That is the reason why the point where the force is applied elongates the highest as dT here is highest.

Improve this answer
$\endgroup$
1
  • $\begingroup$ But to find the elongation, I need to know the tension as a function of x. For that I need to equate dT to something and integrate. How do I proceed? $\endgroup$
    – Aura Sartori
    Commented Mar 18, 2018 at 9:11
-1
$\begingroup$

For that you can use Youngs modulus or stress strain equation. The length is x elongation dx and tension dT. Therefore Y=(dT/a) *(dx/x). dx is the elongation of the element. Note that the area varies as x. U shall have to take into account of that

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.