Force required to move bar at one end

Question

A uniform bar of length $L$ and weight $W$ rests on a level floor so that there is a uniform contact pressure between floor and bar. A horizontal force $P$ is applied at one end, normal to the bar. If the coefficient of friction between bar and floor is $\mu$ show that the force $P$ needed to move the bar slowly is $(\sqrt{2} - 1) \mu W$.

I'm having trouble with this question, specifically with how friction acts on the bar. I believe that the approach should be to formulate equations for translational and rotational equilibrium, and since we are only considering horizontal forces there should only be two forces of interest, $P$ and friction.

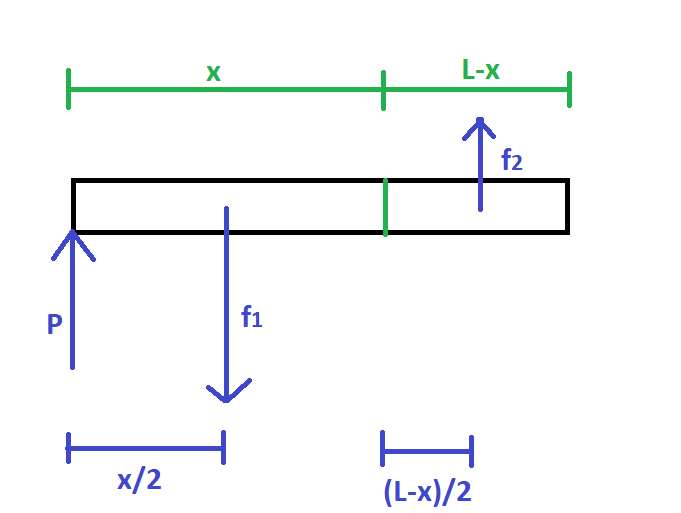
However, I'm confused as to how friction is distributed across the bar (as opposed to $P$, which acts only at one end).

• What can we say about the direction of friction acting on the bar? Initially, I thought the friction should always act opposite to $P$. However, I'm now thinking that the friction acts opposite to $P$ closer to $P$ and acts in the same direction as $P$ nearer to the other end, because the bar would pivot about some point on the bar - would that be right?

• Can we assume that the magnitude of the friction is uniform across the bar? More generally, if the contact pressure is uniform for any object can we say that the friction is also uniform (even if the object is experiencing different loads at different places)?

Any help would be appreciated.

Answer 1

I will point you in the right direction, as this is a homework question. The key here is to start general and break the friction force into two parts acting on the parts of the bar that are moving in different directions.

Let's say we have the bar along the $x$-axis, and we are applying our force $P$ at the left end of the bar in the positive $y$ direction. Now, let's partition the bar into two pieces: one of length $x$ (left side) and the other of length $L-x$ (right side). The first section we will take to be moving upwards, and the second section will be moving downwards. In other words, if we were to move along with the bar we would see it rotating clockwise about a point a distance $x$ from the left end of the bar. Therefore, the friction force will be acting downwards on the first section (force $f_1$), and will be acting upwards on the second section (force $f_2$). This should answer your first question.

Now, the fraction of the bar's weight on the left partition is $\frac xL W$, and the fraction of the bar's weight on the right is $\frac{L-x}{L} W$. Because of the uniform contact pressure, we can take $f_1$ to have a magnitude of $f_1=\frac xL\mu W$ acting at a distance $\frac x2$ from the left side of the bar. Similarly, we can take $f_2$ to have a magnitude of $f_2=\frac{L-x}{L}\mu W$ acting at a distance $x+\frac{L-x}{2}=\frac{L+x}{2}$ from the left side of the bar. This should answer your second question. We are using a model of friction where the magnitude of the force only depends on the normal force, so it's magnitude is constant for each segment that is moving in the same direction.

This set up could be a lot to take in, so I have included a diagram below to help make more sense of how we have set the problem up

Now for the physics. We can assume "moving slowly" means no net force and no net torque about any point$^*$ (a good point to choose is the left end of the bar where the force is being applied so that $P$ has no torque). If you set up these two equations you should be able to solve them for $P$ and $x$. I will leave it to you from here to set up and solve the system of equations. Let me know if I can explain anything better. Good luck!

---

$^*$ This is therefore assuming that the bar is already moving before we physically set $P$ to the desired value