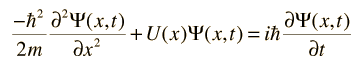Collapse of the wave function and Heisenberg uncertainty

Question

I have been studying quantum mechanics for a few weeks, in particular wave mechanics, as created by Schrodinger, and his equation. As a high school student, I haven't found an answer to this question on the web, so I wanted some help understanding it.

Schrodinger's cat is an example of a paradox including wave function collapse. We do not know if the cat is dead or alive until we observe it, etc. However, by heisenberg uncertainty, we cannot measure the exact momentum or position of a particle/wave ever - therefore, how does the wave function collapse into one quantum state? wouldn't that require the particle to have some exact momentum/position that we have observed?

In addition, we know that the Hamiltonian represents the sum of kinetic and potential energy in a system. However, I'm not quite sure why, intuitively, the time dependent version of the Schrodinger equation becomes $H$$\psi$=$i\hbar$ $\partial$/$\partial$$t$ $\psi$$(r,t)$. where does the $i\hbar$ come from? why does the sum of kinetic and potential energy equal to that?

Answer 1

I will answer this part

In addition, we know that the Hamiltonian represents the sum of kinetic and potential energy in a system.However, I'm not quite sure why, intuitively, the time dependent version of the Schrodinger equation becomes Hψ=iℏ ∂/∂t ψ(r,t).

Quantum mechanics was developed slowly, because experiments showed that light came in quanta from the hydrogen atom. At that time they were still thinking classically, and Bohr developed a model of an electron rotating around a proton for the hydrogen atom, similar to the way the moon rotates around the earth. BUT there was a problem for this. In classical electricity and magnetism the electron would not stay in an orbit but would lose energy and fall on the proton.

Bohr postulated that it was a standing wave, and postulated only certain orbits ; electrons could fall from one to the other emitting a photon of energy hnu ( nu the frequency). That the photon's energy came as hnu was known from the photoelectric effect and from black body radiation. The model then explained the spectrum of hydrogen which had been fitted with a series.

This is how the h enters the game. Because the model has to take into account that an electron changing energy levels will release from the system energy proportional to h*nu.

The Schrodinger equation gives the same series as a solution to the hydrogen problem, but now it comes as a theory which is much more general. h necessarily has to play its role.

Quantum mechanics has a number of postulates.

1. Associated with any particle moving in a conservative field of force is a wave function which determines everything that can be known about the system.

   2. With every physical observable q there is associated an operator Q, which when operating upon the wavefunction associated with a definite value of that observable will yield that value times the wavefunction.

   3. Any operator Q associated with a physically measurable property q will be Hermitian.

   4. The set of eigenfunctions of operator Q will form a complete set of linearly independent functions.

   5. For a system described by a given wavefunction, the expectation value of any property q can be found by performing the expectation value integral with respect to that wavefunction.

   6. The time evolution of the wavefunction is given by the time dependent Schrodinger equation.

Number 2) of these postulates is what relates to your question.

where does the iℏ come from? why does the sum of kinetic and potential energy equal to that?

The h bar comes so that the dimensions and the energy of the photon comes out correctly as h*nu. The complex "i" so that the equation has the form that will give the appropriate solutions.

The operator for the time dependent Hamiltonian is $i\hbar$$\partial$/$\partial$$t$

So the $H$$\psi$=$i\hbar$ $\partial$/$\partial$$t$ is an identity, used to solve for a time dependent psi:

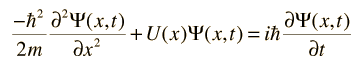
The formalism developed by trial and error in the beginning, fitting the models to the data and then using the models to predict further behaviors.The successful fitting of the same spectral series as the Bohr model led to the development of quantum mechanics, rather than the theory coming first and then looking at the data.

The real answer is that this mathematical formulation fits the data and has great predictive power proven over and over again.

Answer 2

You ask a couple of different questions:

1) You say "by Heisenberg's uncertainty, we cannot measure the exact momentum or position of a particle/wave ever". No, Heisenberg's uncertainty principle doesn't say that. It says that IF you measure the position of a QUANTUM particle with precision Δx, i.e. you localize the particle within an interval Δx, then the linear momentum is DISTURBED, it's imprecision becomes AT LEAST ħ/2Δx . So, you can measure the position with whatever precision you want, but there will be a price to pay in the position of the particle after some time t, because if the perturbation in the linear momentum, Δp, is very big, tΔp is big and the particle can be projected into a wide region in the space.

2) A quantum particle, to say it simply, has a wave-function. A classical object cannot have such a thing, if we try to calculate its wave-function, it is much, much smaller than the object.

3) You ask "how does the wave function collapse into one quantum state? wouldn't that require the particle to have some exact momentum/position that we have observed?" The correct answer to this question is WE DON'T KNOW what was the EXACT position BEFORE we measure position. Only if we PREPARE the particle in a position-state named delta-Dirac, (did you already learn of it?), only then the particle has a well defined position before the measurement. But according to Heisenberg's principle, the linear momentum is completely undefined - takes ANY value. This is what the STANDARD quantum mechanics says. There are different attempts to say other things, but each of these attempts has its drawbacks.

4) The Schrodinger equation is a version of the heat-propagation equation. But it has IMAGINARY diffusion coefficient. WHY? One answer is that the solution - the wave-function - is complex. Another answer, quantum mechanics comes with many strange things.

I hope it helps