Neutrino-Neutron Interaction Feynman Diagram (W Boson Direction)

Question

I am currently studying differential cross sections for my Nuclear Physics module. I'm looking an experiment where muon-neutrinos are interacting with nucleons in a scintillator producing muons (which then cause the scintillation to occur).

I went ahead and drew a Feynman diagram for the interaction: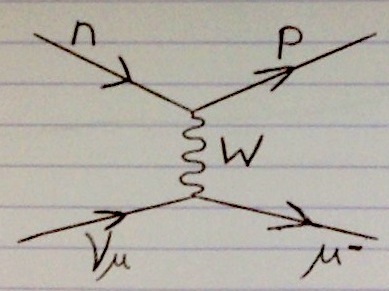

I've read that the direction of the boson (and whether it is $ W^+ $or $ W^- $) depends on the 4-momentum transfer. In this case high energy muon-neutrinos are interacting with low energy (static) neutrons.

So my question is: Should the boson be a $ W^+ $going from the neutrino to the neutron or a $ W^- $going doing the opposite? I was originally going to just write $ W^±$without a directionality arrow, is this too an accepted convention?

Any advice would be much appreciated! Thanks, Sean.

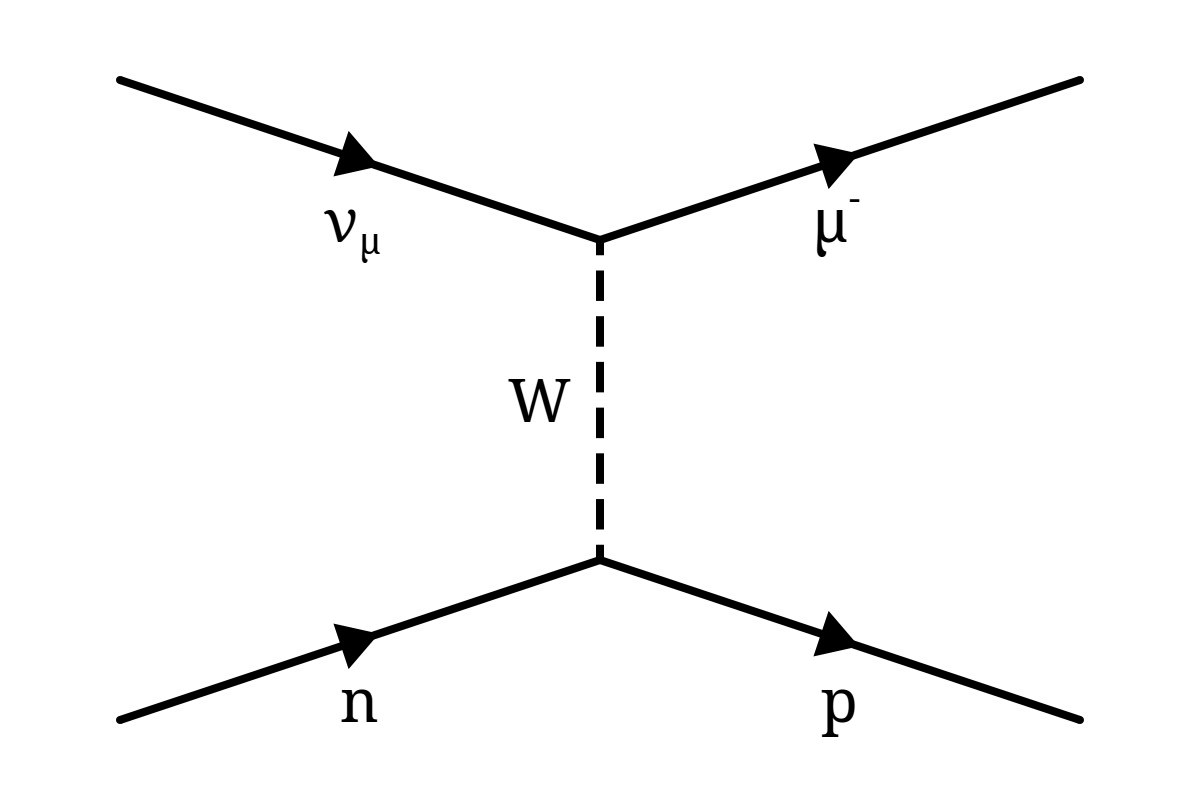
Edit: Here's a model example taken from http://danielscully.co.uk/thesis/interactions.html

Answer 1

Let's just focus on the top vertex.

First, draw an arrow pointing down. Then, you can determine from charge conservation (or looking for the relevant term in the lagrangian) that the particle must be $W^+$. Because $(W^+)^\ast=W^-$, this vertex has precisely the same value if you reverse that arrow and replace the $W^+$ with a $W^-$. Here I am just using the fact that arrow reversal corresponds to replacing the particle by its antiparticle.

Thus, not only do the different diagrams have the same value, in this sense, they are the same diagram. In particular, you don't 'double count' by adding the value of a $W^+$ diagram to the value of a $W^-$ diagram.

For what it's worth, I think it's worthwhile to verify this at the level of the Lagrangian. There is only a single term in the lagrangian that corresponds to the upper vertex interaction, but that term has two names, one with a $W^+$ and one with $(W^-)^\ast$.

So yes, you can safely leave the direction off and simply label it with $W$.