1
$\begingroup$

Hi guys I'm totally stuck on the following question:

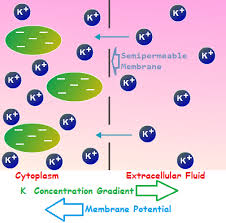
enter image description here

We know the ionic flux between two chambers due to uneven concentration can be computed by the Nernst-Planck equation

$$j = -D_p \left(\nabla C + \frac{Z_pF}{RT}C \, \nabla\phi \right)$$

where $C$ is the concentration, $\phi$ is the potential in chambers and $Z_p$, $F$, $R$ and $T$ are constants. At equilibrium, $j = 0 $ and $$-\nabla C = \frac{Z_pF}{RT}C\, \nabla\phi$$

Can anyone solve for the Nernst Potential (equilibrium potential) $Vm$ = $\phi_i - \phi_o$, where $i$ denotes inside and $o$ denotes outside without reducing the question to one dimension? The main problem I have is taking the volume integration of $\nabla C/C$.

Improve this question
$\endgroup$
1
  • $\begingroup$ LaTeX tips (see edit): When terms inside a set of brackets do not fit, use \left( ... \right) to ensure the brackets scale properly. In your case, some symbols may be too close to each other, or nearly touch, so use \, to add a tiny space. $\endgroup$
    – JamalS
    Commented Oct 7, 2014 at 9:02

0

Reset to default

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.