Reluctance of torus shaped iron core with embedded wire loop

Question

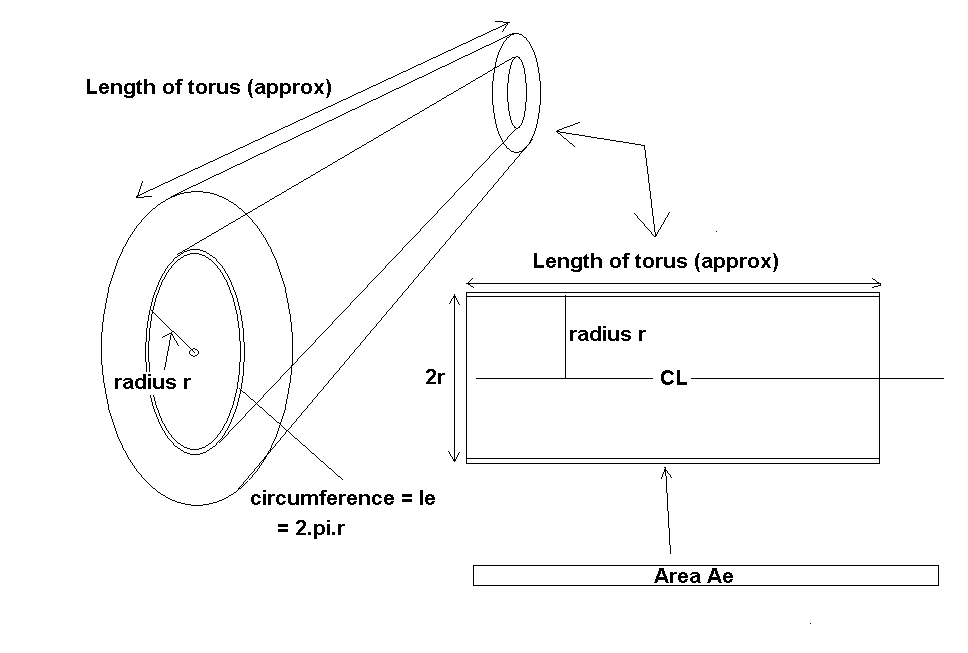
Imagine a circular wire loop (r = 50mm), the wire has an assumed diameter of zero, which is embedded in a torus shaped iron core with a circular cross-section of R = 10mm.

A current in that loop would cause a circular magnetic field around the wire. Is there any possibility to calculate the reluctance of that core?

I'm looking for a solution for weeks now, without any success. A solution for harmonic currents is desired, but I would even be happy for a DC solution.

---

CONTEXT

to explain what this is all about.

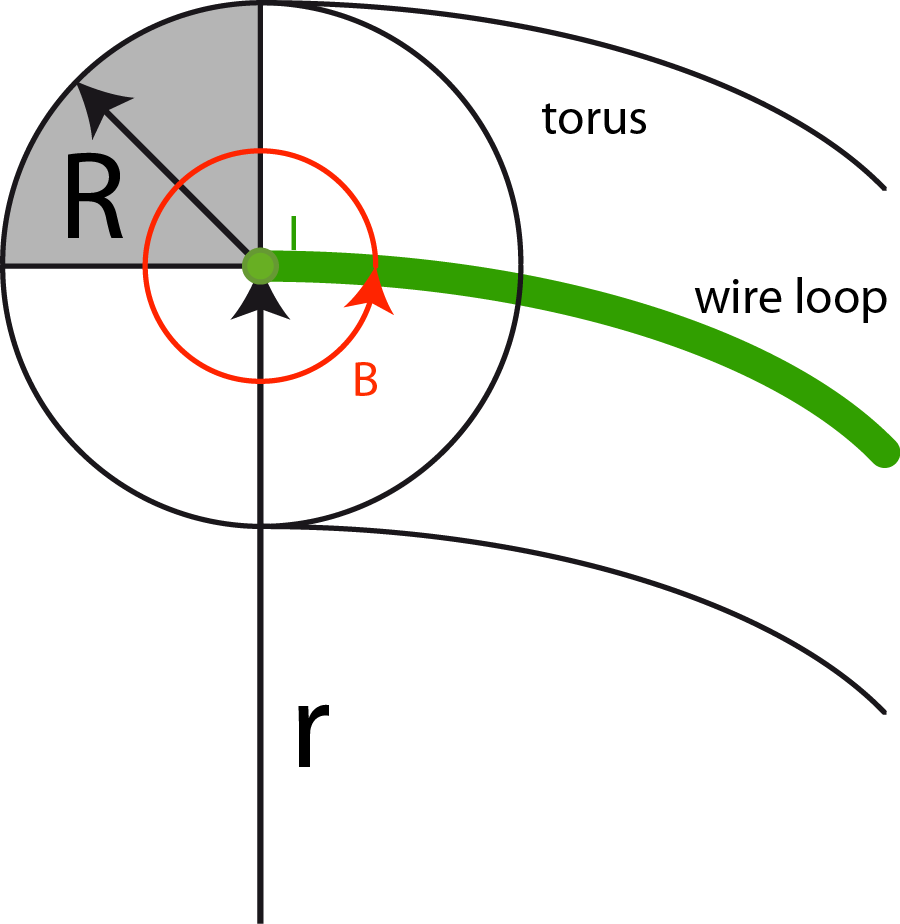
My real geometry looks as following:

A torodial coil surrounded by a core with a cross section of a rounded rectangle. So I'm interested in the reluctance of the greyish part (and the other corners). If you put all corners together you'd get the mentioned torus. The green lines are the magnetic flux, the rectangle in the middle the torodial coil.

For high frequencies and/or high conductive and/or high permeable materials the influence of the corners is negligible, for my case unfortunately not.

I'd guess there is no analytic solution, but any idea which could get me close to it, would help.

Thank you!

---

---

Attempted solution

Preface

If one wants to calculate the permeance $P$ of a rectangular bar:

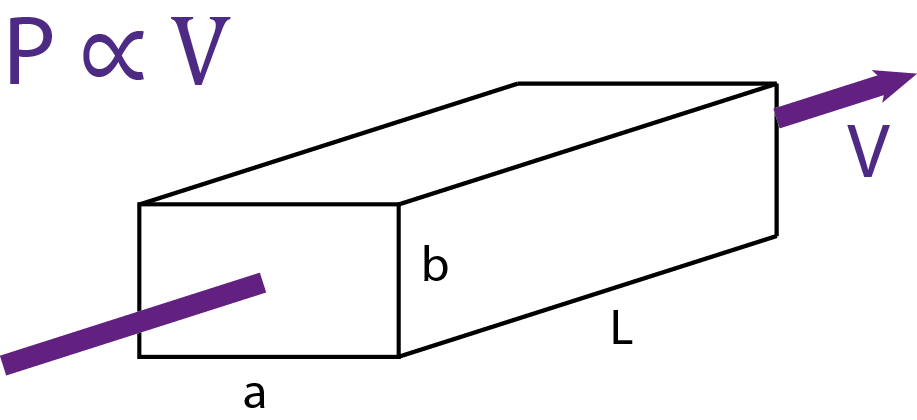
it is an easy task:

$$P = \frac{\mu a b}{L} ~~~~ \rightarrow ~~~~ P\propto ab ~~~~and~~~~ P\propto\frac{1}{L}$$

where $\mu$ is the material constant. (Permeability)

But my geometry is a torus with just a quarter of its circular cross section and the field $V$ passes through it parallel to the circumference of the (full) cross section:

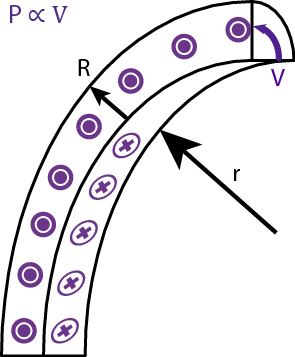
How can I calculate the permeance of this geometry, when there are the same proportional relations as above?

---

Attempted solution

I divide my geometry in $N$ hollow toruses with constant wall thickness $\Delta R$ and medium length element $\Delta L$, so the field passes an area of $\Delta A$:

A little piece of the radius $R$ is $\Delta R = \frac{R}{N}$. Now one can calculate:

$$\Delta P_{n} = \frac{\mu \Delta A_n}{\Delta L_n} $$

with $$ \Delta A_n = \pi \bigg( (r+(n+1) \Delta R)^2-(r+n \Delta R)^2\bigg) $$ (Consider the full torus circumference, not just a quarter as displayed)

and $$ \Delta L_n = \frac{\pi}{2} (2n+1) \frac{\Delta R}{2} $$ (but quarter cross section!)

follows:

$$P = \sum^{N-1}_{n=0} \Delta P_{n} = \mu\sum^{N-1}_{n=0} \frac{\pi(2r\Delta R+(2n+1)(\Delta R)^2)}{\frac{\pi}{2}(2n+1)(\frac{\Delta R}{2})}~~~~~~~~~~~~~~~~~~~~~~~~~$$

$$= 4\mu\sum^{N-1}_{n=0} \frac{2r\Delta R+(2n+1)(\Delta R)^2}{(2n+1)(\Delta R)} $$

$$= 4\mu\sum^{N-1}_{n=0} \Bigg( \frac{2r}{(2n+1)} + \Delta R \Bigg)~~~~~~~~~~ $$

$$= 4\mu \Bigg( R + 2r \sum^{N-1}_{n=0} \frac{1}{(2n+1)} \Bigg)~~~~~~~~~~ $$

And this series does not converge for $N\rightarrow\infty$. Which is physically seen not possible, so there must be a problem with the math. Do you see what I'm missing?

Answer 1

Reluctance = $\dfrac{l_e}{\mu A_e}$ where.....

mu is the absolute permeability of the material, $\mu_0 \mu_r $

$l_e$ is the circumference of a circle at a radius r and $A_e$ is a small cross sectional area.

The circle I refer to only relates to the cross section of the torus and r is the radius from the centre (where the wire is). All these reluctances are in parallel so it might be easier to integrate the inverse of reluctance from zero radius to the edge of the torus.

$A_e$ needs to be visualized as containing one side dimension that is the total length of the torus as if it were stretched out flat and this is partially dependent on radius (above) and the inner and outer radii of the torus.

Good luck.

EDITED to better show what I mean: -