What was the density of the universe when it was only the size of our solar system? Did it approach neutron star density? Is it physically correct to even ask such a question?
-
4$\begingroup$ The universe may well be infinite in size, in which case it was always infinite in size even at the moment of the Big Bang. So there was no point when the universe was the size of the Solar System. $\endgroup$– John RennieCommented Jul 15, 2014 at 5:05
-
3$\begingroup$ There was the old "one universe per solar system" unit, which is recursive. There should be a new definition in the renormalization of SI. $\endgroup$– C. Towne SpringerCommented Jul 15, 2014 at 5:29
-
$\begingroup$ @JohnRennie Maybe he is thinking on the size of the observable universe? $\endgroup$– peterhCommented Jul 15, 2014 at 8:28
-
$\begingroup$ Probably. ..but I am not sure what the difference is between the 'universe' and the 'observable universe' in such discussions. $\endgroup$– ShooksterCommented Jul 16, 2014 at 3:38
-
$\begingroup$ The observable universe is everything we can see (by any means, Hubble, radio-telescopes, etc) - anything within 13.5 billion years time. Due to expansion, the size is larger than that now, but we have a pretty good idea of how big and a fairly good idea how massive the observable universe is. The entire universe - that's a much bigger question and there's at least a pretty good chance it's infinite. $\endgroup$– userLTKCommented Jun 10, 2015 at 9:14
3 Answers
Your question can't be answered because the qualifier when it was only the size of our solar system is meaningless. The size of the universe is a rather vague concept. The universe may well be infinite (it's unlikely we'll ever know for sure) in which case it was always infinite and it doesn't have a size. You could ask about the size of the observable universe i.e. the bit we can see, but even that is tricky. We can see about 13 billion light years, but the bits we see 13 billion light years away we're seeing as they were 13 billion years ago. The current distance of those bits is about 46 billion light years. So is the size (radius) of the observable universe 13 billion light years or 46 billion light years?
But I think we can address the spirit of your question if not the exact text. There is a well defined measure of size that works even for an infinite universe called the scale factor. If you take two points in the universe then the distance between those points changes with time according to the equation:
$$ d = a(t) d_0 $$
where $a(t)$ is called the scale factor and $d_0$ is a constant. It's conventional to define $a(t)$ to be $1$ at the current time, in which case $d_0$ is the current distance. The average density of the universe is then given by:
$$ \rho(t) = \frac{\rho_0}{a^3(t)} $$
where $\rho_0$ is the current average density. We can use this equation to work out at what time the density of the universe was equal to that of a neutron star.
The scale factor is calculated by solving Einstein's equation for a homogenous isotropic universe, and the result is the FLRW metric. As you would probably expect for anything related to general relativity this doesn't give a simple answer. As Pulsar explains here the scale factor is given by:
$$ \begin{align} t(a) &= \int_0^a \frac{a'\,\text{d}a'}{a'^2H(a')}\\ &= \frac{1}{H_0}\int_0^a \frac{a'\,\text{d}a'}{\sqrt{\Omega_{R,0} + \Omega_{M,0}\,a' + \Omega_{K,0}\,a'^2 + \Omega_{\Lambda,0}\,a'^4}}, \end{align} $$
You have to calculate $a(t)$ by numerically integrating this expression, but this isn't as hard as it looks and I did it in Excel (see link here) to get:
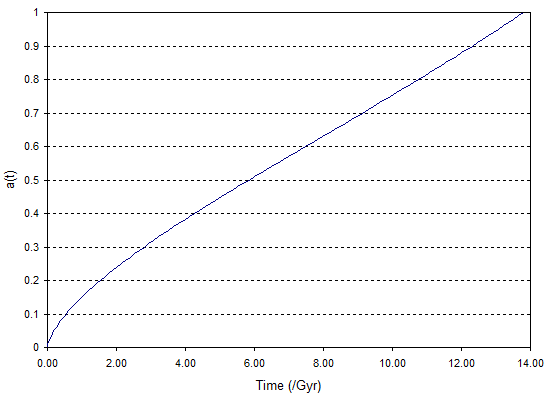
There are some interesting features to this. Up to about 6Gyrs after the Big Bang the expansion was slowing as you'd expect because the mutual gravity of all the matter is decelerating it. However more recently dark energy has been causing the expansion to accelerate, and you can just see the line beginning to curve upwards. This curvature will get more pronounced in the next few tens of billions of years.
Anyhow, back to your question. The current radius of the observable universe is about 46 billion light years, and the radius of Neptune's orbit is about $4.5 \times 10^{12}$ m, so the ratio of the two is about $9.7 \times 10^{13}$. The current density (including dark matter and dark energy) is about 5 hydrogen atoms per cubic metre, so the density when the observable universe was the size of Neptune's orbit was about $5 \times 10^{42}$ atoms of hydrogen per cubic metre. This is about $7.5 \times 10^{15}$ kg/m$^3$. The density of a neutron star is around $5 \times 10^{17}$ kg/m$^3$ so actually the density of the universe at this time wasn't that far off neutron star densities.
We can use our calculation of $a(t)$ to work out what time the universe had this density, and it comes out to be about $10^{-25}$ seconds. However I'd be cautious about this number because the calculated time lies within the range that the electroweak symmetry breaking was happening, and this may include new factors that influence the scale factor.
-
$\begingroup$ I added a link to your excel spreadsheet: on first reading my reaction was "lemme get this straight: you used WHAT?". On looking at the method though, and thinking about the kind of integrand we have, one realizes trapezoid integration is quite reasonable as long as one's stepsize is small enough compared with $\Omega_{R,\,0}$ $\endgroup$ Commented Jun 10, 2015 at 9:08
-
$\begingroup$ @WetSavannaAnimalakaRodVance: I can't remember putting the spreadsheet on Google Docs. Where did you find the link? Did I put it somewhere in my question that I now can't find or did you get from somewhere else? Oddly, I now can't find it in my Google drive account. $\endgroup$ Commented Jun 10, 2015 at 9:13
-
$\begingroup$ You linked to it in another answer which was referred, along with this one, from a third answer of yours. I can't find it now! $\endgroup$ Commented Jun 10, 2015 at 9:15
-
$\begingroup$ Here it is: physics.stackexchange.com/a/136057/26076 $\endgroup$ Commented Jun 10, 2015 at 9:17
I think, John Rennie's answer is far far better than anything I can do, but I'm going to give you the "universe for dummies" answer anyway.
Mass of the observable Universe: 10^53 KG
Density of the observable Universe: 9.9×10−30 g (equivalent to 6 protons per cubic meter of space - not very dense.)
Source (Wiki): http://en.wikipedia.org/wiki/Observable_universe
Density of a Neutron Star: 3.7×1017 to 5.9×1017 kg/m3
Source (Wiki): http://en.wikipedia.org/wiki/Neutron_star
So, 10^53 KG / 5.9x10^17 kg/m3 = 1.7 x 10^35 cubic meters, or about 550 billion meters on a side (square) or about 680 billion meters diameter. That's 340 million KM radius - a fair bit past the orbit of Mars, less than half the orbit of Jupiter.
That's less than I thought it would have been, but there's your answer, for the observable universe anyway. The answer is no - not when the observable universe was the size of the solar system.
Was the very young/early universe ever denser than a Neutron Star, you know, when the observable universe was even smaller? - probobly, since they say the (observable) universe used to be the size of a baseball , but that occurred early in the expansion phase and expansion moved faster than gravity (which can only pull things at the speed of light) - so the that much mass at that density wasn't a problem like it would be in conventional physics (it would form a black hole).
A lot of this probobly happened before the Higgs field formed, so there might have been no mass when the universe was that "dense" - or hot is perhaps a better term.
(Corrections on my universe for dummies version are welcome)
If you haven't read it, I've enjoyed the Wiki writeup on the chronology of the Universe, which doesn't talk much about size, but otherwise it's related: http://en.wikipedia.org/wiki/Chronology_of_the_universe
One of the most troublesome of the anomalies with which the Big Bang is marred, is that it is not possible to physically describe the Big Bang process in an infinite universe. The reason for this impossibility is that it is equally impossible to speak of the concept of concurrency everywhere in such a universe. Yes, in such a universe even the concept of "everywhere" has no other meaning than a metaphysical one. And even if the universe is assumed to be finite, but very large, we have to deal with the problem that all around us, just beyond the event horizon, there would seem to be a continuous Big Bang still in full swing, which certainly would have had such an impact on the event horizon that it would be displaced and moved billions of light years further out in space. The COBE and WMAP satellites (and more recently Planck) found that the back-ground radiation in the universe follows a black body spectrum with a temperature of 2.73 K. With a Big Bang in full career next door, such a low back-ground radiation temperature would certainly be impossible.
Bo Widarsson Soderquist
-
2$\begingroup$ The background radiation has a "temperature" of 2.73 K because of the expansion. As the space expands, so does the wavelength of the photons travelling in it - they are "red-shifted". When the "primordial photons" were still young, they were incredibly "hot" - they just lost most of that already. $\endgroup$– LuaanCommented Sep 24, 2014 at 7:31
