Your question can't be answered because the qualifier when it was only the size of our solar system is meaningless. The size of the universe is a rather vague concept. The universe may well be infinite (it's unlikely we'll ever know for sure) in which case it was always infinite and it doesn't have a size. You could ask about the size of the observable universe i.e. the bit we can see, but even that is tricky. We can see about 13 billion light years, but the bits we see 13 billion light years away we're seeing as they were 13 billion years ago. The current distance of those bits is about 46 billion light years. So is the size (radius) of the observable universe 13 billion light years or 46 billion light years?
But I think we can address the spirit of your question if not the exact text. There is a well defined measure of size that works even for an infinite universe called the scale factor. If you take two points in the universe then the distance between those points changes with time according to the equation:
$$ d = a(t) d_0 $$
where $a(t)$ is called the scale factor and $d_0$ is a constant. It's conventional to define $a(t)$ to be $1$ at the current time, in which case $d_0$ is the current distance. The average density of the universe is then given by:
$$ \rho(t) = \frac{\rho_0}{a^3(t)} $$
where $\rho_0$ is the current average density. We can use this equation to work out at what time the density of the universe was equal to that of a neutron star.
The scale factor is calculated by solving Einstein's equation for a homogenous isotropic universe, and the result is the FLRW metric. As you would probably expect for anything related to general relativity this doesn't give a simple answer. As Pulsar explains herePulsar explains here the scale factor is given by:
$$ \begin{align} t(a) &= \int_0^a \frac{a'\,\text{d}a'}{a'^2H(a')}\\ &= \frac{1}{H_0}\int_0^a \frac{a'\,\text{d}a'}{\sqrt{\Omega_{R,0} + \Omega_{M,0}\,a' + \Omega_{K,0}\,a'^2 + \Omega_{\Lambda,0}\,a'^4}}, \end{align} $$
You have to calculate $a(t)$ by numerically integrating this expression, but this isn't as hard as it looks and I did it in Excel (see link here) to get:
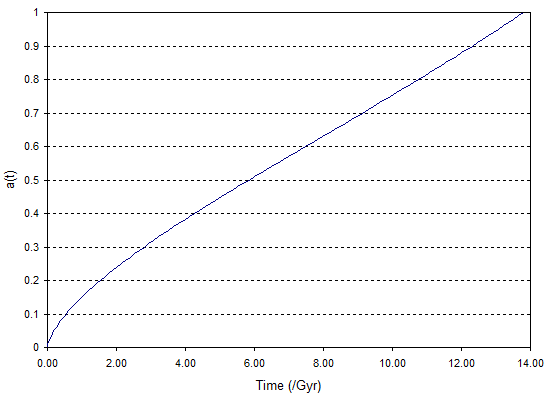
There are some interesting features to this. Up to about 6Gyrs after the Big Bang the expansion was slowing as you'd expect because the mutual gravity of all the matter is decelerating it. However more recently dark energy has been causing the expansion to accelerate, and you can just see the line beginning to curve upwards. This curvature will get more pronounced in the next few tens of billions of years.
Anyhow, back to your question. The current radius of the observable universe is about 46 billion light years, and the radius of Neptune's orbit is about $4.5 \times 10^{12}$ m, so the ratio of the two is about $9.7 \times 10^{13}$. The current density (including dark matter and dark energy) is about 5 hydrogen atoms per cubic metre, so the density when the observable universe was the size of Neptune's orbit was about $5 \times 10^{42}$ atoms of hydrogen per cubic metre. This is about $7.5 \times 10^{15}$ kg/m$^3$. The density of a neutron star is around $5 \times 10^{17}$ kg/m$^3$ so actually the density of the universe at this time wasn't that far off neutron star densities.
We can use our calculation of $a(t)$ to work out what time the universe had this density, and it comes out to be about $10^{-25}$ seconds. However I'd be cautious about this number because the calculated time lies within the range that the electroweak symmetry breaking was happening, and this may include new factors that influence the scale factor.
