Intuitive explanation
Suppose you drill two, perpendicular holes through the center of the Earth. You drop an object through one, then drop an object through the other at precisely the time the first object passes through the center.
What you have now are two objects oscillating in just one dimension, but they do so in quadrature. That is, if we were to plot the altitude of each object, one would be something like $\sin(t)$ and the other would be $\cos(t)$.
Now consider the motion of a circular orbit, but think about the left-right movement and the up-down movement separately. You will see it is doing the same thing as your two objects falling through the center of the Earth, but it is doing them simultaneously.
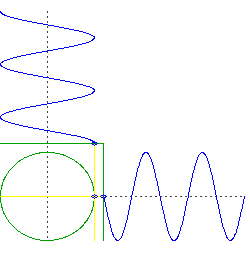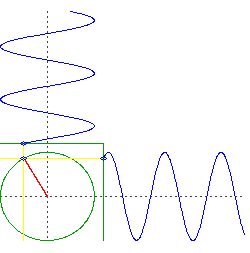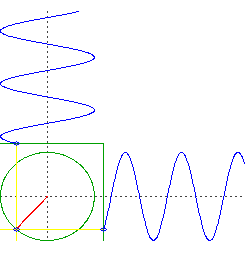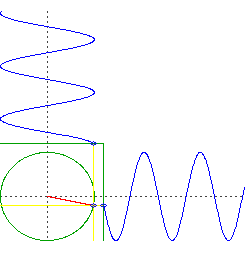
image source
caveat: an important assumption here is an Earth of uniform density and perfect spherical symmetry, and a frictionless orbit right at the surface. Of course all those things are significant deviations from reality.
Mathematical proof
Let's consider just the vertical acceleration of two points, one inside the planet and another on the surface, at equal vertical distance ($h$) from the planet's center:
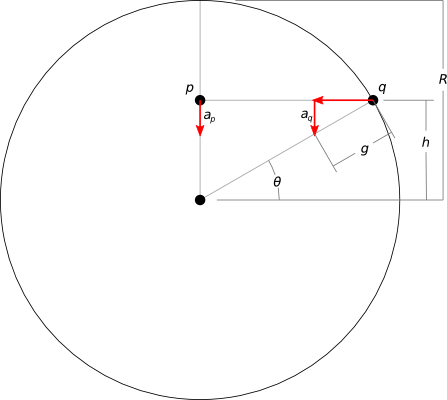
- $R$ is the radius of the planet
- $g$ is the gravitational acceleration at the surface
- $a_p$ and $a_q$ are just the vertical components of the acceleration on each point
If we can demonstrate that these vertical accelerations are equal, then we demonstrate that the differing horizontal positions have no relevance to the vertical motion of the points. Then we can free ourselves to think of vertical and horizontal motion independently, as in the intuitive explanation.
Calculating $a_q$ is simple trigonometry. It's at the surface, so the magnitude of its acceleration must be $g$. Just the vertical component is simply:
$$ a_q = g (\sin \theta) $$
If you have worked through the "dropping an object through a tunnel in Earth" problem, then you already know that in the case of $p$, its acceleration linearly decreases with its distance from the center of the planet (this is why the "uniform density" assumption is important):
$$ a_p = g \frac{h}{R} $$
$h$ is equal for our two points, and finding it is again simple trigonometry:
$$ h = R (\sin \theta) $$
So:
$$ \require{cancel}
a_p = g \frac{\cancel{R} (\sin \theta)}{\cancel{R}} \\
a_p = g (\sin \theta) = a_q $$
Q.E.D.
This also gives some insight to an unfortunate consequence: this method can be applied only to orbits on or inside the surface of the planet. Outside of the planet, $p$ no longer experiences an acceleration proportional to the distance from the center of mass ($a_p \propto h$), but instead proportional to the inverse square of distance ($a_p \propto 1/h^2$), according to Newton's law of universal gravitation.


