3
$\begingroup$

Using the equation for Kepler's constant for Earth $K$:
$$K = \frac{GM}{4\pi^2}$$ $$K = 1.01 \times 10^{13} \;\rm m^3/s^2$$

Finding time ($r = 6.4 \times 10^{6} \;\rm m$ (radius of Earth)):

$$K = \frac{r^3}{T^2}$$ $$T = \sqrt{\frac{r^3}{K}}$$ $$T = 5095 \;\rm s = 85\; minutes$$

So 85 minutes is approximately the minimum time for a satellite to orbit the Earth. Why can't it just go faster to take less time? Would that mean it would fall out of orbit?

Improve this question
$\endgroup$
1
  • $\begingroup$ your math was quite a mess. First, please make sure to indicate the units on all quantities that have units (your value for $K$ was missing them). Also, be careful to keep your notation consistent (you switched from $K$ to $C$ halfway through your question). Finally, try to use the MathJax markup to format the math - if you click edit on your post you can see what I did to get the nice formatting that now appears. $\endgroup$
    – Kyle Oman
    Commented Jul 11, 2014 at 17:04

3 Answers 3

Reset to default
6
$\begingroup$

Following the same orbit at greater velocity would mean that the satellite has greater acceleration (its velocity is changing at a greater rate than if it follows the same route more slowly). But the only force acting on it is earth's gravity, which creates a particular acceleration at any given altitude above the earth. It can't go any faster or slower unless it can apply another force.

So fuel permitting, a satellite could circle the earth faster, by applying additional force directed towards the earth. It could go slower with a force directed away from the earth. This isn't an "orbit" in the usual sense, since it's powered. If you want to travel using only gravity, then you go at the speed gravity takes you.

Improve this answer
$\endgroup$
2
$\begingroup$

A satellite stays in a circular orbit if centripetal acceleration needed to move in a circle is the acceleration of gravity.

$Gm_e/r^2 = v^2/r$

or

$r = Gm_e/v^2$

Faster speeds are for lower orbits. The lowest orbits are just above the atmosphere. These are also the shortest distance, and therefore shortest time.

Improve this answer
$\endgroup$
1
$\begingroup$

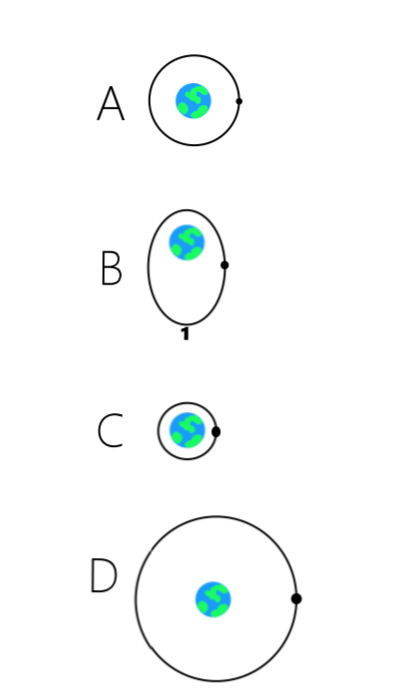
In figure A the satellite is orbiting in a circular orbit. By the satellite increasing speed it would turn the orbit into a more egg shaped orbit as shown in B. This would rather increase the time for orbit revolution rather than decreasing it

When the satellite is approaching B.1 it's velocity will begin to slow down as earths gravity begins to pull it. Once it reaches 1 the speed will begin increasing until the satellite reaches periapsis (nearest point in orbit to earth) followed by the satellites speed then decreasing until it reaches apoapsis (farthest point in orbit which is b.1)

You can decrease the time of orbit for the satellite by making its orbit closer to earth. This increases the velocity of the satellite. But when the satellite is closer to the atmosphere it is susceptible to more upper atmospheric drag which is not suitable as this would eventually slow the satellite enough to enter the atmosphere and burn up.

Having a larger orbit as shown in D would cause the satellite's velocity to decrease as well as the orbit revolution time to increase.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ Your picture B appears to show the Earth at the geometric center of the ellipse. But really, the Earth would be at one of the foci. $\endgroup$
    – Solomon Slow
    Commented May 25, 2016 at 17:18
  • 1
    $\begingroup$ Fixed the image @jameslarge $\endgroup$
    – mateos
    Commented May 25, 2016 at 17:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.