Lorentz length contractions states that the length of any moving object gets divided by the Lorentz factor equal to the Lorentz factor for that object (always $\geq 1$), equal to $$ \gamma=\frac{1}{\sqrt { 1-\frac { { v }^{ 2 } }{ { c }^{ 2 } } } } $$ However, in massless particles $v=c$, so the Lorentz factor becomes $\infty$, meaning that an object traveling at $c$ will have $0$ length. However, photons and obviously all forms of electromagnetic waves move at c when traveling through a vacuum, such as from a space shuttle to a space station or back to Earth. Does this mean that photons have no length? How does this affect wavelength?
6 Answers
Let's think clearly about length contraction.
In the frame of reference in which an object is at rest, the measured length of an object is $L_0$.
In a frame of reference in which the object is moving with velocity $v$ parallel to the length of the object, the measured length of the object is
$$L'(v) = L_0\sqrt{1 - \frac{v^2}{c^2}} $$
Now, in your question, you wrote:
However, in massless particles $v=c$, so the Lorentz factor becomes $\infty$, meaning that an object traveling at $c$ will have $0$ length.
The problem here is that, for a massless particle, there is no frame of reference in which the object is at rest; a massless particle has speed c in all frames of reference.
Thus, the proper length, $L_0$, doesn't exist so the formula above is not valid for massless particles.
This shouldn't be too surprising since the Lorentz transformations, from which the length contraction formula above is derived, doesn't exist for $v = c$ since the Lorentz factor
$$\gamma = \frac{1}{\sqrt{1 - \frac{v^2}{c^2}}}$$
is undefined for $v=c$.
This brings us back to the question of whether or not a massless object can have extent along the direction of motion since we cannot use length contraction, as you have, to reason that it can't.
I had intended to address the open question above as an update after spending some time thinking about it while mowing. In the meantime, another answer has been given that approaches this question in essentially the same manner. For what it's worth, here's the addendum.
Consider the question: Given the equation for the world line of a uniformly moving particle in some frame of reference, what is the equation in another relatively moving frame of reference?
Working in 1-D and with standard configuration, assume a particle's world line in the unprimed frame of reference is given by
$$x(t) = ut + x_0$$
where $u$ is the velocity of the particle and $x_0$ is the position when $t=0$.
In the primed frame of reference, which has velocity $v$ in the unprimed frame, the particle's world line is given by
$$x'(t') = u't' + \frac{x_0}{\gamma_v(1 - \frac{uv}{c^2})} $$
where
$$u' = \frac{u - v}{1 - \frac{uv}{c^2}}$$
Now, assume we have two particles with world lines given by
$$x_1(t) = ut$$
$$x_2(t) = ut + d$$
Clearly, we have
$$x_2(t) - x_1(t) = d$$
$$x'_2(t') - x'_1(t') = \frac{d}{\gamma_v(1 - \frac{uv}{c^2})}$$
It's straightforward to show and, indeed, intuitive that, except for one special case, there is a maximum value when $v = u$
$$x'_2(t') - x'_1(t') = \frac{d}{\sqrt{1 - \frac{u^2}{c^2}}} = d_0 $$
The value $d_0$ has physical significance in that it is the separation of the world lines in the frame of reference in which the particles are at rest.
This is physically significant since, in this frame of reference only, the separation can be measured without requiring synchronized, spatially separated clocks. This separation, $d_0$ is thus an invariant, an objective quantity of physical significance.
In terms of $d_0$ and the speed $u$, we can write
$$d(u) = d_0 \sqrt{1 - \frac{u^2}{c^2}}$$
which is the familiar length contraction formula.
However, for the case that $|u| = c$ (the world lines are light-like), we cannot set $v = u$; the Lorentz factor is not defined for $v = c$.
For $u = c$, we have
$$x'_2(t') - x'_1(t') = \frac{d}{\gamma_v(1 - \frac{cv}{c^2})} = d\sqrt{\frac{1+\frac{v}{c}}{1- \frac{v}{c}}}$$
Thus, for the case that the particles are light-like, there is no maximum value of $d$; no $d_0$ that we can attach physical significance to.
-
2$\begingroup$ The term "rest length" is not really standard, and is a bad idea. Max Born showed long ago that the concept of a rigid body actually makes no sense in Special Relativity. $\endgroup$ Commented Jun 1, 2014 at 3:45
Lorentz' law only applies to physical objects, i.e. those with rest mass. Wavelength is a parameter -- as you probably know, particles with mass have a wavelength related to their momentum -- so it's not sensible to discuss wavelengths changing with speed for photons whose speed is always $c$ .
As an aside, I find it hard to conceive of a way to assign a "length" to a massless particle travelling at $c$ in the first place :-) .
-
1$\begingroup$ @CarlWithoft: "it's not sensible to discuss wavelengths changing with speed for photons whose speed is always c", I think the speed of photons changes depending upon the medium in which it is travelling, I think it is not constant. $\endgroup$– SensebeCommented May 31, 2014 at 1:59
-
1$\begingroup$ @Godparticle that is true, but it's not related to any kind of Lorentz transformation. But thanks for pointing that out so I don't lead anyone astray. $\endgroup$ Commented May 31, 2014 at 11:31
-
$\begingroup$ @CarlWithoft: I really appreciate your answer. Thank you for the response. $\endgroup$– SensebeCommented May 31, 2014 at 13:40
-
1$\begingroup$ Lorentz's law applies to empty space as well: it is kinematical, and has nothing to do with mass or matter or rigid bodies. $\endgroup$ Commented May 31, 2014 at 21:51
-
$\begingroup$ That wavelengths of a light ray change with the speed of the observer is the Doppler effect, and that is what is really the point here. The observer's speed can never be equal to the speed of light, but it can be very close, and then something similar to what the O.P. asks about does indeed happen. $\endgroup$ Commented Jun 1, 2014 at 3:39
There are several conceptual confusions here. Mass is irrelevant. This is purely a kinematical question, it does not depend on dynamics at all.
The difference between Lorentz's (and Fitzgerald's and Larmor's) approach to the Lorentz--Fitgerald contraction (and Larmor time dilation) on the one hand, and Einstein's revolutionary and conceptual breakthrough, the relativity principle, is precisely that Lorentz and the others thought of the contraction as somehow being a property of matter and dynamics and forces, it was a property of a material body. It was Einstein (and Poincaré) who realised that no, this is a property of space-time itself, of the geometry of space-time. It happens even for massless points not even connected to each other by a body at all.
So you could suppose the Universe is completely empty---no mass or energy, completely flat Minkowski space. For simplicity, suppose there is one spatial dimenion, $x$, and one time dimension, and the velocity of light is unity, and $ds^2 = dx^2 - dt^2.$ If one co-ordinate system has its origin moving at the speed of light with respect to the origin of another co-ordinate system, one of them must fail to be an inertial reference frame. They cannot be connected by a Lorentz transformation. This is a kinematical theorem which does not depend on what dynamical law of motion Nature employs: it only depends on the principle of relativity. (It is true in General Relativity also, but has no importance there because in GR we do not care about whether a co-ordinate system is inertial or not.) So we cannot use a Lorentz transformation to compare what is seen by an observer travelling along with a photon with whast is seen by another observer for whom that origin is moving at the speed of light.
But the O.P. is getting at something, it just needs to be slightly rephrased: two observers in relative motion to each other, each using an inertial frame, will see the shape of a photon differently---there will be a contraction or expansion---this is the Doppler effect. No one really knows what the shape of the photon is, but let us suppose that it has no mass and is perfectly spherical to observer 1 in the x,t reference frame (a sphere in one dimension is an interval) with unit diameter. I.e., suppose one edge of the photon is at (0,0) and the other edge at (1,0) in the x,t reference frame. If anyone wants to criticise the O.P. and naive ideas of spherical massless photons, just think of two points in space-time which are moving at the speed of light. There are no rigid bodies in the real world either, so we need not imagine anything in between.
For the sake of generality, though, we need not assume these two points are travelling at the speed of light: assume only that they are travelling at the same velocity $w$, i.e., at the same speed $|w|$ and in the same direction, so that for observer 1 they are always the same spatial distance apart.
This means that we are considering two world-lines: the world-line of (0,0) (point one) is $$x_1 = wt_1$$ and the world-line of the other point, which started at (1,0), is $$x_2 = wt_2 + 1.$$
Consider another reference frame x',t' travelling at velocity $v$ relative to the first one, so that the co-ordinates are connected by the usual Lorentz transformation, $$ x' = {x-vt\over\sqrt{1-v^2}}$$
$$ t' = {t-xv\over\sqrt{1-v^2}}.$$
The first world-line becomes $$ x'_1 = {wt_1-vt_1\over\sqrt{1-v^2}}$$ $$ t'_1 = {t_1-vwt_1\over\sqrt{1-v^2}}$$ where $t_1$ is merely a parameter. On eliminating this parameter, be obtain $$x_1' = {w-v\over 1-vw}t_1',$$ i.e., the apparent velocity has suffered a shift, which is not the Lorentz contraction at all. Of course. Unless $w=1$, in which case there has been no change (the speed of light is invariant under a Lorentz transformation).
But the O.P. asks about the change in the spatial separation between the two world-lines.
The second world-line is $$x'_2 = {wt_2+1-vt_2\over\sqrt{1-v^2}}$$ $$t'_2 = {t_2-v(wt_2+1)\over\sqrt{1-v^2}},$$ which will have the same slope but a different intercept: eliminating $t_2$ we proceed as follows. $$x'_2 = {t_2(w-v)\over\sqrt{1-v^2}} + {1\over\sqrt{1-v^2}}$$ $$t'_2 = {t_2(1-vw)\over\sqrt{1-v^2}} -{v\over\sqrt{1-v^2}},$$ and so $$x'_2 = {w-v\over 1-vw} t'_2 + {v{w-v\over 1-vw}\over\sqrt{1-v^2}} + {1\over\sqrt{1-v^2}}.$$
The second reference frame sees, at $t'_2 = 0$, the first point at (0,0) but the second point at $((v{w-v\over1-vw}+1)/\sqrt{1-v^2},0)$. Simplifying, since $v{w-v\over 1-vw} + 1 = {vw-w^2\over1-vw} + {1-vw\over1-vw} = {1-v^2\over 1-vw}$, and now dividing by $\sqrt{1-v^2}$, we obtain a simpler expression for point 2 at this time, $$ ({\sqrt{1-v^2}\over1-vw}, 0 ).$$ This is not the Lorentz contraction, it is the Doppler effect, unless $w=0$, which is the case considered by the Lorentz contraction, so we do get the Lorentz contraction as a special case of the Doppler effect: a separation in space of $\sqrt{1-v^2}$ between the two world lines in the second reference frame, instead of unit separation in space as seen by the first reference frame in which the points are motionless.
That is, the Doppler effect includes the Lorentz--Fitzgerald contraction as a special case.
But suppose $w=1$, as is the case with a photon. We then get a separation in space of $${\sqrt{1-v^2}\over1-v} = \sqrt{1+v\over1-v}.$$ Sometimes this is a contraction of the spherical photon, other times, an expansion. In reality, of course, it is impractical to observe the two diametrically opposite ends of a photon...so the practical application of this formula is for two wave crests of a wave travelling at the speed of light or the speed of sound. The apparent wavelength will expand or contract. But in theory, if the photon were a massless particle with a spherical shape in reference frame 1, it would have some other shape in reference frame 2. And by letting $v\rightarrow \pm 1$, one can see the contraction approach zero, and the expansion approach infinity.
-
1$\begingroup$ "No one really knows what the shape of the photon is" implies that there is an objective property shape possessed by a photon. But, there is no reference frame that is privileged; the photon has speed c in all frames and the 'shape' is different in each. This argues for the position that, whatever properties a massless particle has, shape is not one of them. $\endgroup$ Commented Jun 1, 2014 at 11:43
-
1$\begingroup$ Remarkably, while mowing, I was running through more or less the same separation of worldlines reasoning you've shown above and I had planned to write it up and add as an addendum to my answer (which I left somewhat open ended). $\endgroup$ Commented Jun 1, 2014 at 11:56
-
$\begingroup$ I think, he said harshly, you answered too quickly. After all, the Doppler effect is the real point of the O.P., and no one quicker than me really picked up on that. And B: this anthropomorphic teaching of beginning Relativity is precisely what produces the O.P.'s confusion, and so answers that still pander to the idea that rigid bodies and mass and physical observers are important, do not clear up those confusions. Admittedly, many teachers need to use some sort of crutch to start teaching. But when the confusions produced by those simplifications have arisen, $\endgroup$ Commented Jun 1, 2014 at 16:28
-
$\begingroup$ Then it is time to present the material without the misleading crutch. This is kinematics, not dynamics, and there are no rigid bodies. $\endgroup$ Commented Jun 1, 2014 at 16:29
-
$\begingroup$ What misleading crutch are you referring to Joseph? Is there something in particular about the phrase "the measured length of the object" that you object to? $\endgroup$ Commented Jun 1, 2014 at 16:53
Light has a frequency and a velocity (c). The wavelength, in appropriate units (i.e. with c=1), is simply the reciprocal of the frequency. It's the distance the light travels in the course of one cycle. It's not a measure of the size of an individual photon. A wavelength can be several meters, and obviously (or at least I hope it's obvious) this doesn't mean that the photons are each several meters long. However I don't know how you would measure the length of an individual photon, other than by measuring its wavelength, or what such a measurement might mean.
A photon would be contracted to length zero, in its own reference frame, if we could define such a thing. In that frame it would be stationary, and in going from A to B (for any A and any B) it would cover a distance of zero, taking zero time to do so. How it would oscillate through a possibly large number of cycles during an interval of time equal to zero seconds is left as an exercise to the reader.
-
1$\begingroup$ "A photon would be contracted to length zero, in its own reference frame." This is false, assuming you are boosting in the direction that the beam is traveling (to naively try to boost to the light's reference frame which you can't ever do), then the frequency (via doppler shift) would decrease and thus the wavelength would go to infinity not 0. $\endgroup$ Commented May 30, 2014 at 19:43
-
$\begingroup$ Measuring the length of a photon practically useless as far I know so far, but we do know things such as the diameter of atoms (not sure) the size of an electron, so maybe photons can have size. Aside from this, how can a photon have velocity $c$ in its own reference frame? Any object is stationary relative to itself. $\endgroup$– Arc676Commented May 31, 2014 at 3:32
-
$\begingroup$ whether it goes to infinity or zero depends on what direction you are moving. Now @Arc676: it is true that any object is stationary relative to itself. And in theory you could define a co-ordinate system with origin always located at a photon. And in that co-ordinate system, the photon is motionless. But it is not an inertial system, the laws of Physics would take a strange form in it (you would hae to use General Relativity to get them). And that system could not be connected by a Lorentz transformation to the system of an observer for whom the photon was travelling at the speed of light. $\endgroup$ Commented Jun 1, 2014 at 3:53
-
$\begingroup$ The usual analysis of the Doppler effect, which I developed in my own way above, analyses how different observers see the wave length of the same light wave: that wavelength being the spatial separation between two crests of the wave. But what people do not usuall notice is that the same analysis applies to any two points moving in the same direction and the same speed. So if the photon were a particle, and if it had a diameter, and if the two diametrically opposite points were moving in the same direction and at the same speed, the analysis applies without any changes. $\endgroup$ Commented Jun 1, 2014 at 16:33
Q: Does Light Experience Length Contraction?
"... so the Lorentz factor becomes ∞, meaning that an object traveling at c will have 0 length. However, photons and obviously all forms of electromagnetic waves move at c when traveling through a vacuum, ..."
An object traveling at the full speed of light (not just nearly so), that has mass (weighs something, anything), would require infinite energy to reach the speed of light; that would also take an extremely long time or it would be flattened by the acceleration, let alone contraction.
"Does this mean that photons have no length? How does this affect wavelength?"
A photon has no mass, no size; therefore no height, width or length.
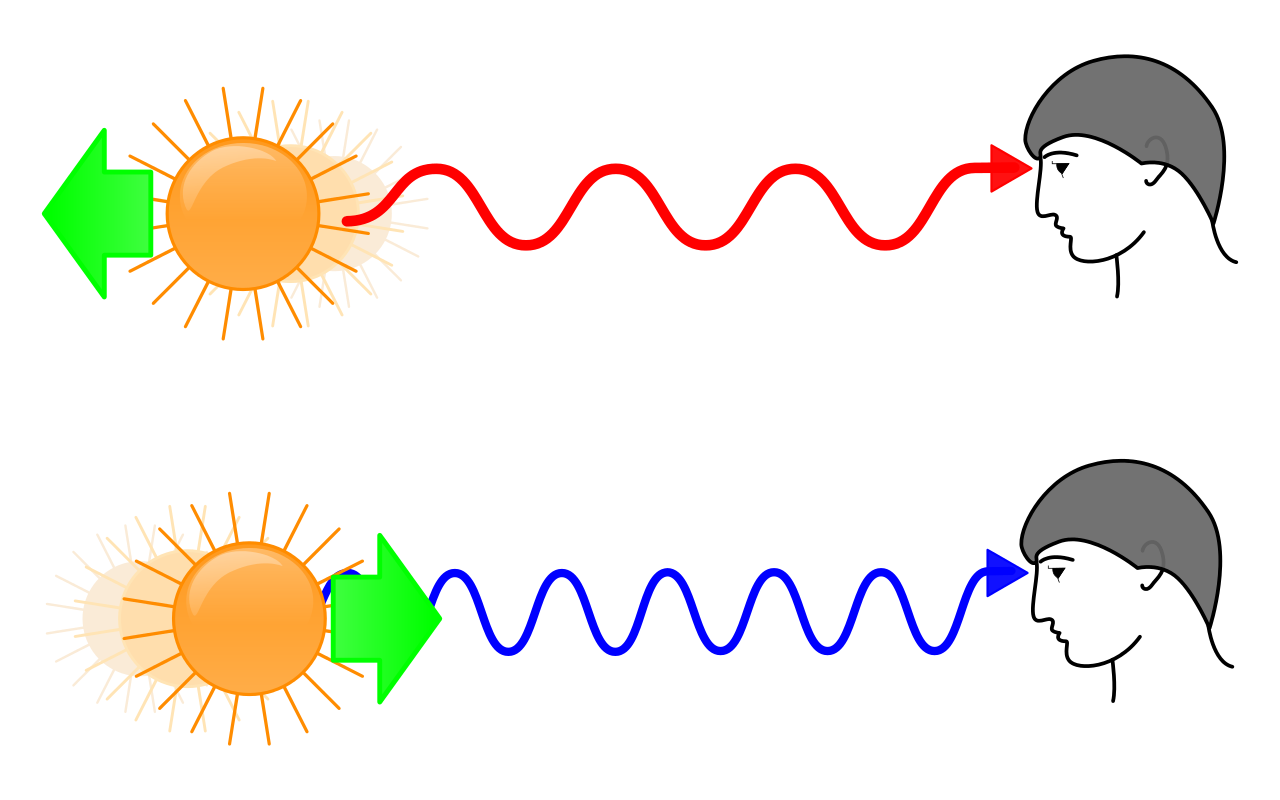
The speed of the photon's emitter (and other factors not speed related) that determine it's frequency, it's wavelength. An object moving away from you becomes red shifted, an object moving towards you gains blueshift.
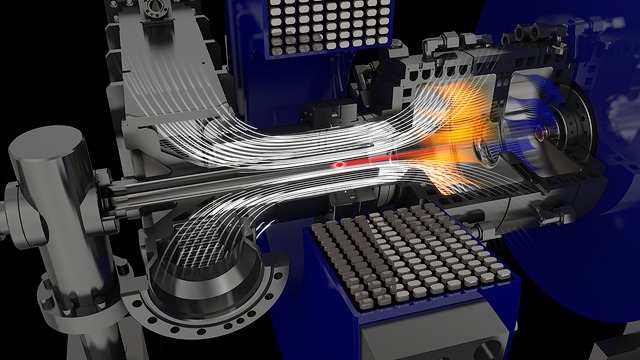
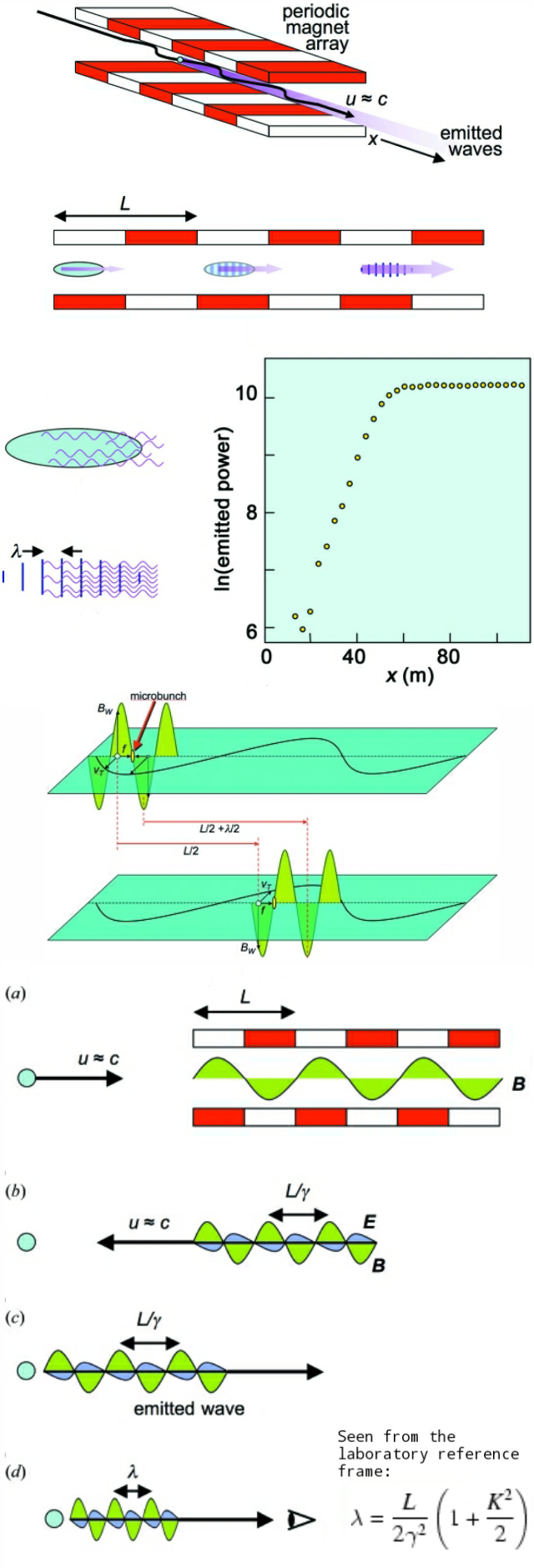
Instead of giving a mathematical answer I'll provide an example using a free-electron laser (FEL); a laser whose lasing medium consists of very-high-speed electrons moving freely through a magnetic structure, hence the term free electron.
A FEL is particularly useful as an example because it uses electrons (which have weight and mass) to create photons (which have no weight or mass), once the electrons have done as much work as is possible (saturation) they are dumped out of the beam.
Since the electrons have weight they can't pass the photons that they create because they can't be accelerated to light speed (though they come close to it in a FEL).
Beam Creation
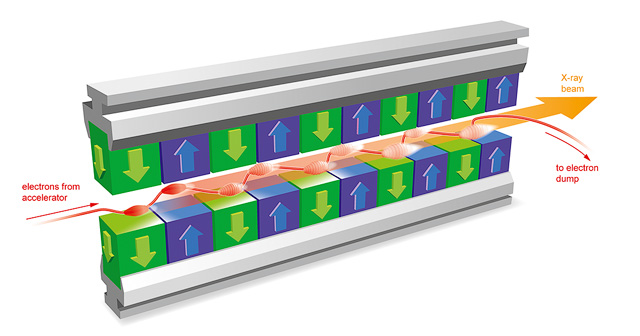
To create a FEL, a beam of electrons is accelerated to almost the speed of light. The beam passes through a periodic arrangement of magnets with alternating poles across the beam path, which creates a side to side magnetic field. The direction of the beam is called the longitudinal direction, while the direction across the beam path is called transverse. This array of magnets is called an undulator or a wiggler, because due to the Lorentz force of the field it forces the electrons in the beam to wiggle transversely, traveling along a sinusoidal path about the axis of the undulator.
The transverse acceleration of the electrons across this path results in the release of photons (synchrotron radiation), which are monochromatic but still incoherent, because the electromagnetic waves from randomly distributed electrons interfere constructively and destructively in time. The resulting radiation power scales linearly with the number of electrons.
Mirrors at each end of the undulator create an optical cavity, causing the radiation to form standing waves, or alternately an external excitation laser is provided. The synchrotron radiation becomes sufficiently strong that the transverse electric field of the radiation beam interacts with the transverse electron current created by the sinusoidal wiggling motion, causing some electrons to gain and others to lose energy to the optical field via the ponderomotive force.
This energy modulation evolves into electron density (current) modulations with a period of one optical wavelength. The electrons are thus longitudinally clumped into microbunches, separated by one optical wavelength along the axis. Whereas an undulator alone would cause the electrons to radiate independently (incoherently), the radiation emitted by the bunched electrons is in phase, and the fields add together coherently.
The radiation intensity grows, causing additional microbunching of the electrons, which continue to radiate in phase with each other. This process continues until the electrons are completely microbunched and the radiation reaches a saturated power several orders of magnitude higher than that of the undulator radiation.
The wavelength of the radiation emitted can be readily tuned by adjusting the energy of the electron beam or the magnetic-field strength of the undulators.
FELs are relativistic machines. The wavelength of the emitted radiation, λr, is given by
$${\lambda _{r}={\frac {\lambda _{u}}{2\gamma ^{2}}}\left(1+{\frac {K^{2}}{2}}\right)},$$
or when the wiggler strength parameter K, discussed below, is small
$$\lambda _{r}\propto {\frac {\lambda _{u}}{2\gamma ^{2}}},$$
where λ$_u$ is the undulator wavelength (the spatial period of the magnetic field), $\gamma$ is the relativistic Lorentz factor and the proportionality constant depends on the undulator geometry and is of the order of 1.
This formula can be understood as a combination of two relativistic effects. Imagine you are sitting on an electron passing through the undulator. Due to Lorentz contraction the undulator is shortened by a $\gamma$ factor and the electron experiences much shorter undulator wavelength λ$_u/\gamma$.
However, the radiation emitted at this wavelength is observed in the laboratory frame of reference and the relativistic Doppler effect brings the second $\gamma$ factor to the above formula. Rigorous derivation from Maxwell's equations gives the divisor of 2 and the proportionality constant. In an X-ray FEL the typical undulator wavelength of 1 cm is transformed to X-ray wavelengths on the order of 1 nm by $\gamma$ ≈ 2000, i.e. the electrons have to travel with the speed of 0.9999998c.
Wiggler Strength Parameter K
K, a dimensionless parameter, tells the wiggler strength as the relationship between the length of a period and the radius of bend,
$${\displaystyle K={\frac {\gamma \lambda _{u}}{2\pi \rho }}={\frac {eB_{0}\lambda _{u}}{2\pi m_{e}c}}}$$
where $\rho$ is the bending radius, B$_0$ is the applied magnetic field and m$_e$ the electron mass.
Expressed in practical units, the dimensionless undulator parameter is $${\displaystyle K=0.934\cdot B_{0}\,{\text{[T]}}\cdot \lambda _{u}\,{\text{[cm]}}}$$.
Quantum Effects In most cases, the theory of classical electromagnetism adequately accounts for the behavior of free electron lasers.
For sufficiently short wavelengths, quantum effects of electron recoil and shot noise may have to be considered
How it works https://www.xfel.eu/facility/overview/how_it_works/index_eng.html
To generate the X-ray flashes, bunches of electrons are first accelerated to high energies and then directed through special arrangements of magnets. Electrons are first brought to high energies in a superconducting accelerator. They then fly on a slalom course through a special arrangement of magnets (called an "undulator"), in which they emit laserlike flashes of radiation.
There's a virtual tour here: http://winweb.desy.de/pr/Virtueller_Rundgang/xfel/index.html
First the electron bunches are generated by knocking the particles out of a piece of metal using a conventional laser.
Next the electrons are accelerated in special cavities, the so-called resonators. In these resonators, an oscillating microwave transfers its energy to the electrons.
Buncher Video: http://winweb.desy.de/pr/Virtueller_Rundgang/xfel/assets/beschleuniger.mp4
Finally the accelerated electrons race through so-called undulators, periodic arrangements of magnets that force the electrons onto a tight slalom course. In the process, each individual electron emits X-ray radiation that amplifies more and more.
This amplification process is induced by the interaction of the X-ray radiation with the electrons: Because the radiation is faster than the electrons speeding along their slalom path, the radiation overtakes the electrons flying ahead and interacts with them along the way, accelerating some of them and slowing others down.
As a result, the electrons gradually organize themselves into a multitude of thin disks. The key property of this process is the fact that all of the electrons in a given disk emit their light “in sync.” This produces extremely short and intense X-ray flashes with the properties of laser light.
Read "A simplified description of X-ray free-electron lasers" for a layperson's explanation.
Why are the emitted wavelengths in the X-ray range?
Relativity provides the answer.
(a) The relativistic electron approaches the periodic B-field of the undulator.
(b) In the electron reference frame the undulator period L is Lorentz-contracted to L/γ and the B-field is accompanied by a transverse E-field perpendicular to it: the two fields resemble an electromagnetic wave.
(c) This wave stimulates the electron to oscillate and emit waves of equal wavelength.
(d) The (relativistic) Doppler effect further reduces the wavelength in the laboratory frame, bringing it to the X-ray range.
You can see how closely the electron follows the photon it produces but due to it's greater mass and slower speed it's a race that the electron can never win.
(When written the MathJax renderer was working but during editing it failed. The few equations and special characters are from this Wikipedia article should any subsequent editing be necessary. Thanks. This is my first attempt at using this feature.)
I won’t write ten paragraphs just to try sound smart. The answer to this question, I think, is quite simple. Space doesn’t have mass, yet it must contract where mass experiences a time dilation, to prevent the possibility of mass exceeding light speed from an unprimed observer’s perspective. It would contract in accordance with the Lorentz Factor for length contractions. To prevent energy from blue shifting for the unprimed observer, and ensure that wavelengths remain just as constant as light speed in all frames of references, I think it would be fair to assume, therefore, that light contracts with space, or at least vanishes with it. If this weren’t the case a light bulb in a ship moving close to light speed would become lethal due to thickening energy. Assuming photons have three dimensions, this would even work for light moving perpendicular to a ship’s motion. This answer involves an assumption, but I think it’s a reasonable one.