2
$\begingroup$

Trying to understand Schutz's AFCIGR, where does the minus sign appear from in the metric tensor?

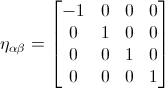
metric

I understand that this expresses the invariance of the spacetime interval. Schutz says (I think) that the metric is a (0,2) tensor. I assume that means it is the product of two one-forms, so presumably one of these one-forms has a -ve time component. What does that mean? Why don't both one-forms have a -ve time component? Looking at a Minkowski diagram what is a straightforward way to understand/visualise those one-forms? At my level, two things have been multiplied together to give a 4x4 matrix which has -1 in the top left corner. What are those two things and why do they give a -1 time component?

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ A comment to the question (v1): Note that in general (and in fact also in the specific case mentioned), a $(0,2)$ tensor is not always a product of co-vectors. Instead, it is a sum of products of co-vectors. (In the specific case the sum contains at least $4$ terms.) In fact, the same is true if the $-1$ had been a $+1$. $\endgroup$
    – Qmechanic ♦
    Commented Jun 23, 2011 at 12:15

2 Answers 2

Reset to default
3
$\begingroup$

The metric is a symmetric bilinear form $$ ds^2 = \eta_{ij} dx^i dx^j = -dt^2 + dx^2 + dy^2 + dz^2 $$ Hence the minus sign is not a property of the one-forms $dx^i$ but of the coefficients $\eta_{ij}$

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks for that but I still don't understand where the minus sign comes from. $\endgroup$
    – Peter4075
    Commented Jun 24, 2011 at 6:51
  • 1
    $\begingroup$ @user4075: I'm not sure I understand what you mean with "where the minus sign comes from". In SR the space-time is a flat space with a metric of signature (-+++), more or less per definition. There are of course other ways of saying the same thing, eg the isometry group is SO(3,1), but I don't think this answers your question does it? $\endgroup$
    – Olof
    Commented Jun 24, 2011 at 8:04
  • $\begingroup$ Olaf: I'm happy to admit I'm out of my depth here (eg I don't know what a "symmetric bilinear form" is, and I've still struggling with one-forms). I assumed (erroneously - thanks Qmechanic) that a (0,2) tensor is always the product of two one-forms, and thus assumed this might explain why there's a minus sign in the metric. Wikipedia (Introduction to special relativity) says, "Minkowski... found that the correct formula was actually quite simple, differing only by a sign from Pythagoras's theorem". So I'm assuming he just sort of discovered it worked. $\endgroup$
    – Peter4075
    Commented Jun 24, 2011 at 14:37
1
$\begingroup$

Although one can think of the metric as about infinitesimal distances, it can also be thought of in a dual way, as about differential equations. Thus, with your sign convention for the metric, the wave equation is $$g^{ij}\frac{\partial^2\phi(x)}{\partial x^i\partial x^j}=-\frac{\partial^2\phi(x)}{\partial t\partial t}+\frac{\partial^2\phi(x)}{\partial x\partial x}+\frac{\partial^2\phi(x)}{\partial y\partial y}+\frac{\partial^2\phi(x)}{\partial z\partial z}=0.$$ In this POV, the metric determines the differential equations that are satisfied by the fields that are introduced in the space-time. The Laplace equation has very different properties than does the wave equation.

There are formalisms in which d$t$ is replaced by id$t$, so that the metric can be uniformly positive. However most people keep this as a mathematical trick up one's sleeve, not as a fundamental part of the construction of a theory. There was a period in the development of GR when imaginary time was much more commonly used than it is now.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.