I'm an autodidact and can't follow the part after "it is easily seen that"... which is the 31st equation:
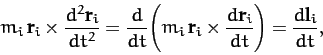
Shouldn't it be:
$m_i\,{\bf r}_i\times \frac{d^2{\bf r}_i }{dt^2}= \frac{d}{dt}(m_i r_i \times \frac{dr_i}{dt}) - \frac{d(m_i r_i)}{dt} \times \frac{d(r_i)}{dt}$
According to the rule of derivation:
$(u v)' = u'v + v'u$ where $u(t)=m_i r_i$ and $v(t)=(r_i)'$
Furthermore, I would be most grateful if you could point me to resources that could help me with this sort of problems.
It should be:
$m_i\,{\bf r}_i\times \frac{d^2{\bf r}_i }{dt^2}= \frac{d}{dt}(m_i r_i \times \frac{dr_i}{dt}) - \frac{d(m_i r_i)}{dt} \times \frac{d(r_i)}{dt}$
BUT, look at the last term of this equation, it can be written as,
$\frac{m_id(r_i)}{dt} \times \frac{d(r_i)}{dt}$ assuming mass is not a function of time. This can further be written as, $m_i[\frac{d(r_i)}{dt} \times \frac{d(r_i)}{dt}]$ (convince yourself of this expression by writing out the matrix form of the cross product).
You are taking the cross product of the same vector, and cross product is the area of the parallelogram created by the 2 vectors (assume you take the absolute value of the cross product). So in case of same vectors, the area is zero, hence the last term goes to zero.
Hence: $\frac{d(m_i r_i)}{dt} \times \frac{d(r_i)}{dt}=0$
You're right, what you have written is the correct expression. Notice, however, that
$$\frac{\mathrm{d}}{\mathrm{d}t} (m_i \vec{r}_i) \times \frac{\mathrm{d} \vec{r}_i}{\mathrm{d}t} = m_i \frac{\mathrm{d} \vec{r}_i }{\mathrm{d}t} \times \frac{\mathrm{d} \vec{r}_i}{\mathrm{d}t} = \vec{0}$$
where the last equality comes from the fact that $\vec{a} \times \vec{a} = 0$ for any vector $\vec{a}$.
