Tricky question involving finding the magnetic field given the wave equation for the electric field and it's solution

Question

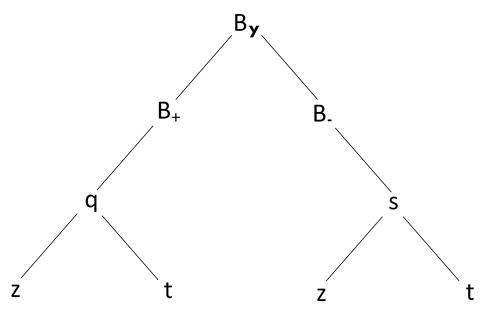
Consider the wave equation for linearly $x$ polarized waves travelling in the $\pm z$ directions:

$$\frac{\partial^2\vec E_x}{\partial t^2}=c^2\frac{\partial^2\vec E_x}{\partial z^2}\tag{1}$$ The general solution to equation $(1)$ is $$\vec E_x=\vec E_{+}(q)+\vec E_{-}(s)$$ where $\vec E_{+}$ and $\vec E_{-}$ are arbitrary functions. $$q=z-ct$$ & $$s=z+ct$$

Calculate the general form of the magnetic field in terms of $\vec E_{+}$ and $\vec E_{-}$

---

I am stuck at the very beginning.

I have the solution to this question, but the problem is I cannot understand the author's solution.

So instead I will ask questions about the author's solution which is as follows:

We can obviously write $$\vec B_y=\vec B_{+}(q)+\vec B_{-}(s)\tag{a}$$ and $$\frac{\partial \vec B_y}{\partial t}=-\frac{ \partial \vec E_x}{\partial z}\tag{b}$$ then $$\frac{\partial q}{\partial t}\Bigg |_z\cdot\frac{d\vec B_{+}}{dq}+\frac{\partial s}{\partial t}\Bigg |_z\cdot\frac{d\vec B_{-}}{ds}=-\left(\frac{\partial q}{\partial z}\Bigg |_t\cdot\frac{d\vec E_{+}}{dq}+\frac{\partial s}{\partial z}\Bigg |_t\cdot\frac{d \vec E_{-}}{ds}\right)\tag{c}$$ $$\implies -c\frac{d\vec B_{+}}{dq}+c\frac{d\vec B_{-}}{ds}=-\frac{d\vec E_{+}}{dq}-\frac{d\vec E_{-}}{ds}\tag{d}$$ therefore $$\vec B_y=\frac{1}{c}\left[\vec E_{+}(q)-\vec E_{-}(s)\right]\tag{e}$$

---

I understand completely how $(\mathrm{d})$ follows from $(\mathrm{c})$.

I don't understand why you "can obviously write $\vec B_y=\vec B_{+}(q)+\vec B_{-}(s)$"; What is the origin of this equation: $(\mathrm{a})$? It is far from obvious to me that you can write $\vec B_y=\vec B_{+}(q)+\vec B_{-}(s)$.

Also, what is the origin of equation $(\mathrm{b})$? What does it mean? Is it a reformulation of one of Maxwell's equations?

Lastly, how does $(\mathrm{c})$ follow from $(\mathrm{b})$? I note that the author is using the chain rule here, but I'm not sure about the logic.

---

If anyone could help me by giving hints or explanations to any of the questions I have raised then I would be most grateful.

---

EDIT:

Thanks to @Farcher I now understand part $(\mathrm{a})$ and was able to write an answer of my own elaborating on parts $(\mathrm{b})$ and $(\mathrm{c})$.

---

EDIT 2:

The only part of the authors solution which I still don't understand is how $(\mathrm{e})$ follows from $(\mathrm{d})$.

Rearranging $(\mathrm{d})$ we have that $$c\left(\frac{d\vec B_{-}}{ds}-\frac{d\vec B_{+}}{dq}\right)=-\left(\frac{d\vec E_{-}}{ds}+\frac{d\vec E_{+}}{dq}\right)\tag{d}$$ we know that $$\vec E_x=\vec E_{+}(q)+\vec E_{-}(s)$$ and $$\vec B_y=\vec B_{+}(q)+\vec B_{-}(s)$$

Rearranging $(\mathrm{d})$ further I find that

$$c\frac{d\vec B_{-}}{ds}+\frac{d\vec E_{-}}{ds}=c\frac{d\vec B_{+}}{dq}-\frac{d\vec E_{+}}{dq}\tag{d}$$

My first thought was to integrate both sides but since the LHS depends on $s$ and the RHS on $q$ it cannot be correct to write

$$\int \left(c\frac{d\vec B_{-}}{ds}+\frac{d\vec E_{-}}{ds}\right)ds=\int \left(c\frac{d\vec B_{+}}{dq}-\frac{d\vec E_{+}}{dq}\right)dq\tag{?}$$

So I am stuck at this point.

Could someone please explain to me how I can obtain the result $$\bbox[5px,border:2px solid red]{\vec B_y=\frac{1}{c}\left[\vec E_{+}(q)-\vec E_{-}(s)\right]}\tag{e}$$ from $$\bbox[yellow]{-c\frac{d\vec B_{+}}{dq}+c\frac{d\vec B_{-}}{ds}=-\frac{d\vec E_{+}}{dq}-\frac{d\vec E_{-}}{ds}}\tag{d}\,\,?$$

Answer 1

For my own reference (and others if they're interested) I'm going to expand on what @Farcher wrote in his answer for parts $(\mathrm{b})$ and $(\mathrm{c})$:

To show part $(\mathrm{b})$ from Faraday's Law: $$\begin{align}\frac{\partial \vec B_y}{\partial t}&= -\vec \nabla \times \vec E_x\\&=- \begin{vmatrix} \hat i & \hat j & \hat k \\ \frac{\partial}{\partial x} & \frac{\partial}{\partial y} &\frac{\partial}{\partial z} \\ E_x & 0 & 0 \\ \end{vmatrix}\\&=-\left(\frac{\partial E_x}{\partial z }\hat j-\frac{\partial E_x}{\partial y}\hat k\right)\\&=-\frac{\partial \vec E_x}{\partial z}\end{align}$$

as the $\hat k$ component disappears since $E_x$ does not depend on $y$ so it's derivative is zero.

The $\vec B_y$ only has a $\hat j$ component as it is oscillating in the $y$ direction. After taking the curl of the electric field only the $\hat j$ component survived; so this makes perfect sense as the vector components must match for equality to hold.

Hence, we conclude that $$\frac{\partial \vec B_y}{\partial t}=-\frac{\partial \vec E_x}{\partial z}$$ which is indeed equation $(\mathrm{b})$

---

For part $(\mathrm{c})$ we have $$\vec B_{y}=\vec B_{y}(\vec B_{+},\vec B_{-})$$ $$\vec B_{+}=\vec B_{+}(q) \qquad\text{and}\qquad \vec B_{-}=\vec B_{-}(s)$$ $$q=q(z,t) \qquad\text{and}\qquad s=s(z,t)$$

So the appropriate tree diagram which connects the dependent variables at the top to the independent variables at the bottom is:

From the tree diagram we see that

$$\frac{\partial\vec B_y}{\partial t}=\frac{\partial\vec B_y}{\partial\vec B_{+}}\cdot\frac{d\vec B_{+}}{d q}\cdot \frac{\partial q}{\partial t}+\frac{\partial\vec B_y}{\partial\vec B_{-}}\cdot\frac{d\vec B_{-}}{d s}\cdot \frac{\partial s}{\partial t}$$

Now since $$\frac{\partial\vec B_y}{\partial\vec B_{+}}=\frac{\partial\vec B_y}{\partial\vec B_{-}}=1$$ and $$\frac{\partial q}{\partial t}=-c\,,\qquad \frac{\partial s}{\partial t}=c$$ therefore I can write $$\fbox{$\color{blue}{\frac{\partial\vec B_y}{\partial t}=-c\frac{d\vec B_{+}}{d q}+c\frac{d\vec B_{-}}{d s}}$}$$

which is the LHS of $(\mathrm{c})$

---

The RHS of $(\mathrm{c})$ is completely analogous to the method used to get the LHS. But for reference I'm going to write out the steps explicitly.

Similar to before we have $$\vec E_{x}=\vec E_{x}(\vec E_{+},\vec E_{-})$$ $$\vec E_{+}=\vec E_{+}(q) \qquad\text{and}\qquad \vec E_{-}=\vec E_{-}(s)$$ $$q=q(z,t) \qquad\text{and}\qquad s=s(z,t)$$

So the appropriate tree diagram for this case is:

and from it we see that

$$-\frac{\partial\vec E_x}{\partial z}=-\left(\frac{\partial\vec E_x}{\partial\vec E_{+}}\cdot\frac{d\vec E_{+}}{d q}\cdot \frac{\partial q}{\partial z}+\frac{\partial\vec E_x}{\partial\vec E_{-}}\cdot\frac{d\vec E_{-}}{d s}\cdot \frac{\partial s}{\partial z}\right)$$

Now since $$\frac{\partial\vec E_x}{\partial\vec E_{+}}=\frac{\partial\vec E_x}{\partial\vec E_{-}}=1$$ and $$\frac{\partial q}{\partial z}=\frac{\partial s}{\partial z}=1$$ therefore I can write $$\fbox{$\color{red}{-\frac{\partial\vec E_x}{\partial z}=-\frac{d\vec E_{+}}{d q}-\frac{d\vec E_{-}}{d s}}$}$$

which is the RHS of $(\mathrm{c})$.

Answer 2

Let $c=1$ to simplify the notation.

We know that $$ \boldsymbol B'_-(s)+\boldsymbol E'_-(s)=\boldsymbol B'_+(q)-\boldsymbol E'_+(q) $$ where the prime denotes differentiation.

Note that $s$ and $q$ are independent variables. The l.h.s. depends on $s$ only, and the r.h.s. on $q$ only. Thus, both sides must in fact be constants: $$ \begin{aligned} \boldsymbol B'_-(s)+\boldsymbol E'_-(s)&=\boldsymbol\alpha\\ \boldsymbol B'_+(q)-\boldsymbol E'_+(q) & =\boldsymbol \alpha \end{aligned} $$ for some constant vector $\boldsymbol\alpha$. These equations are trivial to integrate: $$ \begin{aligned} \boldsymbol B_-(s)+\boldsymbol E_-(s)&=\boldsymbol \alpha s+\boldsymbol\beta\\ \boldsymbol B_+(q)-\boldsymbol E_+(q) & =\boldsymbol \alpha q+\boldsymbol\gamma \end{aligned} $$ for some integration constants $\boldsymbol\beta,\boldsymbol\gamma$.

From this, we obtain $$ \boldsymbol B_y=(\boldsymbol E_+(q)-\boldsymbol E_-(s))+z\boldsymbol \alpha'+\boldsymbol\beta' $$ with $\boldsymbol\alpha'=2\boldsymbol\alpha$ and $\boldsymbol\beta'=\boldsymbol\beta+\boldsymbol\gamma$.

This is the most general solution consistent with your equations. The boundary conditions, which you didn't specify, presumably set $\boldsymbol\alpha'=\boldsymbol\beta'=\boldsymbol 0$.

Answer 3

(a) An electromagnetic wave has a magnetic field $B_+/B_-\,\hat y$ oscillating exactly in phase with and at right angles to an electric field $E_+/E_- \, \hat x$ both of which are at right angles to the direction of propagation $\hat z$.

(b) is Faraday's law in differential form $\dfrac{\partial \vec B}{\partial t}= -\vec \nabla \times \vec E$

(c) is the application of the chain rule.