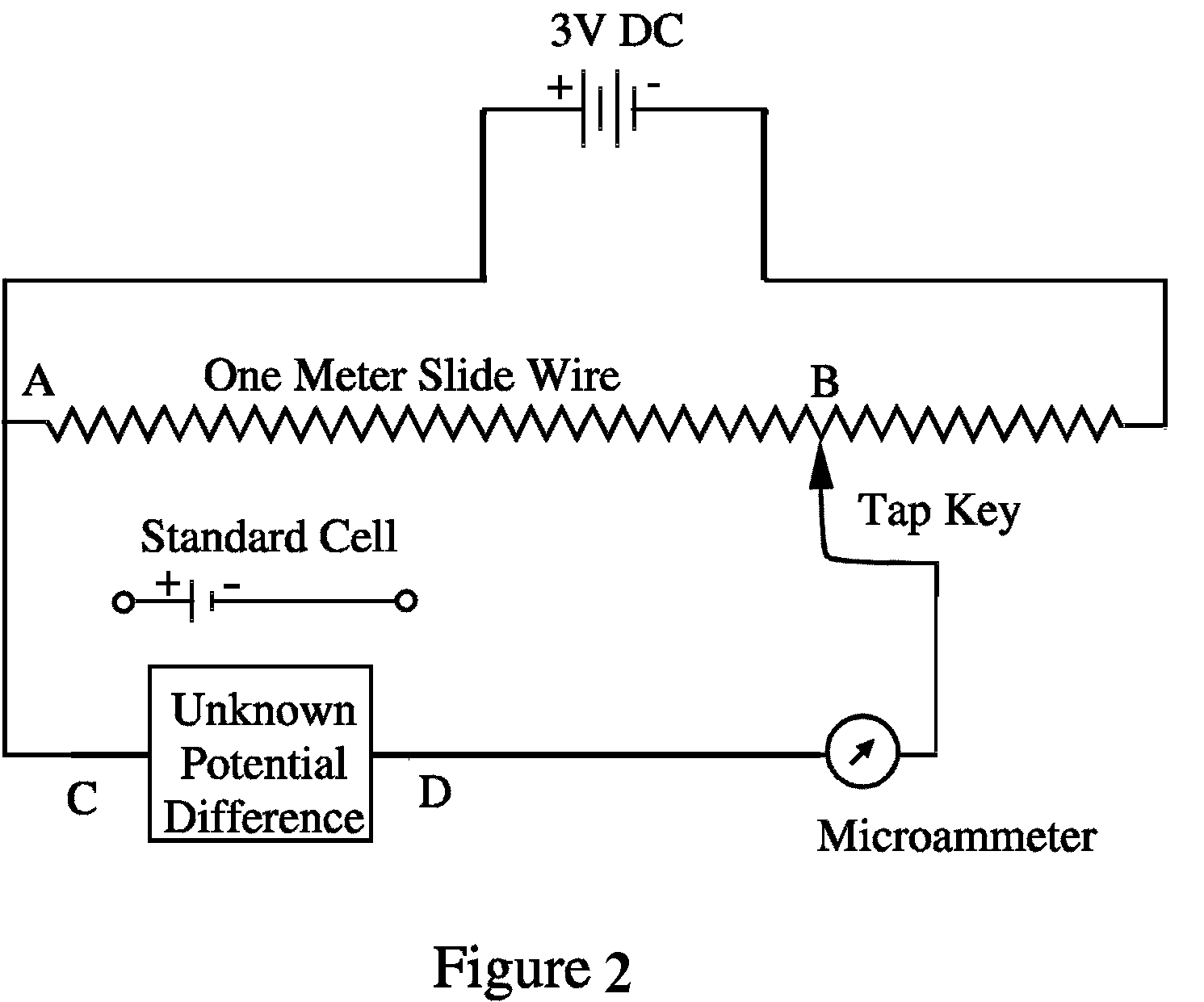
Now, is there any current in the potentiometer wire at the null point?
If by potentiometer wire, you mean the resistance element, the answer is yes.
Let the current, from left to right, through the section of the potentiometer resistance from $A$ to $B$, $R_{AB}$ be labelled $I_{AB}$.
Let the current, from left to right, through the remainder of the potentiometer resistance $R_B$ be labelled $I_B$.
Clearly, if $I_{AB} = I_B$, there is no current through the arm of the potentiometer but this does not imply that these currents are zero.
The voltage across $R_{AB}$ is the unknown voltage $V_{CD}$ thus, by Ohm's law
$$I_{AB} = \frac{V_{CD}}{R_{AB}}$$
By KVL, the voltage $V_B$ across $R_B$ is
$$V_B = 3V - V_{CD}$$
and thus, by Ohm's law
$$I_B = \frac{V_B}{R_B} = \frac{3V - V_{CD}}{R_B}$$
Setting both currents equal yields
$$\frac{V_{CD}}{R_{AB}} = \frac{3V - V_{CD}}{R_B}$$
which can be solved for $V_{CD}$
$$V_{CD} = 3V \frac{R_{AB}}{R_B + R_{AB}}$$
But this is just, by voltage division, the voltage across $R_{AB}$ if the unknown voltage source were removed which, if you think about it clearly, must be the case for there to be zero current in the wiper arm.
Finally, if the total resistance of the potentiometer resistance element is $R$, we have
$$R_{AB} = R \chi$$
and
$$R_B = R (1 - \chi)$$
where
$$0 \le \chi \le 1$$
Thus, we can write
$$V_{CD} = \chi 3V $$