I've often seen in textbooks that authors renormalize diagrams by setting external momentum to zero. Under what conditions is this justified?
An example of this is done in Manohar and Wise's book on Heavy Quark Physics after they renormalize QED and then calculate the operator renormalization, $ Z _S $, of \begin{equation} S = \frac{1}{ Z _S }\bar{\psi} _b \psi _b = \frac{ Z _\psi }{Z _S } \bar{\psi} \psi \end{equation} where $ \psi_b $ is the bare field. They calulate this through the diagram,
$\hspace{5cm}$ 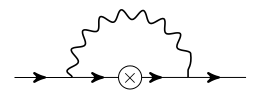
where the cross indicates an operator insertion. The authors then say ``The operator, $ S $, contains no derivatives (and $Z _S$ is mass independent in the $\overline{MS}$ scheme), so $Z _S$ can be determined by evaluating (the diagram) at zero external momentum (and neglecting the (fermion) mass).'' Are these the two conditions necessary,
- The operator doesn't have derivatives
- The quantity of interest has no mass dependence
and if so how do we know that the quantity you want to calculate (in this case, $ Z _S $) is mass independent, ahead of time?
