Intuition behind mass corrections to massless fermions

Question

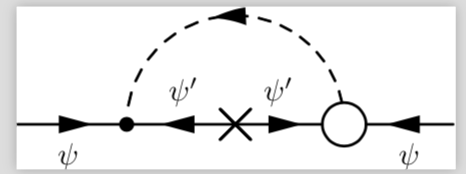
I'm trying to understand the intuition behind the mass correction to massless fermions. To be concrete lets consider a theory with a massless Weyl fermion ($\psi $), as well as two massive particles, a complex scalar ($\phi $) and another Weyl fermion ($ \psi ' $), \begin{equation} {\cal L} = {\cal L} _{ kin} - \frac{1}{2} m ^2 \left| \phi \right| ^2 - M \left(\psi '\psi ' + h.c. \right) - \frac{ \lambda }{ 4!} \left| \phi \right| ^4 - g \phi \psi \psi ' + h.c. \end{equation} This Lagrangian would be valid if for example we impose a $ U(1) $ symmetry such that, \begin{align} & \psi \rightarrow e ^{ i \alpha } \psi \\ & \phi \rightarrow e ^{ - i\alpha } \psi \\ & \psi ' \rightarrow \psi ' \end{align} Now consider the lowest order mass correction the for massless fermion,

$\hspace{4cm}$

The mass correction can be calculated by setting external momenta to zero: \begin{align} i {\cal M} & \sim g ^2 \int \frac{ d^4 \ell }{ (2\pi)^4 } \frac{ 1 }{ \ell ^2 - m ^2 } \frac{ M }{ \ell ^2 - M ^2 } \\ & \sim \frac{g ^2}{16 \pi^2}M \left( \frac{1}{ \epsilon } + \log \left( \frac{ \mu ^2 }{ \Delta ( m , M ) } \right) \right) \end{align} where $ \Delta $ is a function of $m$ and $ M $. In $\overline{MS}$ we toss the infinity and the first order correction is \begin{equation} m _{ \psi } \sim g ^2 M \log \frac{ \mu ^2 }{ \Delta } \end{equation} This has three interesting features which I am trying to understand:

1. It seems that the fermion doesn't decouple from the theory in the limit that its mass goes to infinity. Why are we not justified in integrating it out in this case?
2. The calculation is very insensitive to the boson mass. Is this an accident or has a deeper explanation?
3. The calculation has large logs unless $ \mu \sim m, M $. It seems if you renormalize at this scale the log goes away and the physical mass goes to zero! So if I am understanding correctly the particle is massless when doing experiments at the scale of the other particles in the theory (at least at lowest order in perturbation theory) but heavy when its much below this scale. This seems very strange!

Answer 1

I guess that the confusion here is that you mix Weyl notation and Feynman diagrams with Dirac propagators. As you notice yourself, the theory has a global (anomalous) U(1) symmetry that prohibits a mass term $m\,\psi\psi$ (in Weyl notation). If I try to draw your diagram (ab)using the usual Dirac propagator lines for Weyl fermions, I get

Here, the right (empty) vertex does not exist, as it violates U(1) charge conservation. On the other hand, in order to obtain the $M$ in the numerator of your expression, I need the mass insertion (indicated by the cross). (BTW, there is a typo in your transformation law $\phi\to e^{-\mathrm{i}\alpha}\psi$, it should be $\phi\to e^{-\mathrm{i}\alpha}\phi$). If you are unhappy with the Weyl argumentation, you may recast everything in Dirac notation, which yields the same result.