What is the third law action pair of buoyancy?

Question

Consider a block floating in a fluid. The force due to gravity balances the buoyant force exerted on the block. But there must be a third-law action pair to the buoyant force. If the block exerts a force on the fluid, that would mean that the pressure in fluid just below the block is more than that in other places that are on the same horizontal level.

I do not believe that to be the case, since it doesn't conform to real world observations.

Also, if the block has a $m$ and density $\rho$, and the fluid + the container has a mass $M$. What is the mass of the block + container + fluid (as in the picture), as measured by a weighing machine underneath the container.

According to my common sense, since the buoyant force is internal, the measured mass of the system should be $m + M$. Am I right?

Answer 1

The buoyant block does exert a force on the water, it's force is equal to the mass of the displaced water, so the pressure of the water immediately beneath the block is exactly the same as the pressure of the water at that height in the rest of the container.

Indeed, the mass of the system is just the mass of container with water + mass of block

Answer 2

The force due to gravity balances the buoyant force exerted on the block

The buoyant force is there because of gravity (There is a difference in pressure as we go deeper in to the ocean).

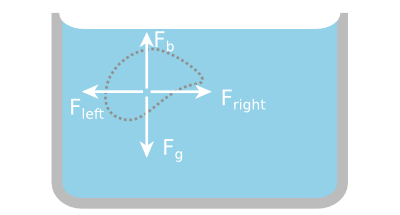
There's an easy way to think it through. Imagine a beaker with some water, and it is standing still. There is no internal motion. Consider a small portion of this water(there's nothing else, just water). Who is balancing this portion of water?

The pressure from all sides are not equal! There is a gradient of pressure inside because of varying height(due to gravity). Thus bottom layer exerts more pressure than its top ones. Clearly a net effective pressure is pushing the water upwards.This pressure force is the buoyant force. The force that balances gravity. Note that this is not the third-law opposite pair; that would be the gravitational force on earth by the liquid piece (very small effect on earth).

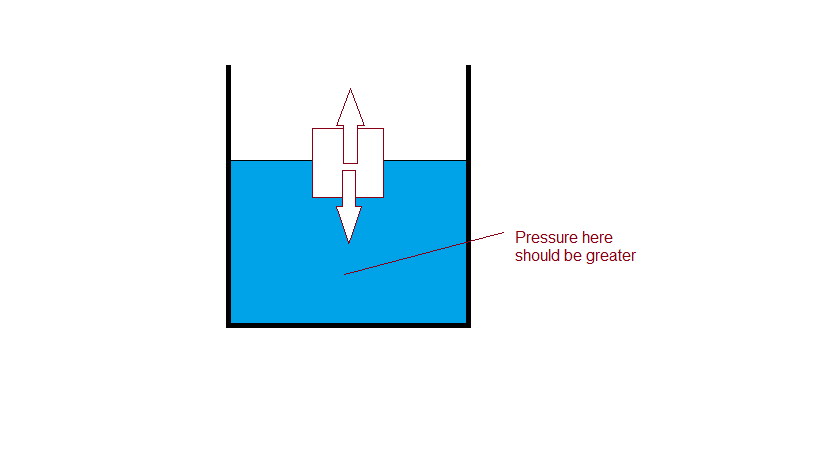
If the block exerts a force on the fluid, that would mean that the pressure in fluid just below the block is more than that in other places that are on the same horizontal level.

Pascal's law states exactly otherwise! It would be the same everywhere in the liquid. But this case is different because gravity already established a pressure gradient inside the water body. If there was an uneven pressure in the same layer, water would begin to flow on its own!

We'll try to derive an expression to determine whether or not the object will float.

$F_{b}=mg$

$F_{b}=\rho_{water} Vg$

Let's replace the same piece of water with an empty box. Three things could happen:

1. It could float
2. It could sink
3. It could stand still

$F_{b}=\rho_{box} V(g+a)$

Where acceleration $a$ could be taken as positive for upward motion. Equating both, we get

$\rho_{water} Vg=\rho_{box} V(g+a)$

On simplifying

$a=g\left ( \frac{\rho _{water}}{\rho_{box}}-1 \right )$

If the density of the box exceeds that of the water, RHS becomes negative and acceleration is downwards(sinking). Otherwise it would be positive(floating). If it is equal, $RHS = 0$ (still).