1
$\begingroup$

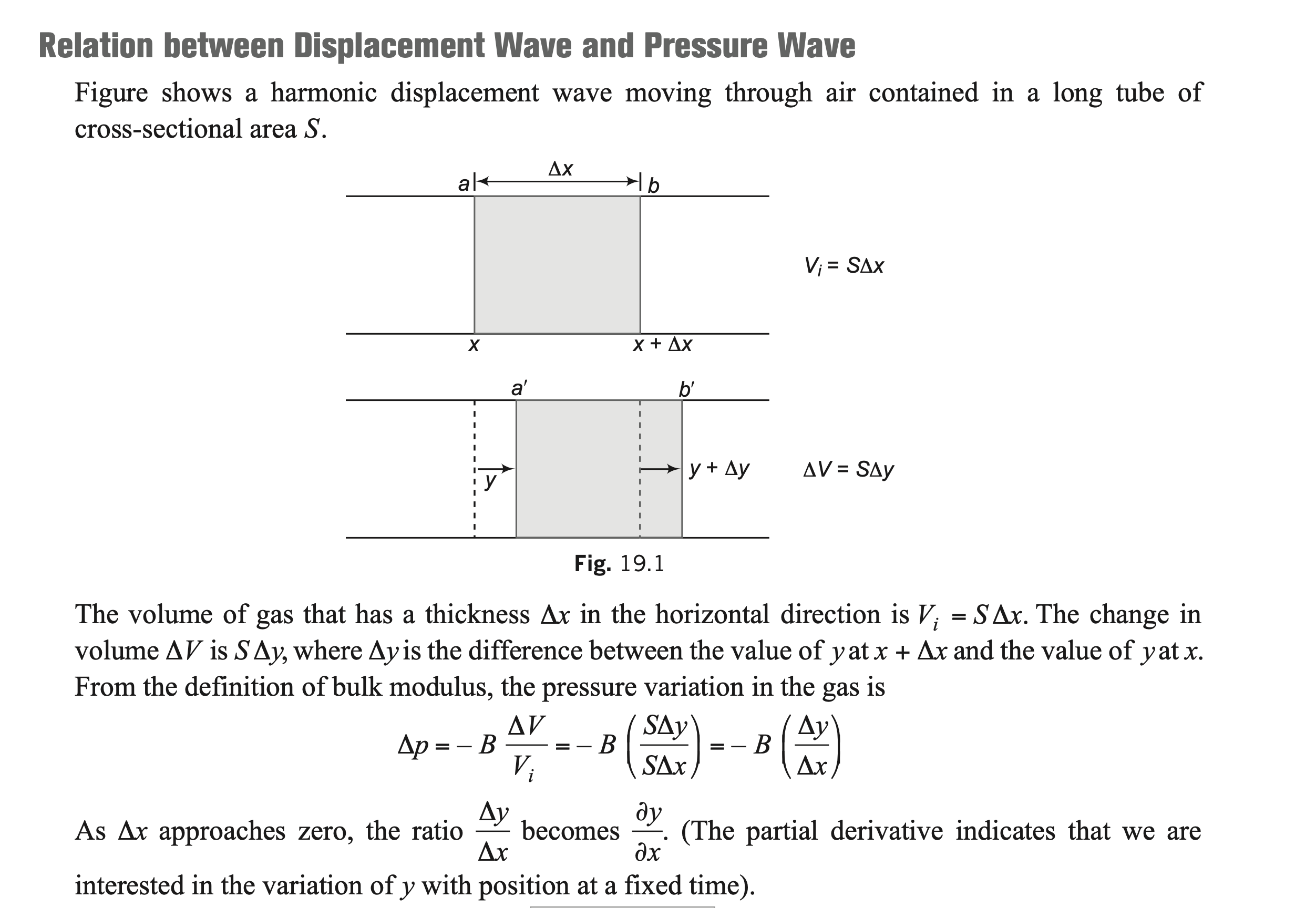
acoust

The attached image shows the relation between displacement wave and pressure wave.

We have derived the change in volume as:

$$\Delta V = (S)(\Delta y)$$

And used the relation with the Bulk Modulus to find the change in pressure.

But since the whole volume element has shifted to right by $y$ (the displacement), how are we measuring the change in pressure at the point $x$ anymore, aren't we measuring the change in pressure at the point $x + y$?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

When we define the Bulk Modulus, $$B=\frac{∆p}{\frac{-∆V}{V}} \tag{1}$$

we consider the "pressure change", $∆p$ applied initially at the initial position and then as the volume squeezes, the pressure change is assumed to be invariant and that same pressure is written on the numerator.

Similarly, here we can consider the pressure change to be at position $x$ and as the volume squeezes, the $eq(1)$ is used to get $∆p$ using the precalculated $∆V$.

So, that pressure change is not the one at $x+y$ instead it is that at $x$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ So, is it that we are finding the pressure that created the above change and not the pressure that resulted from that change? $\endgroup$
    – User 314159
    Commented 2 days ago
  • 1
    $\begingroup$ Not exactly, but yes. It is that we are finding the pressure at the initial position and not at the position which resulted due to the compression of the volume due to that initial pressure. $\endgroup$
    – CP of Physics
    Commented 2 days ago

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.