Problem in understanding wave equation in a gas column

Question

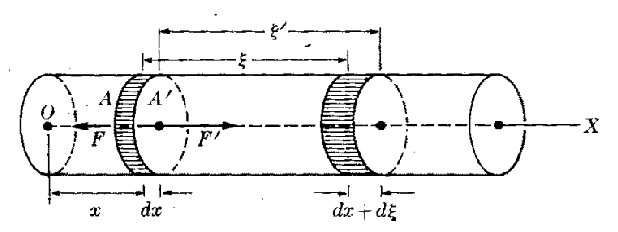
In my textbook, the author derives the displacement wave equation in a gas within a cylindrical pipe (when the deformation of the gas is small):

$$\frac{\partial^2\xi}{\partial t^2} = \frac{B}{\rho_0} \frac{\partial^2\xi}{\partial x^2}$$

where $B$ is the bulk modulus of elasticity of the gas and $\rho_0$ is the initial density of the gas, the steps are the followings:

When the pressure of the gas is disturbed, a volume element such as $Adx$ is set in motion, as a result, section $A$ is displaced an amount $\xi$; and section $A'$ an amount $\xi'$. The mass within the undisturbed volume is $\rho_0 Adx$, if $\rho$ is the density of the disturbed gas, the mass of the disturbed volume element is $\rho A(dx + d\xi)$. The conservation of the matter requires that both masses be equal, i.e.

$$\rho A(dx+d\xi)=\rho_0 Adx$$

from this, we get that

$$\rho = \frac{\rho_0}{1 + \partial\xi/\partial x}$$

when $\partial\xi/\partial x$ is small we can approximate it by:

$$\rho = \rho_0\left(1-\frac{\partial\xi}{\partial x}\right)$$

here is where my problem arises, $\partial\xi/\partial x$ is the deformation of the volume element at $x'$ and $\rho$ is the density of the perturbed gas which is at $x' + \xi(x')$, then the last equality can be written more formally as:

$$\rho\big(x' + \xi(x')\big) = \rho_0\left(1-\left.\frac{\partial\xi}{\partial x}\right|_{x=x'}\right)$$

But in the next steps, the equality is regarded as if both sides were valued at the same point, i.e.

$$\rho\big(x'\big) = \rho_0\left(1-\left.\frac{\partial\xi}{\partial x}\right|_{x=x'}\right)$$

I think that this is because $\xi(x')$ is small compared with $x'$, Is this what is happening? What am I doing wrong?

Answer 1

Short Answer:

We are able to ignore the difference in measurement location because we are assuming very small disturbances.

Longer, Very Mathy Answer:

More formally, we should write the mass within the element between $x$ and $x'$ as the integral $$M_{xx'} = \int_x^{x'}\rho(y)S\ dy,$$ where we have assumed that the density $\rho$ may vary with position. If we define $x'-x$ as $dx$ and assume that $dx$ is very small, then we may expand the integral in a Taylor series about $x$ as $$ M_{xx'} = \left[\int_x^z\rho(y)S\ dy\right]_{z=x} + dx\left[\frac{\partial}{\partial z}\int_x^z\rho(y)S\ dy\right]_{z=x} + \text{h.o.t.} = dx\rho(x)S + \text{h.o.t.}, $$ where $\text{h.o.t.}$ denotes higher order terms, or terms that are proportional to $dx^2$. As long as the difference between $x$ and $x'$ is very small, this relationship holds. Thus, if at some time $t$ we have $x'= x + dx + \xi(t)$, as long as $\xi(t)$ is very small, we may still write $$ M_{xx'} \approx (dx + \xi(t))\rho(x,t)S. $$ By the same logic, we could also have written $$ M_{xx'} \approx (dx + \xi(t))\rho(x'(t),t)S, $$ as the error terms would hav been the same order. In the end, the fact that $dx$ and $\xi$ are assumed to be very small (and that the density varies smoothly in space) is what allows us to change where the density is evaluated. Cases when this assumption is violated occur when you are looking at large deformations (think of long-time fluid flow or really stretching elastic media), or you are trying to impose a boundary condition on a boundary that moves a lot. But for small signal acoustics (in any domain), this approximation is usually very good.

For the sake of completion, here is the rest of the derivation with this same approach: Note that $\xi$ here has been defined relative to $x$, and so is an implicit function of $x$. Thus, we may write $$ M_{xx'} \approx (dx + \xi(x,t))\rho(x,t)S. $$ Since $M_{xx'}$ does not change with time (it may change with position, though), we then conclude that $$ (dx + \xi(x,t))\rho(x,t) = (dx + \xi(x,0))\rho(x,0). $$ Rearranging yields $$ \rho(x,t) = \frac{1 + \xi(x,0)/dx}{1 + \xi(x,t)/dx}\rho(x,0). $$ Finally, assuming $\xi(x,0)=0$ and noting that $$\frac{\partial\xi}{\partial x} \approx \frac{\xi(x,t)}{dx},$$ we may obtain your result: $$ \rho(x,t) = \frac{\rho(x,0)}{1 + \partial\xi/\partial x}. $$