Criteria for using $\tau = I \alpha$ about particular points (simple 2D rotations)

Question

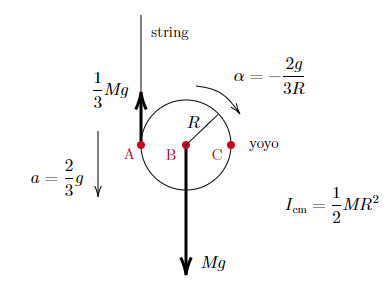
I'm looking at the classic yoyo problem wherein a solid disk of radius $R$, mass $M$, (and thus moment of inertia $I_\text{cm} = \frac{1}{2}MR^2$) unfurls from the string coiled around it due to the pull of gravity. This is well-known and well-solved problem: the tension in the string is known to be $\frac{1}{3}Mg$, the resulting linear acceleration of the yoyo is $a = \frac{2}{3}g$ and the angular acceleration of the yoyo is $\alpha = - \frac{2g}{3R}$ (taking positive as counterclockwise). I've provided a diagram of all of these well-known results as well as three different points $A$, $B$, and $C$, which are chosen relative to the position of the yoyo at this snapshot in time, but are at rest relative to our observational frame.

Using point $A$ as the reference point, $\tau_A = -RMg$ (due to gravity only) and $I_A = \frac{3}{2}MR^2$ (via the parallel axis theorem). In this case the quantity $\frac{\tau_A}{I_A} = -\frac{2g}{3R}$ agrees with $\alpha$.

Using point $B$ as the reference point, $\tau_B = -\frac{1}{3}RMg$ (due to tension only) and $I_B = I_\text{cm} = \frac{1}{2}MR^2$. In this case the quantity $\frac{\tau_B}{I_B} = -\frac{2g}{3R}$ also agrees with $\alpha$.

However, using point $C$ as the reference point, $\tau_C = MRg - \frac{2}{3}MRg = \frac{1}{3}MRg$ (net torque from both forces) and $I_C = \frac{3}{2}MR^2$ (via the parallel axis theorem). In this case, we find $\frac{\tau_C}{I_C} = \frac{2g}{9R}$, which is clearly not $\alpha$.

Obviously, something is wrong with using point $C$ along with the relationship $\tau = I \alpha$, whereas using points $A$ and $B$ yield the correct results. What is the criteria for using $\tau = I \alpha$ that fails with point $C$ but is satisfied for points $A$ and $B$?

Answer 1

Let's derive $\tau = I\alpha$ to see the conditions of its validity.

At any instant, we may describe the motion of a rigid body as a rotation about an arbitrary point, on top of a translation. Specifically, the velocity of any point on the rigid body may be expressed as $$\vec v = \vec v_0 +\vec \omega\times\vec r,$$ where $\vec v_0$ is the velocity of the center of rotation you have chosen, $\vec r$ is the relative position with respect to this point, and $\vec \omega$ is the angular velocity. Taking the derivative with respect to time, $$\vec a = \vec a_0 + \vec \alpha \times \vec r + \vec \omega\times (\vec v - \vec v_0)$$ $$=\vec a_0 + \vec \alpha\times \vec r + \vec \omega\times (\vec \omega \times \vec r).$$ The second term on the right hand side is the tangential acceleration, while the third term is the centripetal acceleration.

To simplify things a bit, since this is a 2D problem with the axis of rotation perpendicular to the page, I will assume that $\omega$ and $\alpha$ are both perpendicular to the page, i.e. (anti-)parallel to each other. We can break down the position vector into two components: $\vec r = \vec R + \vec r_\parallel$, where $\vec R$ is perpendicular to $\vec \omega$ (and hence the axis of rotation), and $\vec r_\parallel$ is parallel to it. Then, $\vec\alpha\times\vec r = \vec\alpha\times\vec R$ and $\vec\omega\times\vec r = \vec\omega\times\vec R$. Using the vector identity $\vec A \times (\vec B \times \vec C) = (\vec A \cdot \vec C)\vec B-(\vec A \cdot \vec B)\vec C$, we may simplify the centripetal acceleration term to obtain $$\vec a=\vec a_0 + \vec \alpha\times \vec R-\omega ^2\vec R.$$

Now to get the out-of-plane component of the torque, $$\require{cancel}\vec \tau =\int\rho(\vec r)\vec R\times \vec a \ dV$$ $$=\left(\int\rho\vec R\ dV\right)\times \vec a_0 + \int\rho\vec R\times (\vec \alpha \times \vec R) \ dV - \int\rho\omega^2\cancelto{0}{(\vec R \times \vec R)}\ dV$$ $$=\left(\int\rho\vec R\ dV\right)\times \vec a_0 + \left(\int\rho R^2\ dV\right)\vec\alpha$$ $$=M\vec R_\text{CM}\times\vec a_0 + I\vec\alpha$$ or $$\tau - M\vec R_\text{CM}\times\vec a_0 = I\vec\alpha.$$

We see that $\vec\tau = I\vec \alpha$ holds if only if $- M\vec R_\text{CM}\times\vec a_0 = 0$, where $\vec R_\text{CM}$ is the position of the center of mass relative to the reference point you pick. $- M\vec R_\text{CM}\times\vec a_0$ is the fictitious torque term that you need to account for to be able to use $\vec\tau = I\vec\alpha$ around an arbitrary point.

If you pick $A$, this point has no downward acceleration, so $-M\vec R_\text{CM}\times\vec a_0 = 0.$ If you pick $B$ (the center of mass), $\vec R_\text{CM} = 0$, so $-M\vec R_\text{CM}\times\vec a_0 = 0.$ Finally, for point $C$, the downward (tangential) component of $\vec a_0$ is $-2\alpha R$, so $-M\vec R_\text{CM}\times\vec a_0$ is $2MR^2\alpha$, out-of-plane (counter-clockwise). We can write in the counter-clockwise direction $$\tau_C + 2MR^2\alpha = I_C \alpha.$$ Plugging in $\tau_C = \frac13MRg$ and $I_C=\frac32MR^2$, we find the correct result $\alpha = -\frac{2g}{3R}$.

Answer 2

Newton's second law works in frames of reference which are not accelerating.
If an accelerating frame of reference is chosen then a fictional force acting at the centre of mass has to be added to enable Newton's second law to be used.

When doing such problems points $A$ and/or $B$ are usually chosen because:

• point $A$ is not acceleration and so no fictitious force is needed, and

• point $B$ has a downward acceleration of $2g/3$, and a fictitious force $2Mg/3$ acting at the centre of mass upwards is added but contributes zero torque about the centre of mass, $B$.

Point $C$ has a downward acceleration of $4g/3$ downwards, and a fictitious force $4Mg/3$ acting at the centre of mass upwards is added.

$\tau_{\rm C} = I_{\rm C} \,\alpha \Rightarrow \frac 13 Mg\cdot 2R+\frac 43 Mg\cdot R -Mg\cdot R= \frac 32 MR^2 \cdot \alpha\Rightarrow \alpha = \dfrac{2g}{3R}$

Note that this is no different to your diagram being rotated anticlockwise and representing a cylinder rolling down a slope without slipping.