Shouldn't the relation between torque and moment of inertia and angular acceleration be $\tau = I\alpha \sin\theta$?

Question

According to Fundamentals of Physics Extended 10th Edition by Halliday, Walker & Resnick, the relation between torque $(\tau)$ and moment of inertia $(I)$ and angular acceleration $(\alpha)$ is $\tau = I\alpha$, and I don't have a problem with this relation.

I think an alternate correct relation can be $\tau = I\alpha \sin\theta$. As $\tau=Fr$ was used in the deriving the formula, we get $\tau = I\alpha$. Instead, if $\tau = Fr\sin\theta$ was used in the derivation, we would've gotten $\tau = I\alpha \sin\theta$.

If we want to use $Fr\sin\theta$ for deriving the relation between torque, moment of inertia, and angular acceleration, we can see the derivation below:

$\tau=Fr_1\sin\theta$

$\implies \tau=m_1a_1r_1\sin\theta$

$\implies \tau=m_1\alpha r_1 r_1\sin\theta$

$\implies \tau=\alpha m_1\sin\theta(r_1)^2$

$\implies \tau_\text{net}=\alpha \sin\theta[m_1r^2_1+m_2r^2_2+m_3r^2_3+...]$

$\implies \tau_\text{net}=mr^2\alpha \sin\theta$

$\implies \tau_\text{net}= I\alpha \sin\theta$

To prove that my formula is correct, I will do a math with both my formula and $\tau=I\alpha$:

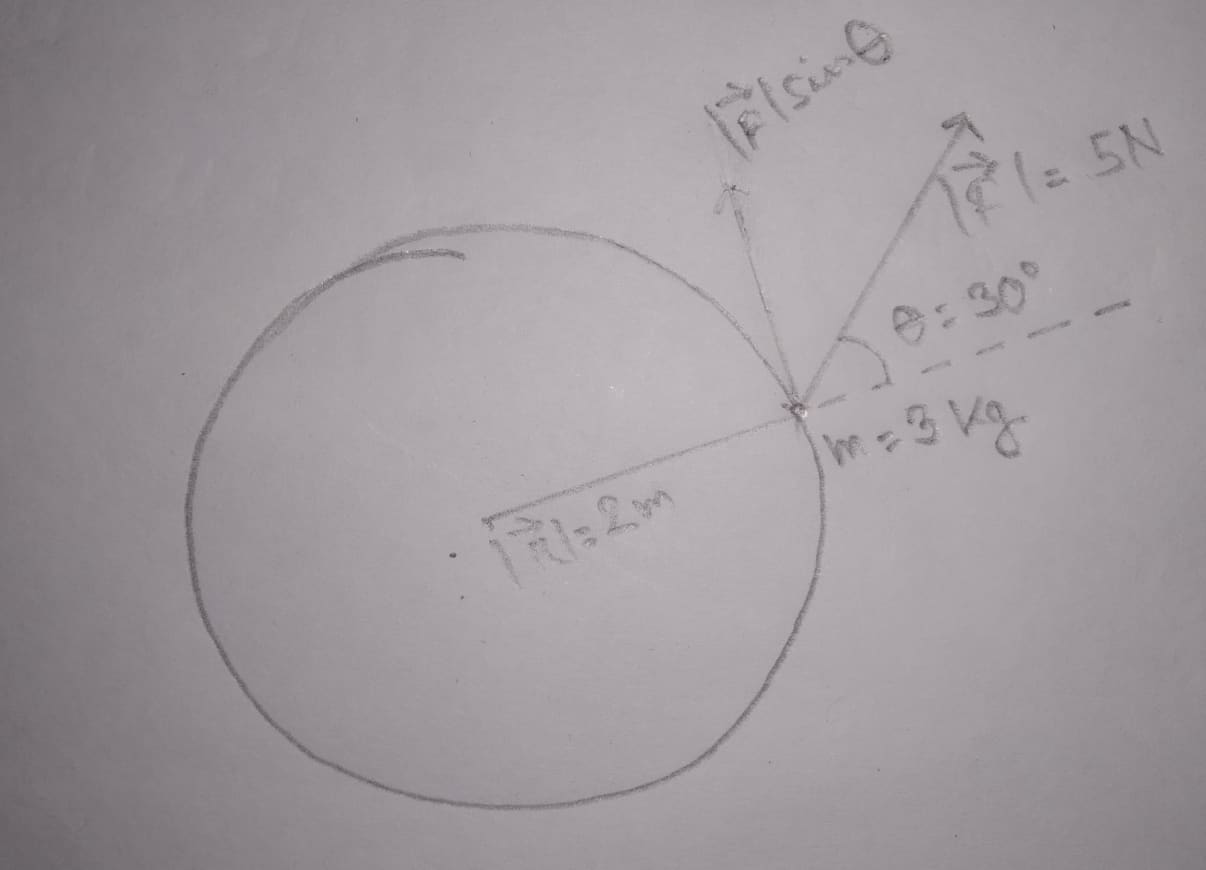
A point mass ($m = 3 \;\text{kg}$) is revolving in a circle of radius $2 \;\text{m}$. If a force $\vec F \ (|\vec F|=5 \;\text{N})$ that is not perpendicular to $\vec r$ acts on the point mass, what will be the applied torque, $\tau$?

Using $\tau=I\alpha$:

$\tau=I\alpha$

$\implies \tau=mr^2\times\frac{a}{r}$

$\implies \tau=3\times2^2\times\frac{\frac{5\times\sin(30)}{3}}{2}$

$\implies \tau=5 \;\text{N}\,\text{m}$

Using $\tau= I\alpha \sin\theta$:

$\tau= I\alpha \sin\theta$

$\implies \tau=mr^2\times\frac{a}{r}\times \sin\theta$

$\implies \tau=3\times2^2\times\frac{\frac{5}{3}}{2}\times\sin 30°$

$\implies \tau=5 \;\text{N}\,\text{m}$

So, we can see that my formula and $\tau=I\alpha$ are the same formulae. When $\theta=90°$, that is, when $\vec F$ & $\vec r$ are perpendicular, then my formula also becomes $\tau=I\alpha\times\sin 90°$$\implies \tau=I\alpha$. So, my derivation and formula are legitimate for the above reasons. How am I wrong?

Answer 1

The thing is that the relation $a_t=\alpha r$ gives the tangential component of the acceleration $a$, i.e. $a_t=a\sin{\theta}$. You can see this by differentiating $\vec{v}=\vec{\omega}\times \vec{r}$. You'd get $\vec{a}=\vec{\alpha}\times \vec{r} + \vec{\omega} \times \vec{v}$. The second term is directed along $\vec{r}$ and is called radial acceleration. The first term $\vec{\alpha}\times \vec{r}$ is perpendicular to $\vec{r}$ and is called tangential acceleration. So the tangential acceleration is only a part of the total acceleration $\vec{a}$

Even $\vec{\omega} \times \vec{r}$ only gives you the tangential velocity. Since this cross product is perpendicular to $\vec{r}$, it can't have any radial component. But the thing is, the radial component is 0. As all the particles are going in circles, the tangential velocity is equal to the total velocity $\vec{v}$. Things change when we talk about total acceleration $\vec{a}$ because, for any particle to go in a circle, it must experience a centripetal acceleration which is directed along the radius.

$$\tau=Fr\sin{\theta}$$ $$=mra\sin{\theta}$$ $$=mra_t$$ $$=mr^2\alpha$$ $$=I\alpha$$

Answer 2

The relationship $\tau = I \alpha$ is the correct one. It relates torque about an axis to the angular acceleration about the same axis, and the mass moment of inertia also about this axis.

It is true that if $\tau$ is a result of an offset force $F$ at a radius distance $r$, with angle $\theta$ between the force direction and the radial direction, then

$$ \tau = (r \sin \theta) F $$

But that just described the left hand side of $\tau = I \alpha$ to produce

$$ (r \sin \theta) F = I \alpha $$

Here $r \sin \theta$ is the moment arm of $F$ about the axis.

In the question you blindly substitute $F = m a$ without consideration of all possible forces acting on the body (such as pin reaction forces) and no consideration of which direction is the force $F$ acting and what direction is the acceleration $a$ possible.

This is where your equation progression fell apart.

To derive $\tau = I \alpha$ you sum up all the individual angular momentum of each particle in the body to show that $H = I \omega$. Then use Newton's second law relating force to derivative of translational momentum, for each particle of the body to find that $$\tau = \frac{{\rm d}}{{\rm d}t} H = \frac{{\rm d}}{{\rm d}t} (I \omega) = I \alpha$$

Better yet read-up on the derivation of the Newton-Euler equations of motion in vector from

$$ \begin{aligned} \sum \boldsymbol{F} & = m \boldsymbol{a} \\ \sum \boldsymbol{\tau} & = \mathrm{I} \boldsymbol{\alpha} + \boldsymbol{\omega} \times \mathrm{I} \boldsymbol{\omega} \end{aligned}$$

Answer 3

The $\sin(\theta)$ is included in the definition of the torque, which uses only the component of the force which is doing work in the tangential direction.