0
$\begingroup$

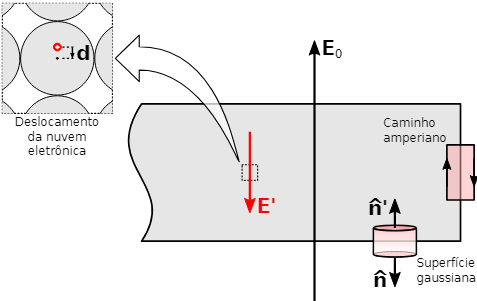
Studying electrostatics, using Griffths, I got the following issue whose explanation I couldn't find anywere. Consider the image below to follow the problem.

If a have a chunk of a linear dielectric under the influence of an external uniform electric field $E_0$. The polarization of the material produces an opposite electric field $E'$, so that the total electric field inside the material is $E$.

From Gauss' law, using a tiny Gaussian cylindrical surface, we can show that the electric field is discontinuous, ie., $\epsilon_0 E_0 - \epsilon E = 0$, where $\epsilon_0$ and $\epsilon$ are the outside and inside electric permitivity, respectively. However, from Faraday's law, using a tiny rectangular amperian loop, we can show that the field is continuous, ie., $E - E_0 = 0$.

How can it be possible that the electric field is continuous and discontinuous at the same time, depending on the choice it is analyzed, either by Gauss or Faraday equation?

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Faraday's Law: $\nabla \times \mathbf{E} = - \partial \mathbf{B}/\partial t$. The Amperian loop is just the name of the rectangular path $\endgroup$
    – Brasil
    Commented Sep 2 at 22:23

2 Answers 2

Reset to default
1
$\begingroup$

To illustrate the effcet of the "bulging" electric field here is an illustration from Maxwell's A Treatise on Electricity and Magnetism which shows the electric field lines and the equipotential lines at the end of a pair of parallel plates.

enter image description here

Using the integral form of Faraday's law one has to evaluate $\oint \vec E\cdot d\vec \ell$ around path $abcda$.

$a\to b$ along equipotential with line integral zero,
$b\to c$ along an electric field line with line integral $\Delta V$,
$c\to d$ along an equipotential with line integral zero, and
$d\to a$ along an electric field line with line integral $-\Delta V$.

Thus $\oint \vec E\cdot d\vec \ell =0$.

The magnetic equivalent of "bulging" fields is explained in the answer to Why is Ampère's law violated if there are no fringe fields?

Improve this answer
$\endgroup$
3
  • $\begingroup$ So, you are trying to tell that all books of physics are wrong? Since they state that the boundary conditions for the tangential component of the electric field at the interface of a dielectric is continuous? $\endgroup$
    – Brasil
    Commented Sep 15 at 14:19
  • $\begingroup$ The books make approximations which neglect the “negligible” fringe fields to make analysis easier. $\endgroup$
    – Farcher
    Commented Sep 15 at 15:10
  • $\begingroup$ The books take this so seriously that this is the only way I find to derive Fresnel relations. Is there another wey? Because if there isn't, then my question remains unsolved... $\endgroup$
    – Brasil
    Commented Sep 15 at 16:02
0
$\begingroup$

You are using a wrong model for this. The real field will be closer to a parallel plate capacitor, and it is not the type where you have a rectangular sudden increase of E field at the edges. The Gaußian surface is still correct, but in your Ampèrian loop, the field is decaying smoothly, so that loop integral is zero. That is also why textbooks say that the field at the sides of a capacitor is half that of the field at the centre. The field spills out, in a sense.

Improve this answer
$\endgroup$
5
  • $\begingroup$ What is wrog with assuming that the slab is under uniform $E_0$ field and is uniformly polarized? If it is wrong, how can I obtain correctly the boundary conditions to derive the Fresnel equations of reflection/transmission of electromagnetic waves between transparent media? $\endgroup$
    – Brasil
    Commented Sep 3 at 3:30
  • $\begingroup$ You yourself know that Maxwell's equations will not make fields that change abruptly at a boundary without special stuff happening, like those surface charges. There is nothing that can make the fields be $E$ on one side of the boundary and $E_0$ on the other side, in the Ampèrian loop, and so that is extremely unphysical. At least in the Gaußian surface you have surface charges causing this change to be physical. What you do is to take a tremendously large slab, so that you can ignore the Ampèrian loop boundary and instead only focus upon the Gaußian surface. $\endgroup$
    – naturallyInconsistent
    Commented Sep 3 at 3:47
  • $\begingroup$ Is this not the Faraday's law equivalent of Why is Ampère's law violated if there are no fringe fields? $\endgroup$
    – Farcher
    Commented Sep 3 at 8:42
  • $\begingroup$ @Farcher indeed! That's a nice answer on your part; take my upvote $\endgroup$
    – naturallyInconsistent
    Commented Sep 3 at 8:50
  • $\begingroup$ Again, if my model is wrong, how these boundary conditions can be possibly applied to derive the Fresnel Equations of reflection and transmission of electromagnetic waves? $\endgroup$
    – Brasil
    Commented Sep 3 at 18:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.