Why cant we save the heat rejected in a heat engine?

Question

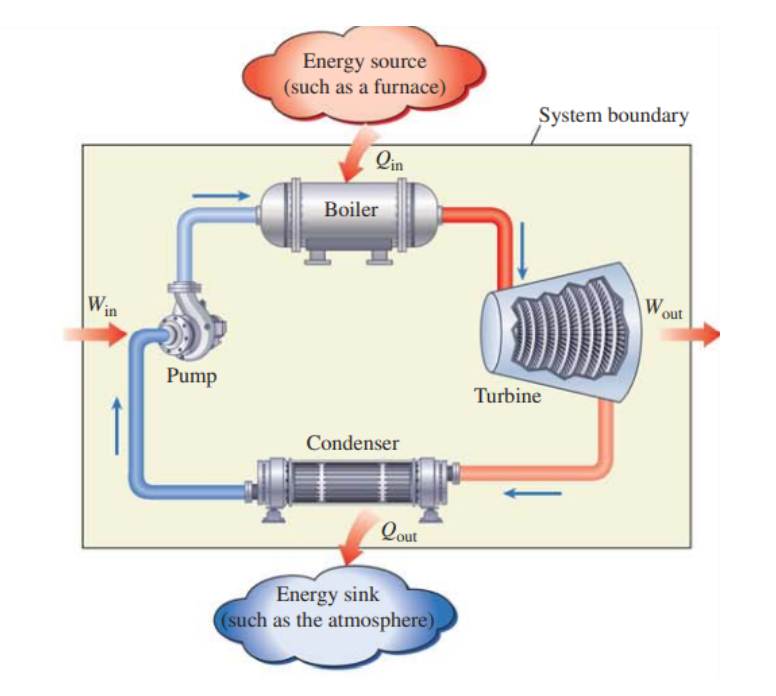
Suppose the working fluid is heated in a boiler by combustion of fuel outside the heat engine. If we assume that this a steady flow process with no change in time, neglect the kinetic and potential energies and heat losses from the boiler. The energy balance reduces to

$m^{.}h_{1}+Q^{.}_{in}=m^{.}h_{2} $

The working fluid expands then expands in a turbine thus generating work. If we assume that this a steady flow process, the system is adiabatic (heat transfer from turbines is negligible), and neglect kinetic and potential energy changes. The energy balance reduces to

$ m^{.}h_{2}=W^{.}_{out}+m^{.}h_{3} $

The working fluid leaving the turbine enters the condenser rejecting the remaining waste heat to a low-temperature sink. If we assume that this a steady flow process with no change in time, neglect the kinetic and potential energies and heat losses from the boiler. The energy balance reduces to

$ m^{.}h_{3}+Q^{.}_{out}=m^{.}h_{4} $

The working fluid is compressed or pumped to the boiler. The pump or compressor require power input from external source. If assume that this a steady flow process, adiabatic, and neglect the the kinetic and potential energies. The energy balance reduces to

$ m^{.}h_{4}+W^{.}_{in}=m^{.}h_{1} $

$ W^{.}_{net}=W^{.}_{out}-W^{.}_{in}=(m^{.}h_{2}-m^{.}h_{1})+(m^{.}h_{4}-m^{.}h_{3}) $

$ Q^{.}_{in}=m^{.}h_{2}-m^{.}h_{1} $

The performance of the heat engine is measured by $ \eta=\frac{(m^{.}h_{2}-m^{.}h_{1})+(m^{.}h_{4}-m^{.}h_{3})}{m^{.}h_{2}-m^{.}h_{1}} $

If we discard the condenser to save the waste heat and we send the working fluid directly to the pump or condenser. The energy balance for the compressor changes to

$ m^{.}h_{3}+W^{.}_{in}=m^{.}h_{1} $

$ W^{.}_{net}=W^{.}_{out}-W^{.}_{in}=(m^{.}h_{2}-m^{.}h_{1})+(m^{.}h_{3}-m^{.}h_{3})=(m^{.}h_{2}-m^{.}h_{1}) $

The efficiency equals 1 in this case. Why is the above scenario not feasible? The enthalpy of the working fluid that expands in a turbine to produce work decreases but which of the two terms of the combination (u+Pv) decreases (internal energy or flow energy) or do they both decrease?. I am asking this question to determine whether the temperature of the fluid decreases or stays the same. If the condenser is included, and we assume negligible pressure drop, enthalpy would decrease as the working fluid exchanges heat accompanied by a decrease in temperature. During compression, enthalpy increases but which of the two terms of the combination (u+Pv) increases (internal energy or flow energy) or do they both increase?

Should we reject heat because if we discard the condenser and directly compress the working fluid at a higher temperature we would need to intentionally cool it to bring it to the temperature of state 1 (the fluid entering the boiler)? Without cooling we can never attain state 1 we would obtain a state that's at a higher temperature

Answer 1

You are essentially misunderstanding the function of the heat sink. It is not to reject the heat but rather to cool down the area of the turbine output so that it has lower pressure than the turbine input (from the heat source).

If we don't do that then the pressure at the turbine output will continue to increase until it reaches equilibrium with the turbine input and thus the pressure difference across the turbine becomes zero and the turbine stops spinning.

The heat sink is there to make the turbine spin.

Answer 2

The efficiency equals 1 in this case. Why is the above scenario not feasible?

It would violate the Kelvin-Planck statement of the second law of thermodynamics, which essentially says no heat engine can produce net work operating in a cycle while exchanging heat with a single heat reservoir.

If I understand your proposal correctly, you would take the output of the turbine (saturated vapor), bypass the condenser (heat rejection) and send it directly to the pump to compress it into saturated vapor at the boiler pressure and temperature (which is the same as the input pressure and temperature to the turbine). In effect, the pump would perform the reverse work of the turbine for a net cycle work of zero.

Hope this helps.

Answer 3

Rather than a physics answer, let's look at the practical answer. The steam entering the turbine is at a high pressure and a high temperature. As that steam goes through the turbine, it loses pressure and temperature while generating shaft work. Upon exiting the turbine, that working fluid is still steam, albeit at a low temperature and pressure. It would take a LOT of input shaft work (aka PV work) to use a compressor to increase the temperature and pressure of the steam back to the conditions needed to run the steam turbine, and probably more shaft work than was extracted at the turbine because every process operates at less than 100% efficiency.

Accordingly, the low pressure steam is condensed into a much lower volume of water and sent through a pump. It takes MUCH less work to increase the water pressure high enough to get it into the boiler because water is more or less incompressible, meaning that while the pump substantially increases the water pressure there is little or no change in the water's volume (aka very low PV work). The boiler then heats the water enough to turn it back into high pressure and high temperature steam before being sent to the turbine.

Answer 4

All known conversion of thermal energy into work requires a certain amount of entropy transported across a temperature differential. Entropy is not destructible. In your problem, the conversion of thermal energy to work requires that some amount of entropy, say, $\Delta S_b$ absorbed by the boiler from the furnace per cycle or unit time, be moved from a higher temperature that is the boiler temperature, $T_b$, to a lower temperature, here that of the condenser, $T_c$.

The amount of thermal energy absorbed is $T_b \Delta S_b,$ and if the process is reversible then in the adiabatic expansion in the turbine the absorbed entropy is conserved and in the condenser the same amount of entropy is rejected representing $T_c\Delta S_b$ thermal energy. The difference of the two energies, absorbed and rejected, is available to be converted to work $\Delta E= (T_b-T_c)\Delta S_b$.

In a reversible process all of $\Delta E$ is available for work, in an irreversible process in which entropy, $\sigma_i \ge 0,$ is internally generated at various temperatures, $T_i,$ only a portion of $\Delta E$ is available for work $\Delta W$, the rest is dissipated as heat, specifically $$\Delta W = \Delta E - \sum_i T_i\sigma_i \le \Delta E.$$

If you measure your efficiency as the ratio of the maximum available work $\Delta E$ relative to the input thermal energy, $T_b\Delta S_b$, you get $$\eta = \frac{\Delta W}{T_b\Delta S_b}, $$ and then for a reversible process you get the so-called Carnot efficiency, $\eta = 1-\frac{T_c}{T_b} \le 1$.

If instead you measure the efficiency relative to what could be achieved if the process were reversible but in fact it is irreversible then you get a different measure of efficiency $$\eta^* =\frac{\Delta W}{\Delta E}=1-\frac{\sum_i T_i\sigma_i }{(T_b-T_c)\Delta S_b}\le 1.$$