21
$\begingroup$

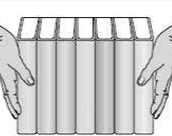
The books held in hand

An even number of books (say four) are held in the manner shown in the figure. It is obvious that if we apply a large force inwards, we can increase the normal force, and hence the friction which keeps the books in equilibrium. Assume all the books are identical.

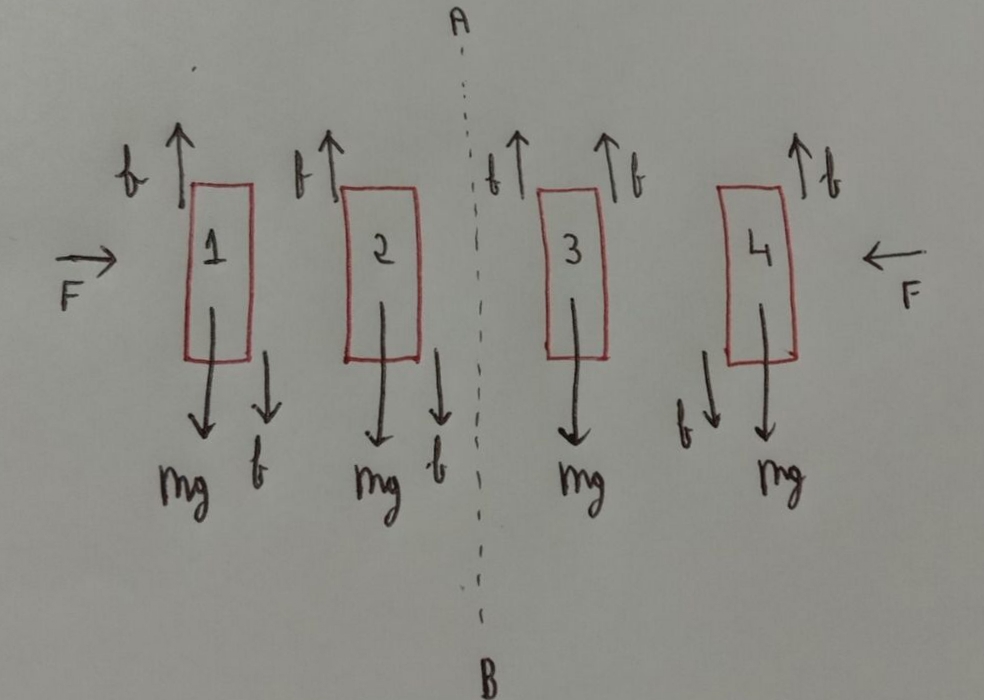
On considering the equilibrium of the books individually, two problems arise when showing the direction of friction acting on the books two and three. (consider figure)

The figure

F denotes force applied and f denotes the friction force between the books which may or may not be same between all the books.

The problems:

  1. The situation is symmetrical about the line AB hence, the free body diagram on the left side of AB must be same as that on the right side of AB.

Thus the friction between the book 2 and 3, I believe, must act in the same direction. However such a thing would violate Newton's third law that friction must act in opposite directions on the two surfaces in contact.

  1. Neglecting the fact that the situation is symmetrical (as I have done in the figure), equilibrium is not possible for both the books 2 and 3 simultaneously as the net upward force balancing weight for both is different.

My questions:

  1. Am I doing something horribly wrong?
  2. Does it mean that no friction acts between books 2 and 3?

NOTE: Such a problem arises only when even number of books are involved.

Improve this question
$\endgroup$
3
  • 2
    $\begingroup$ I can't see why you think "the fact that friction acts in opposite directions" The friction works upwards in all books, $\endgroup$
    – trula
    Commented Jul 14 at 17:07
  • 4
    $\begingroup$ By Newton's third law if friction acts upwards on one surface it must act downwards on the other surface in contact $\endgroup$
    – Nightwing
    Commented Jul 14 at 17:08
  • $\begingroup$ "Neglecting the fact that the situation is symmetrical ... the net upward force balancing weight for both is different" This cannot be, since it is symmetrical so when ignoring that it is the situation must be consistent with it. How do you justify this? The reasoning in the sentence is not clear. (If by "neglecting" you are trying to say "assuming it's not symmetrical", that is poor writing & describes a different case than this one so it doesn't tell you about this case.) $\endgroup$
    – philipxy
    Commented Jul 15 at 2:31

2 Answers 2

Reset to default
18
$\begingroup$

You are correct, in a scenario with an even number of books there will be no friction acting between the pair in the middle. To see this, take the simplest case - two books. The force $F$ applied by the hand gives rise to a frictional force $f_1$ between the hands and the books they are touching. This friction force $f_1$ is equal to the weight of each book, given that $F$ is large enough for the surfaces. Since the books do not have a tendency to move with respect to each other (if $F$ were taken out, they'd fall together!), there is no friction between them.

For more than 2 books (say 4, as in your question), everything outside the middle two books acts just like the hands in the 2-book case. However, for the outer books, there is a different for $f_2$ acting between them and the hands (i.e hand to outer book is $f_2$ and outer book to middle is $f_1$ with $f_1 = mg$ and $f_2 = 2mg$). You can verify that the directions of the forces match to make the net force on each book zero.

P.S The symmetry argument you present is a pretty slick way to show that no friction acts between the middle two books. Avoids all the arrows and head-scratching :)

Improve this answer
$\endgroup$
10
  • $\begingroup$ If there is no friction between 2 & 3, do they not have a torque induced on them due to the friction from 1 and 4? What cancels out the torque, then? $\endgroup$
    – HiddenBabel
    Commented Jul 15 at 3:17
  • 4
    $\begingroup$ @HiddenBabel, the normal forces don't have to point through the center of mass. Alternatively, you could imagine that the normal forces are split into an "upper" part and a "lower" part, and those parts may not be equal. Either would create a torque. $\endgroup$
    – BowlOfRed
    Commented Jul 15 at 4:49
  • $\begingroup$ The available friction force given by $F=\mu N$ will normally be greater than the actual friction forces $f_1$ etc. If they were equal then any upward acceleration of the hands (such as while walking normally) would result in books slipping. The friction force in the centre is theoretically zero, but in practice it will be small but non-zero due to different movement of the two hands, etc. The available friction force is much larger, enough to prevent movement from these small effects. Incidentally, as $f_2>f_1$, the books with slippery covers should be towards the inside of the stack. $\endgroup$
    – Peter
    Commented Jul 15 at 12:38
  • 2
    $\begingroup$ @NuclearHoagie I don't have a problem imaging holding a book up against an effectively frictionless wall. If I push a book into an ice-covered wall it will stay in place while I push it. Or replace the book with a model car, I can hold it against the wall with friction between the car's roof and my palm. $\endgroup$
    – bdsl
    Commented Jul 15 at 14:04
  • 1
    $\begingroup$ @bdsl - No it won't. And even if it did, that wouldn't stop the sliding (you need to apply a counteracting force for that). $\endgroup$
    – Filip Milovanović
    Commented Jul 17 at 4:28
3
$\begingroup$

Not all friction forces labeled $f$ in the sketch are identical. I fact, they become smaller as you move towards the centre on the stack. Realizing this removes all of the mentioned issues.

  • The frictions between the hands and the outer books are large. Essentially, these carry the total weight of all four books.

  • The friction between books 1 and 2 as well as between 3 and 4 are smaller. These carry only the weight of books 2 and 3.

  • There is no friction between books 2 and 3. There is nothing for such a friction force to carry.

The friction forces do appear in opposite force pairs everywhere as per Newton's 3rd law, but you should sketch them progressively smaller towards the centre of the stack. Now there is no symmetry issue, no violation of Newton's 3rd law anywhere, and a clear explanation of the purpose of each friction force.

  • E.g., the friction from book 1 on book 2 points upwards and equals the weight of book 2.
  • In turn, book 2 pulls down in book 1 with this same friction force, which is the weight of book 2. The friction force from the hand on book 1 must carry the weight of book 1 as well as counteract this friction from book 2 (which all in all means that it carries the weight of both books).
  • The farther from the centre you are in the stack, the more the friction forces have to carry.

All in all, be careful not to be "too quick" when subdividing a system into split systems. My advise would be to treat each split system individually and to avoid drawing a combined sketch. In your treatment of each split system you will apply boundary conditions and "effects", which here are constituted by the friction forces. Be very careful with any assumptions on boundary conditions - in fact, try to forget any external information until you have drawn out the full freebody diagram for a split system so as to avoid applying assumptions that only applied to the full system.

Improve this answer
$\endgroup$
3
  • $\begingroup$ +1. A followup question that ties into your theme of being wary of overgeneralizing too easily would be: why doesn't the frictional force decay continuously, but only at the interfaces between books? In this case, friction from the glue holding each book together, plus the fact that the book covers are heavier than the pages, would explain that. But if you had a stack of unbound pages of the same size, you would get a smoother decay. This is just a tangent, not really what the OP was asking, but I just thought it was an interesting generalization that perhaps highlights your main point. $\endgroup$
    – Andrew
    Commented Jul 15 at 22:36
  • $\begingroup$ @Andrew Thank you for that comment. Yes, it is a good point that you essentially can split your system into atoms and then see a perfectly smooth gradual transition from one friction force to the next of a slightly lower magnitude. $\endgroup$
    – Steeven
    Commented Jul 16 at 5:47
  • $\begingroup$ @Steeven I really like your clean modular solution. However, it reawakens in me a suspicion I could never get fully rid off, that is in solving certain tricky physics problems one should know the solution to set the stage in a convenient way, while starting with a pure algorithmic application of the laws is much harder. For example is there any specific criteria to select the verses for the action & reaction inter-book friction forces as you did? A priori it seems the relative motion of books with same mass is undetermined. (In practice this might be irrelevant, as sign of results shows verse) $\endgroup$
    – MarcoD
    Commented Jul 17 at 0:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.