0
$\begingroup$

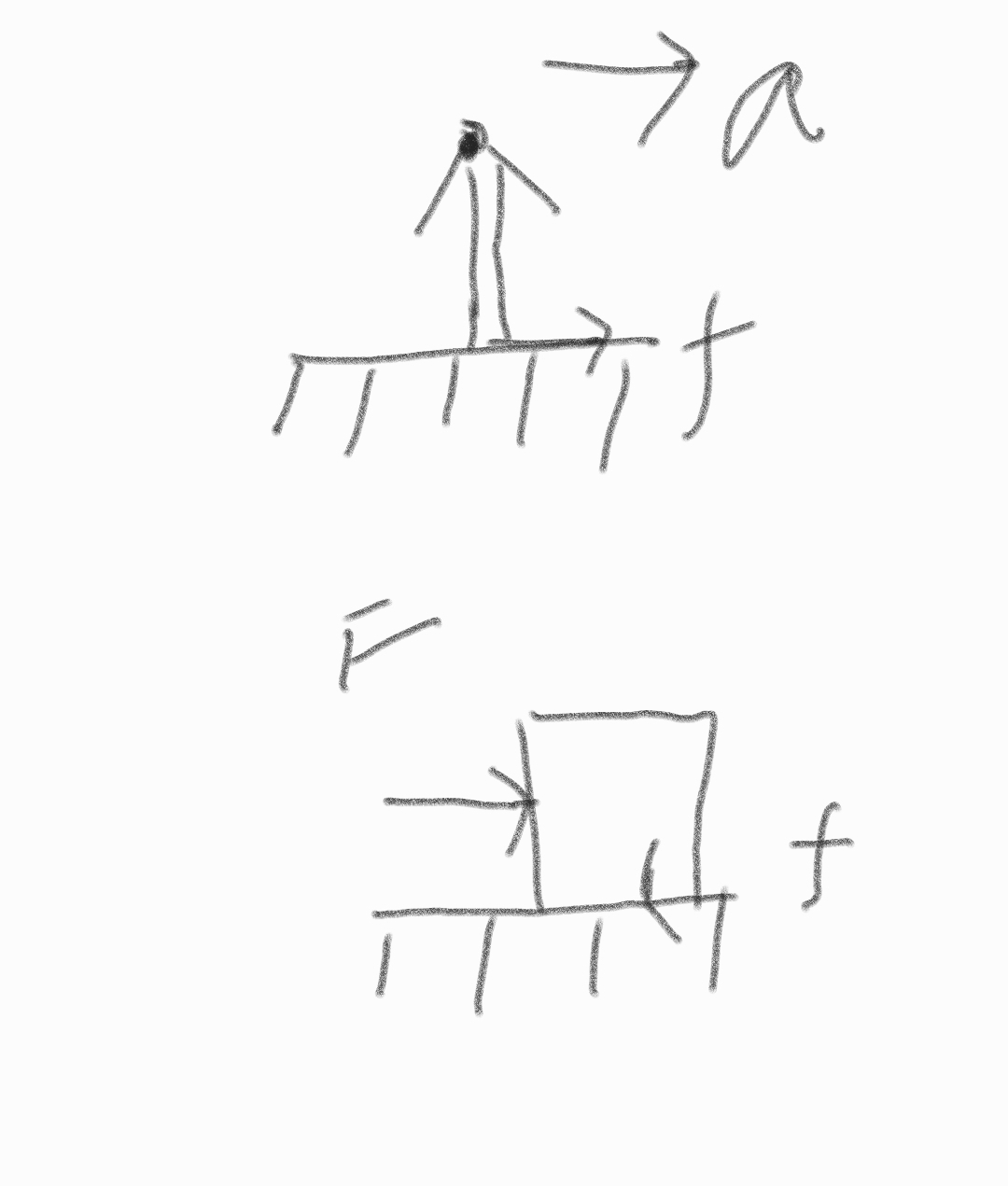
enter image description here

In figure-1,a man is walking on ground with an acceleration $a$ forward. Now since the only force in $+x$ direction is the the frictional force,we say that the frictional force is taking the man forward. So friction is in the direction of motion.

In figure-2 A man applies a force $F$ on the box,but again friction is applied against the direction of force applied or motion. How is it that in the first case,when there is no external force,friction is in the direction of motion but in the 2nd case,when there is external force,friction is agaisnt the direction of motion?

From the definition of friction,we know it resists the relative motion of two objects,meaning it can either hinder one object from moving towards other or try to keep both objects moving. So in the first case, when the man was walking, the ground is always at rest,so relative to the ground,the man is walking away from the surface, but since friction can't make the ground move,shouldn't it act against the direction of motion? I find it very contradictory.

Improve this question
$\endgroup$

3 Answers 3

Reset to default
0
$\begingroup$

How is it that in the first case,when there is no external force,friction is in the direction of motion but in the 2nd case,when there is external force, friction is agaisnt the direction of motion?

In the first case the static friction force that the ground exerts forward on the man is an external (to the man) force. It is the force that is equal and opposite to the force the man's foot exerts back on the ground, per Newton's 3rd law. Since it is the only external force acting on the man (ignoring air resistance), the man accelerates.

In the second case the force exerted by the man on the box is an external force on the box. The other external force on the box is the static friction force the ground exerts back on the box. As long as the maximum possible static friction force between the box and ground is not exceeded, the two external forces acting on the box are equal an opposite, for a net force of zero and no acceleration of the box.

but since friction can't make the ground move, shouldn't it act against the direction of motion? I find it very contradictory.

But the ground does move. It's just that its motion is so infinitesimal as to be immeasurable. That's because its mass is so much greater than that of the man. Its acceleration is $a=F/M$ where $F$ is the force the man exerts on the earth and $M$ is the mass of the earth. Whereas the acceleration of the man is $a=F/m$ where $m$ is the mass of the man and $F$ is the force the earth exerts on the man, per Newton's 3rd law.

Hope this helps.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Thank you very much for replying. In the first paragraph for figure-1,as you said that the horizontal force that the ground exerts on the man is the frictional force,i have a few silly questions on that. We know that friction prevents an attempt of relative motion. So suppose a really absurd situation. Person $A$ is ground and another person $B$ is an object. $B$ is standing on the body of the person $A$. When $B$ applies a force on $A$,it is trying to go away from $A$,meaning there is a possibility of relative motion. So A will surely prevent $B$ from going forward to stop relative motion. $\endgroup$
    – madness
    Commented Aug 15, 2022 at 18:20
  • $\begingroup$ So $A$ will pull $B$ towards him(aka) in the backward direction. So frictional force should act backward. But why is it that frictional force is in the forward direction as you said? $\endgroup$
    – madness
    Commented Aug 15, 2022 at 18:21
  • $\begingroup$ I'm having a hard time following you. For one thing I'm not sure what you mean by "A is ground". But there is a static friction force between A and B. As long as the maximum possible static friction force between them is not exceeded, there will be no relative motion. The static friction force that A exerts on B will cause B to move forward. $\endgroup$
    – Bob D
    Commented Aug 15, 2022 at 18:26
  • $\begingroup$ Now, if by "A is ground" you mean A replaces the earth in your first scenario then we can consider A and B to be in outer space with no external forces acting on the combination of the two. Then if B standing on A exerts a backward force F on A then by Newton's 3rd law A will exert an equal and opposite forward static friction force of F of on B. $\endgroup$
    – Bob D
    Commented Aug 15, 2022 at 19:30
  • $\begingroup$ If the maximum possible static friction force between A and B is not exceeded, there will be no relative motion between A and B (meaning B will not slide on A). Relative to an inertial observer in space, B will have a forward acceleration of $a_{B}=F/M_{B}$ and A will have a backwards acceleration of $a_{A}=F/M_{A}$. $\endgroup$
    – Bob D
    Commented Aug 15, 2022 at 19:30
0
$\begingroup$

Yes, the friction is the force resisting the relative motion on a solid surface. The contradictory in figure-1 is that the man moves forward but the friction force points in the same direction.

If we, instead of using a man, use a box in figure-1, we can conclude that the box would not move forward by itself (i.e. frictional force would point in the backward direction). The fact is that the frictional force, when a man walks, is produced when the man's foot trying to move backward. Thus it points in the forward direction. This can be more clearly seen when looking at the rotation of a wheel of a vehicle moving on a road.

Improve this answer
$\endgroup$
-1
$\begingroup$

With the man, his force is in the direction of motion, friction against the direction.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.