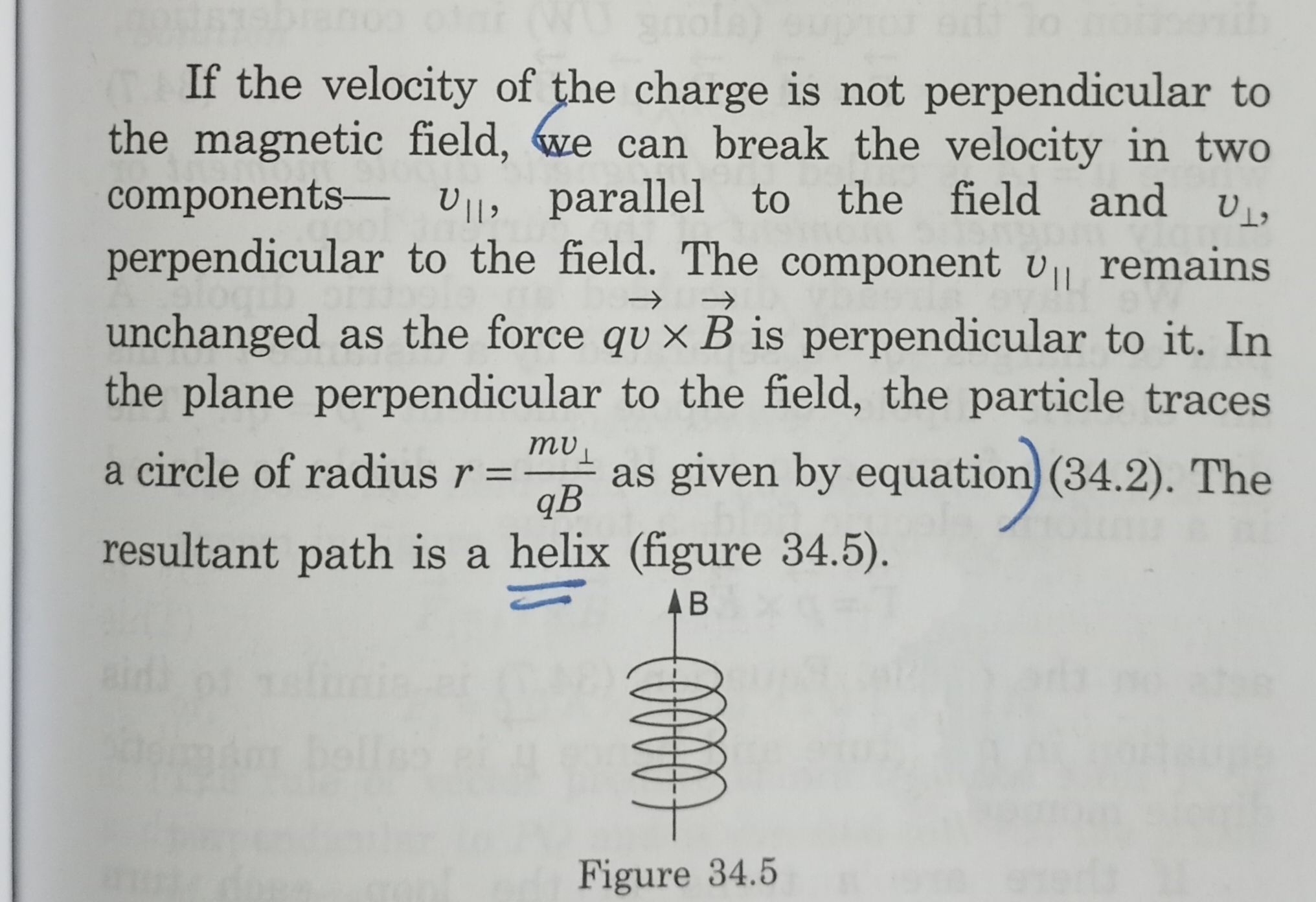
So according to this para, I understand that since one component of velocity is perpendicular to magnetic force (and parallel to magnetic field) that component will not change. But other component is parallel to the force right? Then that means that component should with some acceleration right? Then how can that particle trace a circular path?
If you write the force on the particle due to the magnetic field,
\begin{equation} \vec{F}=q(\vec{v}_{\perp}+\vec{v}_{\parallel})\times\vec{B} \end{equation}
The term $\vec{v}_{\parallel}\times\vec{B}$ vanishes because the two vectors are parallel. So the force is given by,
\begin{equation} \vec{F}=q\vec{v}_{\perp}\times\vec{B} \end{equation} This equation tells you that the force is perpendicular to both $\vec{v}_{\perp}$ and $\vec{B}$ (right hand rule for cross product). It means that the force is in a plane containing $\vec{v}_{\perp}$ and perpendicular to the magnetic field. In this plane perpendicular to $\vec{B}$, the force is always perpendicular to $\vec{v}_{\perp}$, it represents a case of central force for which the centripetal force is the magnetic force. So,
\begin{equation} qv_{\perp}B=\frac{mv_{\perp}^2}{R} \end{equation}
Because the parallel component of the velocity exists but remains unchanged, the particle trajectory is an helix.
